Elements Of Modern Algebra
Elements Of Modern Algebra
8th Edition
ISBN: 9781285463230
Author: Gilbert, Linda, Jimmie
Publisher: Cengage Learning,
Bartleby Related Questions Icon

Related questions

Q: Suppose R is a relation defined on the set A = {1,2, 3, 4}. If R is an equivalence relation, what is…
A: Given below the detailed solution
Q: 9.32. A relation Ris defined on the set A = {a+bv2: a,b € Q, a + bv/2 + 0} by x R y if æ/y E Q. Show…
A:
Q: (3) Let S be the equivalence relation on (0, 1, 2, 3} x {0, 1, 2} defined by (a, b)S(c, d) if and…
A: S=0,1,2,3×0,1,2=0,0;0,1;0,21.0;1,1;1,22.0;2,1;2,23.0;3,1;3,2
Q: 4. Let f : X → Y define a relation on the set X by „Ry if and only if (x) = fy). Is f an equivalence…
A: f is an equivalence relation if f satisfies three properties. 1. if f is a reflexive relation  2. if…
Q: Let R be the relation on the set of integers, s.t., aRb if and only if a=b or a= -b. Is R an…
A:
Q: Let = be an equivalence relation on a set A. For every element a E A, let [a] denote the equivalence…
A:
Q: Given a set define a relation induced by a partition of set A and that partition must consists of at…
A:
Q: Given a non empty set X, consider P(X) which is the set of all subsets of X.      Define the…
A: The relation is defined as follows.
Q: In each item below, verify that ~ is an equivalence relation on the given set. Let S = Z × N. Define…
A: Since you have asked multiple questions, we will solve the Question Q (a) and (b) for you. If…
Q: Determine whether the following relation is an equivalence relation? R= {(a, a), (a, c), (c, a), (b,…
A:
Q: B if there exists o E Sn so that oao- = B. Prove that For a, B E Sn, let a ~ equivalence relation…
A:
Q: Let S be the equivalence relation on {− 1, 0, 1} × {0, 1, 2, 3} defined by (a, b )S(c, d) if and…
A: Let A  = {-1,0,1}×{0,1,2,3}  i.e A=…
Q: Let A be a set and R be a symmetric and transitive relation on A. Prove the following statement: If…
A:
Q: 9.24. Let R be an equivalence relation on A = {a, b, c, d, e, f,g} such that a Rc, c Rd, d Rg and b…
A:
Q: (b) Let R be the equivalence relation on the set S = {1,3, 5, 7, ..., 49} defined by x Ry if and…
A:
Q: Give a specific reason why the following set R does not define an equivalence relation on the set…
A: We will find out the required value.
Q: efine the relation ~ on the set N of positive integers by a~b if and only if  a = b(10^k) for some…
A:
Q: for an equivalence relation R on A, both sets, the class of equivalence classes of R —A/R— is a set.
A:
Q: Consider the equivalence relation ~ on R given by a ~ b if and only if [a] = [b]. You do not need to…
A: Given that the equivalence relation ~ on ℝ defined as a~b if and only if a=b. Define a function…
Q: 2. Define the relation on S = Z by a~b + a = b mod 5. (1) Prove that is an equivalence relation. (2)…
A:
Q: (b) Observe that all the relations in (a) are equivalence relations. Prove that this is true in…
A: Given: A is a nonempty set, and P is a partition of A. To show: The relation R is equivalence…
Q: Subject: Set Theory
A:
Q: In each item below, verify that ~ is an equivalence relation on the given set. (a) Let S = Z x N.…
A:
Q: 3. Given a relation R on a set A, prove that if R is transitive, then so is R-1.
A: We are given  that R is a transitive relation on a set A  Transitive Relation  A relation on a set X…
Q: Show that each of the following relations equivalence classes. on Z is an equivalence relation, and…
A: In the given question we have to find the equivalence classes.
Q: be an equivalence relation on the set A = {1, 2,...,8}, and denote the equivalence class of x E A by…
A:
Q: If R1 and R2 are equivalence relations in a set A, show that R1 ∩ R2 is also an equivalence…
A: Given, R1 and R2 are equivalence relations in a set A. So, both R1 and R2 are Reflexive, Symmetric…
Q: Show that the relation R defined on the set of integers ℤ by (a,b) Î R if a – b = 6k for some k Î ℤ…
A:
Q: Let A be a set and let ∼ be an equivalence relation on A. Let x, y ∈ A. Prove that [x] = [y] if and…
A:
Q: Let A = {a, b, c, d, e} and define an equivalence relation R C A × A on the set A as follows: R =…
A: We will answer the first question as you didn't specify any. Please resubmit the other question…
Q: Consider the set Q = Z × (Z 10), and the relation fupon Q Defined as: (a, b) ↑ (c, d) + a•d = b•c a)…
A: we shall solve the part (a) only , if you want us to do other parts please resubmit the question…
Q: Let S be a set of distinct symbols. Show that the relation defined onW(S) in this chapter is an…
A:
Q: Determine whether or not the following relations are equivalence relations on the given set. If the…
A: Let m, n, p∈ℚ Relation is m~n in ℚ if mn≥0 (i) Reflexive: Let m∈ℚ then mm=m2≥0 Therefore m~m   ∀m∈ℚ…
Q: (a, b, c, d) and define a relation R on A as follows: R = ((a, a), (b, b), (a, b), (c, c), (b, a),…
A:
Q: Define a relation Q on the set R × R as follows. For all ordered pairs (w, x) and (y, z) in R x R,…
A: The relation Q on the set R×R is defined as follows. For all ordered pairs w, x and y, z in R×R, w,…
Q: 1. Show that R is an equivalence relation on P(A). 2. Set A = {1, 2, 3, 4} and B = {1, 24}.…
A:
Q: Consider the subset relation C on the power set of {a,b,c}, that is P{a,b,c}. (a) Construct the…
A: Let S be a partially ordered set. Use the following definitions, to obtain the solution. Let a,b∈S…
Q: (d) Give an example of an equivalence relation on the set f1,2, 3} with exactly two equivalence…
A:
Q: Show that the set T := {(x, y) E Z × Z | x+y is even number } is an equivalence relation on the set…
A:
Q: Prove that the relation is an equivalence relation. 1. For x,y E R say x is congruent to y modulo Z…
A:
Q: Draw the Hasse diagram for the relation C on the set P({1,2,3}).
A: According to the given information, it is required to draw the hasse diagram for the given relation.
Q: Use a particular counterexample to explain why R fails to be an equivalence relation is the…
A: We take counter example   9R10,10R11⇒9R  11
Q: Let A # 0 be a set. Prove that A? is an equivalence relation on A.
A:
Q: Determine whether or not the following relations are equivalence relations on the given set. If the…
A:
Q: 2. Let H ≤ G and define = on G by a = b iff a¯¹b € H. Show that =µ is an equivalence relation.
A:
Q: 10. Let be the relation defind on Z by „R, if and only if a|b. Orve an explicit description of the…
A:
Q: R How to prove that " If is the equivalence B relation on the set and is the function R = IB then
A: given in question R is a equivalence relation on set B that means R is reflexive ,transitive  and…
Q: Let S be a nonempty set. Consider the relation ~ on P(S) defined byA ~ B if A U BC = S. Is the…
A: Let S be a nonempty set. Consider the relation ~ on P(S)  defined by A~B if A∪Bc=S To show: "~" is…
Q: Show that the relation R defined on the set of integers ℤ by (a,b) ∈ R if a – b = 6k for some k ∈ ℤ…
A:
Q: Prove that the given relation is an equivalence relation, and describe the distinct equivalence…
A: Let A is the set of all students at your college. For every x,y∈A,  xSy⇔x is the same age as y. That…
bartleby

Concept explainers

Set Theory
Every field in the present world involves a set. The theory of sets was developed by German mathematician Georg Cantor while working on the “problems of trigonometric series”.
Question

Subject: Set Theory

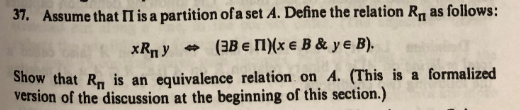
37. Assume that IT is a partition ofa set A. Define the relation Rn as follows: Show that Rn is an equivalence relation on A. (This is a formalized version of the discussion at the beginning of this section.)
expand button
Transcribed Image Text:37. Assume that IT is a partition ofa set A. Define the relation Rn as follows: Show that Rn is an equivalence relation on A. (This is a formalized version of the discussion at the beginning of this section.)
Expert Solution
Check Mark
bartleby

Step by stepSolved in 5 steps with 4 images

See solution
Check out a sample Q&A here
Blurred answer
Knowledge Booster
Advanced Math
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.
Similar questions
  • Let be a relation defined on the set of all integers by if and only if sum of and is odd. Decide whether or not is an equivalence relation. Justify your decision.
    Let (A) be the power set of the nonempty set A, and let C denote a fixed subset of A. Define R on (A) by xRy if and only if xC=yC. Prove that R is an equivalence relation on (A).
    • Recommended textbooks for you
  • Elements Of Modern Algebra
    Algebra
    ISBN:9781285463230
    Author:Gilbert, Linda, Jimmie
    Publisher:Cengage Learning,
    • Elements Of Modern Algebra
    Algebra
    ISBN:9781285463230
    Author:Gilbert, Linda, Jimmie
    Publisher:Cengage Learning,
    SEE MORE TEXTBOOKS