
Elements Of Electromagnetics
7th Edition
ISBN: 9780190698614
Author: Sadiku, Matthew N. O.
Publisher: Oxford University Press
expand_more
expand_more
format_list_bulleted
Concept explainers
Question
- Consider the single degree of freedom system in figure below:
- Make the correct selection: The system above is:
(A) Undamped (B) Underdamped (C) Critically damped (D) Overdamped
Also determine the following:
Also determine the following:
- Draw the Free-Body-Diagram (FBD) of the mass.
- Calculate the numerical value of the system’s natural frequency.
- Derive the equation-of-motion (EOM) using method of undetermined coefficients.
- Calculate the response of the system assuming it is initially at rest.
- Calculate the response of the system assuming it has an initial displacement of 0.05 m and starting from rest.
- Calculate the numerical value of the mass normalized force.
- Write the equation of motion above as a set of first-order ODE’s
- Write the first-order ODE’s in state-space form
- Write a Matlab code -using ODE45- to simulate (solve and plot) the system response above for part (f).
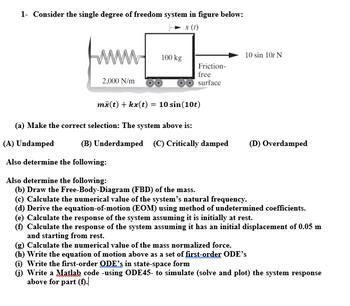
Transcribed Image Text:1- Consider the single degree of freedom system in figure below:
x (1)
www
2,000 N/m
100 kg
Friction-
free
surface
10 sin 10t N
mä(t) + kx(t) = 10 sin (10t)
(a) Make the correct selection: The system above is:
(A) Undamped
Also determine the following:
Also determine the following:
(b) Draw the Free-Body-Diagram (FBD) of the mass.
(c) Calculate the numerical value of the system's natural frequency.
(d) Derive the equation-of-motion (EOM) using method of undetermined coefficients.
(B) Underdamped (C) Critically damped
(D) Overdamped
(e) Calculate the response of the system assuming it is initially at rest.
(f) Calculate the response of the system assuming it has an initial displacement of 0.05 m
and starting from rest.
(g) Calculate the numerical value of the mass normalized force.
(h) Write the equation of motion above as a set of first-order ODE's
(i) Write the first-order ODE's in state-space form
(i) Write a Matlab code-using ODE45- to simulate (solve and plot) the system response
above for part (f).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 4 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- 2. Figure 2 shows a roller rolling and slipping at the same time on a slippery surface. The center of the roller moves with a displacement r(t) and the roller rotates with angle (t). The roller has mass m, mass moment of inertia I, and radius r. In addition, a layer of fluid with viscous damping coefficient c is present to lubricate the roller. Moreover, the roller is pulled by a spring under a given (i.e., prescribed) displacement u(t), whereas the spring has spring constant k. Also, the roller is subjected to an applied torque M(t). Figure 3 shows the free-body diagram of the roller. Answer the following questions. (a) Apply F = ma to derive an equation of motion governing the translation z(t). (b) Apply M = Ia to derive an equation of motion governing the rotation 0(t). (c) Eliminate the variable (t) from the two equations of motion derived in parts (a) and (b). You should obtain a third-order differential equation in x(t). (d) Initially (i.e., at t = 0), the roller has no…arrow_forwardQ4-6 pleasearrow_forwardFor the system shown in the figure, set up the equation of motion for the mass, m, and solve for the steady state amplitude and phase angle.arrow_forward
- A simple harmonic oscillator, of mass and natural frequency , experiences anoscillating driving force . Therefore, its equation of motion is where is its position. Given that at we have , , find thefunction . Describe the solution if is approximately, but not exactly, equal to .arrow_forward4. Problem 2: Consider the block diagram shown in the figure below. Determine the values of K and k such that the system has a damping ratio & = 0.7 and an undamped natural frequency wn = 4 rad/s. Please, use the block diagram algebra and the secondarrow_forwardPARTS A & B solve carefully and label each part and CIRCLE FINAL ANSWER ( Use image Below) Part A - Assuming the elements are all linear, determine a model for the mass-spring-damper system. Make sure to clearly define the direction of positive velocity. Simplify your answer such that the model is in terms of the parameters (?, ?, ??, ??), the input force (?), the spring deflection and the mass velocity as well as their time derivatives. Part B - Take the ANSWER from PART A and replace the damping constant ? with the nonlinear expression ? = ?? + ?? + ? , with ? as the velocity of the mass.arrow_forward
- The equation of motion of a certain mass-spring-damper system is 5x'' + cx' +10x = f(t). Suppose that f(t) = Fsin(wt). Define the magnitude ratio as M = X/F. Determine the natural frequency w_n, the peak frequency w_p, and the peak magnitude ratio M_p for damping ratio = 0.1 and the damping ratio = 0.3arrow_forward2. Consider the following compound pendulum, which consists of a thin rod attached to a pivot point 1/3 down its length, and has a thin disk of radius R attached to the end. Mass of the rod is m, and mass of the disk is me. Tasks: a. Find an Equation of Motion for rotational motion of the pendulum. Assume 0 <15° and apply the small angle approximation. b. Find the natural frequency w of this system. Report in terms of mr, ma, I, R, and g.arrow_forwardQ2: The system shown in Figure Q2 may be assumed to have rigid levers, no mass and frictionless pivot. For small vertical vibrations of the point mass: k C P Figure Q2 a) Derive expression for the damping ratio b) Derive expression for damped natural frequency c) Calculate the value of the critical damping coefficient m tarrow_forward
- 6. The electro-mechanical system shown below consists of an electric motor with input voltage V which drives inertia I in the mechanical system (see torque T). Find the governing differential equations of motion for this electro-mechanical system in terms of the input voltage to the motor and output displacement y. Electrical System puthiy C V V₁ R bac (0) T bac T Motor - Motor Input Voltage - Motor Back EMF = Kbac ( - Motor Angular Velocity - Motor Output Torque = K₂ i Kbacs K₁ - Motor Constants Mechanical System M T Frictionless Supportarrow_forwardis my answer entered correct ?arrow_forwardFigure below shows a single degree of freedom system. The system has a moment of inertia I =37 kg.m² about point O, the stiffness and damping values are k =548 N/m and C =113 N.s/m, respectively. Considering a =1.6 m and b =0.7 m, find the damped natural frequency of the system in rad/s. a b C wwwarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY

Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press

Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON

Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education

Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY

Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning

Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY