1. Prove that the series 7n (a) converges uniformly on every bounded interval (b) does not converge absolutely for any value of r 2. Suppose f is a real continuous function on R, n (t) (nt) and { n :n N) is equicontinuous on 3. Suppose is an equicontinuous sequence of functions on a compact set K, and {fn) converges . Let K be a compact metric space, and S CC(K), where C (K) is the space of all complex continuous 0,1. What conclusion can you draw about f? pointwise on K. Show that {fn converges uniformly functions on K, equipped with the metric d ( .9) = max EK | (x)-g(z) . Suppose that s is closed and pointwise bounded. Show that S is compact 5. Let {fn be a uniformly bounded sequence of functions which are Riemann integrable on [a, b, and put 岔 Fn (x)/fn (t) dt for a s sb 0 Show that there exists a subsequence {Fn^1 which converges uniformly on [a, b
1. Prove that the series 7n (a) converges uniformly on every bounded interval (b) does not converge absolutely for any value of r 2. Suppose f is a real continuous function on R, n (t) (nt) and { n :n N) is equicontinuous on 3. Suppose is an equicontinuous sequence of functions on a compact set K, and {fn) converges . Let K be a compact metric space, and S CC(K), where C (K) is the space of all complex continuous 0,1. What conclusion can you draw about f? pointwise on K. Show that {fn converges uniformly functions on K, equipped with the metric d ( .9) = max EK | (x)-g(z) . Suppose that s is closed and pointwise bounded. Show that S is compact 5. Let {fn be a uniformly bounded sequence of functions which are Riemann integrable on [a, b, and put 岔 Fn (x)/fn (t) dt for a s sb 0 Show that there exists a subsequence {Fn^1 which converges uniformly on [a, b
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN:9781133382119
Author:Swokowski
Publisher:Swokowski
Chapter10: Sequences, Series, And Probability
Section10.1: Infinite Sequences And Summation Notation
Problem 74E
Related questions
Question
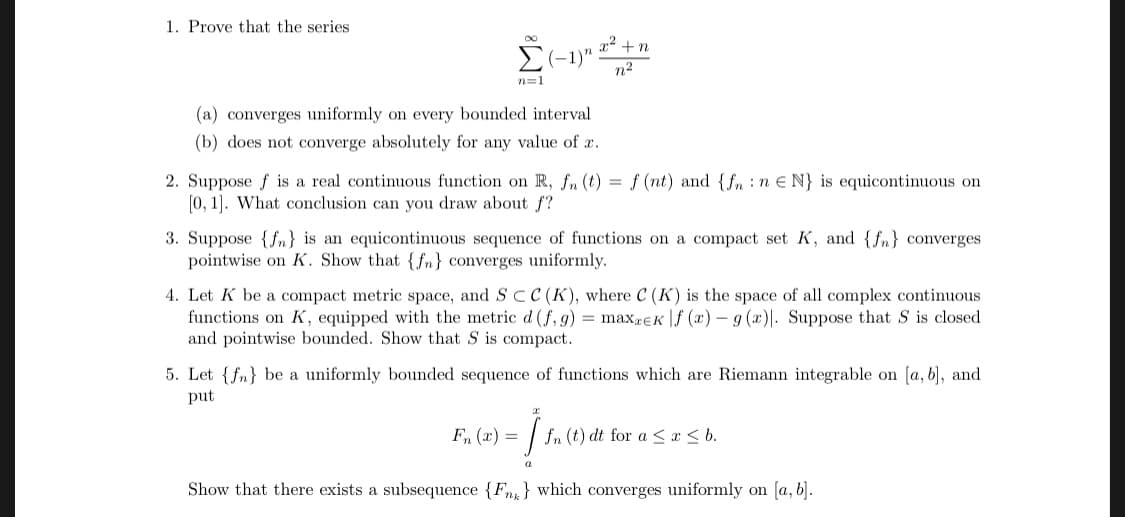
Transcribed Image Text:1. Prove that the series
7n
(a) converges uniformly on every bounded interval
(b) does not converge absolutely for any value of r
2. Suppose f is a real continuous function on R, n (t) (nt) and { n :n N) is equicontinuous on
3. Suppose is an equicontinuous sequence of functions on a compact set K, and {fn) converges
. Let K be a compact metric space, and S CC(K), where C (K) is the space of all complex continuous
0,1. What conclusion can you draw about f?
pointwise on K. Show that {fn converges uniformly
functions on K, equipped with the metric d ( .9) = max EK | (x)-g(z) . Suppose that s is closed
and pointwise bounded. Show that S is compact
5. Let {fn be a uniformly bounded sequence of functions which are Riemann integrable on [a, b, and
put
岔
Fn (x)/fn (t) dt for a s
sb
0
Show that there exists a subsequence {Fn^1 which converges uniformly on [a, b
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 5 steps with 4 images

Recommended textbooks for you

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage