2. Let V and W be vector spaces over a field F and let L: V → W be a linear transformation. Assume that dim(V) = n for some n e N. (a) Define ker(L), the kernel of L and im(L), the image of L. (b) Prove that ker(L) is a subspace of V. (c) If the rank of L is r, what is the dimension of the kernel of L? (d) Give an example of V, W and L where the rank r of L satisfies r = 0. (You can choose a value for n.)
2. Let V and W be vector spaces over a field F and let L: V → W be a linear transformation. Assume that dim(V) = n for some n e N. (a) Define ker(L), the kernel of L and im(L), the image of L. (b) Prove that ker(L) is a subspace of V. (c) If the rank of L is r, what is the dimension of the kernel of L? (d) Give an example of V, W and L where the rank r of L satisfies r = 0. (You can choose a value for n.)
Linear Algebra: A Modern Introduction
4th Edition
ISBN:9781285463247
Author:David Poole
Publisher:David Poole
Chapter6: Vector Spaces
Section6.4: Linear Transformations
Problem 24EQ
Related questions
Question
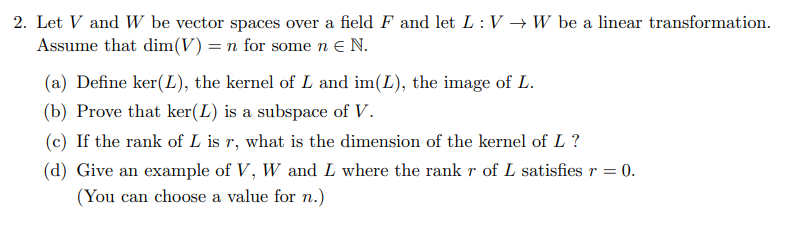
Transcribed Image Text:2. Let V and W be vector spaces over a field F and let L: V → W be a linear transformation.
Assume that dim(V) = n for some n e N.
(a) Define ker(L), the kernel of L and im(L), the image of L.
(b) Prove that ker(L) is a subspace of V.
(c) If the rank of L is r, what is the dimension of the kernel of L?
(d) Give an example of V, W and L where the rank r of L satisfies r = 0.
(You can choose a value for n.)
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:
9781305658004
Author:
Ron Larson
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:
9781305658004
Author:
Ron Larson
Publisher:
Cengage Learning