2n – 7 Prove that the sequence with nth u, = (a) is monotonic increasing, (b) is bounded above, (c) is Зп + 2 bounded below, (d) is bounded, (e) has a limit.
2n – 7 Prove that the sequence with nth u, = (a) is monotonic increasing, (b) is bounded above, (c) is Зп + 2 bounded below, (d) is bounded, (e) has a limit.
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN:9781133382119
Author:Swokowski
Publisher:Swokowski
Chapter10: Sequences, Series, And Probability
Section10.1: Infinite Sequences And Summation Notation
Problem 74E
Related questions
Question
#2.15) I have to explain each step of the solved problems in the picture.
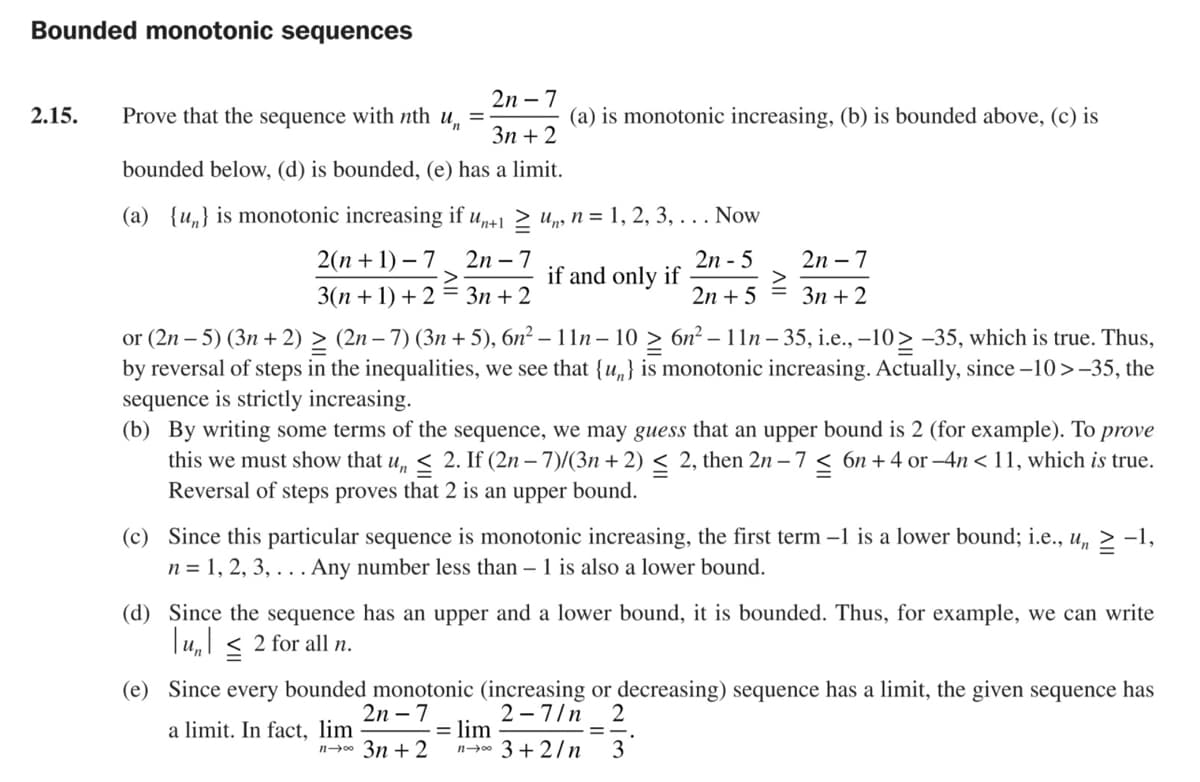
Transcribed Image Text:Bounded monotonic sequences
2n – 7
2.15.
Prove that the sequence with nth u,
(a) is monotonic increasing, (b) is bounded above, (c) is
Зп + 2
bounded below, (d) is bounded, (e) has a limit.
(a) {u„} is monotonic increasing if up+1 > up, n = 1, 2, 3, . . . Now
2(п + 1) — 7
2n – 7
2n - 5
if and only if
2n – 7
3(n + 1) + 2 = 3n + 2
2n + 5
Зп + 2
or (2n – 5) (3n + 2) > (2n – 7) (3n + 5), 6n² – 11n – 10 > 6n² – 11n – 35, i.e., –10> -35, which is true. Thus,
by reversal of steps in the inequalities, we see that {u,} is monotonic increasing. Actually, since –10>-35, the
sequence is strictly increasing.
(b) By writing some terms of the sequence, we may guess that an upper bound is 2 (for example). To prove
this we must show that u, < 2. If (2n – 7)/(3n + 2) < 2, then 2n – 7 < 6n + 4 or –4n < 11, which is true.
Reversal of steps proves that 2 is an upper bound.
(c) Since this particular sequence is monotonic increasing, the first term –1 is a lower bound; i.e., u,
n = 1, 2, 3, . .. Any number less than – 1 is also a lower bound.
-1,
(d) Since the sequence has an upper and a lower bound, it is bounded. Thus, for example, we can write
Tu,l < 2 for all n.
(e) Since every bounded monotonic (increasing or decreasing) sequence has a limit, the given sequence has
2
2 -7/n
lim
n- 3 + 2/n
2n – 7
a limit. In fact, lim
n0 3n + 2
3
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 2 steps

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Recommended textbooks for you

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage