Atwood's machine consists of a smooth pulley with two masses suspended from a light string at each end (Figure 2-11). Find the acceleration of the masses and the tension of the string (a) when the pulley center is at rest and (b) when the pulley is descending in an elevator with constant acceleration a. Fixed Elevator mig m2 (b)
Atwood's machine consists of a smooth pulley with two masses suspended from a light string at each end (Figure 2-11). Find the acceleration of the masses and the tension of the string (a) when the pulley center is at rest and (b) when the pulley is descending in an elevator with constant acceleration a. Fixed Elevator mig m2 (b)
Calculus: Early Transcendentals
8th Edition
ISBN:9781285741550
Author:James Stewart
Publisher:James Stewart
Chapter1: Functions And Models
Section: Chapter Questions
Problem 1RCC: (a) What is a function? What are its domain and range? (b) What is the graph of a function? (c) How...
Related questions
Question
Show the step by step equations
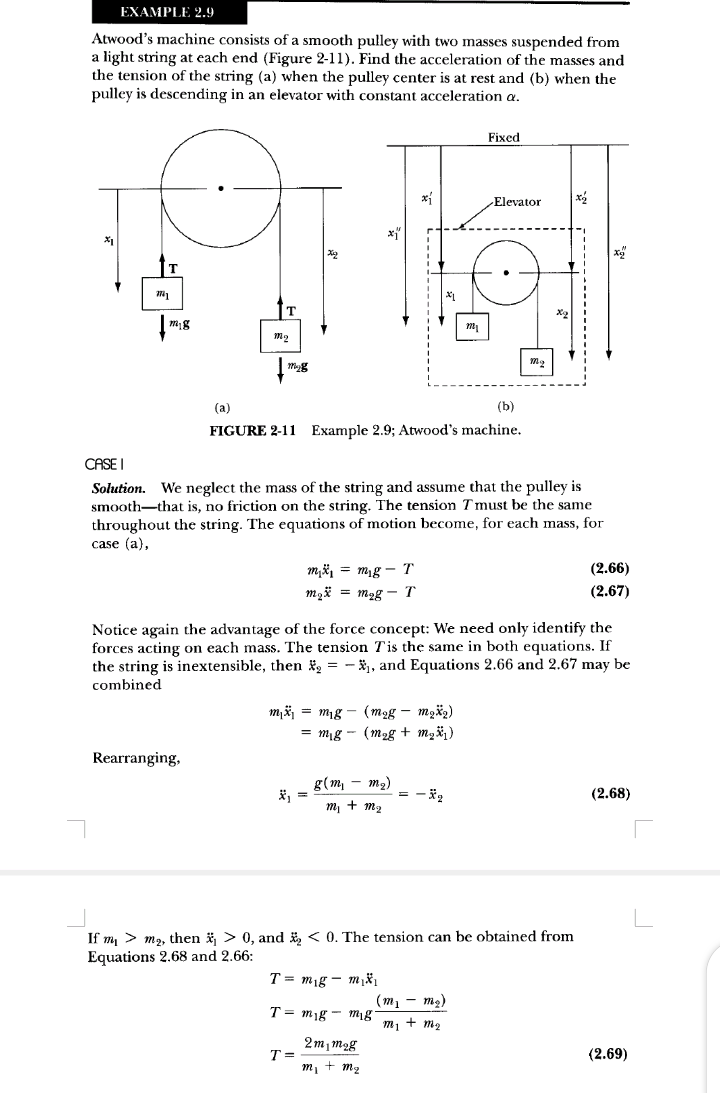
Transcribed Image Text:EXAMPLE 2.9
Atwood's machine consists of a smooth pulley with two masses suspended from
a light string at each end (Figure 2-11). Find the acceleration of the masses and
the tension of the string (a) when the pulley center is at rest and (b) when the
pulley is descending in an elevator with constant acceleration a.
Fixed
Elevator
T
(a)
(b)
FIGURE 2-11 Example 2.9; Atwood's machine.
CASE I
Solution. We neglect the mass of the string and assume that the pulley is
smooth-that is, no friction on the string. The tension Tmust be the same
throughout the string. The equations of motion become, for each mass, for
case (a),
m,k, = mg - T
m2ä = mag – T
(2.66)
(2.67)
Notice again the advantage of the force concept: We need only identify the
forces acting on each mass. The tension T is the same in both equations. If
the string is inextensible, then * = - , and Equations 2.66 and 2.67 may be
combined
m,ä = mig – (mog – mgž2)
= mig - (mog + m2ä)
Rearranging,
g(m, - m2)
*, =
= -*,
(2.68)
т + т,
If m, > mg, then ä, > 0, and #, < 0. The tension can be obtained from
Equations 2.68 and 2.66:
T = mig - m
(m, - mg)
T= mg - mig
m, + m2
2m, mog
T =
m, + m2
(2.69)
---
---------
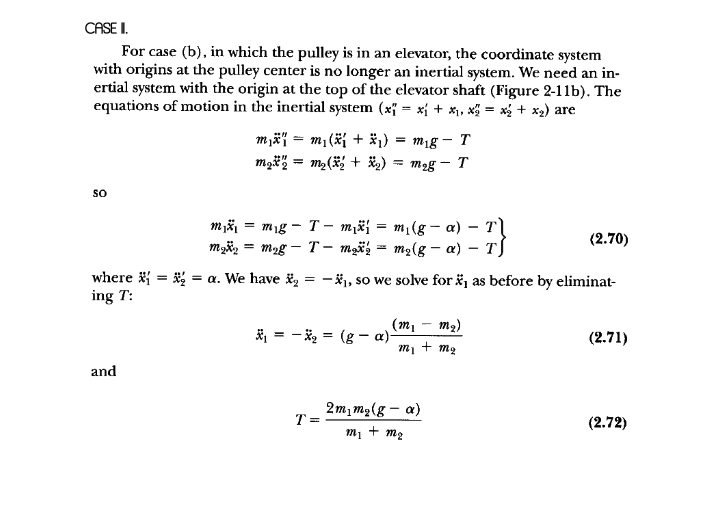
Transcribed Image Text:CASE I.
For case (b), in which the pulley is in an elevator, the coordinate system
with origins at the pulley center is no longer an inertial system. We need an in-
ertial system with the origin at the top of the elevator shaft (Figure 2-11b). The
equations of motion in the inertial system (x = xi + x, xg = x + x2) are
mä¡ = m, (* + #1)
%3D
mig - T
M2g - T
so
mig - T- mží = m1(g – a) – T
ma = mag – T- mgäž = m2(g – æ) – TJ
(2.70)
where * = = a. We have ä,
ing T:
- #1, so we solve for ä, as before by eliminat-
(m,
* = - * = (g – a)-
m2)
(2.71)
m, + mg
and
2m, my(g – a)
T =
(2.72)
m1 + m2
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781319050740
Author:
Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:
W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:
9781337552516
Author:
Ron Larson, Bruce H. Edwards
Publisher:
Cengage Learning