Homework Help is Here – Start Your Trial Now!
Math
Probability
B A Figure 3.2 Figure 3.3 (3.6) Р(А+ B) — Р(А) + P(B) — Р(АВ). To see why this is true, consider Figure 3.2. To find P(A+ B) we add the probabil- ities of all the sample points in the region consisting of A or B or both. But if we add P(A) and P(B), we have included the probabilities of all the sample points in AB twice [once in P(A) and once in P(B)]. Thus we must subtract P(AB), which is the sum of the probabilities of all the sample points in AB. This is just what (3.6) says. If the sample space diagram is like the one in Figure 3.3, so that P(AB) = 0, we say that A and B are mutually exclusive. Then (3.6) becomes (3.7) P(A+B) = P(A) + P(B), A and B mutually exclusive. Example 1. (a) In three tosses of a coin, what is the probability that all three are heads? We found p = for this problem in Section 2 by seeing that one sample point out of eight corresponds to all heads. Now we can do the problem more simply by saying that the probability of heads on each toss is ;, the tosses are independent, and therefore 1 1 1 p = 1 I| 2 2 2 8 (b) If we should want the probability of all heads when a coin is tossed ten times, the sample space would be unwieldy; instead of using the sample space, we can say that since the tosses are independent, the desired probability is p= (c) To find the probability of at least one tail in ten tosses, we see that this event corresponds to all the rest of the sample space except the "all heads" point. Since the sum of the probabilities of all the sample points is 1, the desired probability is 1- ()10. In Figure 3.1 or Figure 3.2 the region AB corresponds to the happening of both A and B. The whole region consisting of points in A or B or both corresponds to the happening of either A or B or both. We write P(AB) for the probability that both A and B occur. We shall write P(A+B) for the probability that either or both occur. Then we can prove that

B A Figure 3.2 Figure 3.3 (3.6) Р(А+ B) — Р(А) + P(B) — Р(АВ). To see why this is true, consider Figure 3.2. To find P(A+ B) we add the probabil- ities of all the sample points in the region consisting of A or B or both. But if we add P(A) and P(B), we have included the probabilities of all the sample points in AB twice [once in P(A) and once in P(B)]. Thus we must subtract P(AB), which is the sum of the probabilities of all the sample points in AB. This is just what (3.6) says. If the sample space diagram is like the one in Figure 3.3, so that P(AB) = 0, we say that A and B are mutually exclusive. Then (3.6) becomes (3.7) P(A+B) = P(A) + P(B), A and B mutually exclusive. Example 1. (a) In three tosses of a coin, what is the probability that all three are heads? We found p = for this problem in Section 2 by seeing that one sample point out of eight corresponds to all heads. Now we can do the problem more simply by saying that the probability of heads on each toss is ;, the tosses are independent, and therefore 1 1 1 p = 1 I| 2 2 2 8 (b) If we should want the probability of all heads when a coin is tossed ten times, the sample space would be unwieldy; instead of using the sample space, we can say that since the tosses are independent, the desired probability is p= (c) To find the probability of at least one tail in ten tosses, we see that this event corresponds to all the rest of the sample space except the "all heads" point. Since the sum of the probabilities of all the sample points is 1, the desired probability is 1- ()10. In Figure 3.1 or Figure 3.2 the region AB corresponds to the happening of both A and B. The whole region consisting of points in A or B or both corresponds to the happening of either A or B or both. We write P(AB) for the probability that both A and B occur. We shall write P(A+B) for the probability that either or both occur. Then we can prove that

Algebra & Trigonometry with Analytic Geometry
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN:9781133382119
Author:Swokowski
Publisher:Swokowski
Chapter10: Sequences, Series, And Probability
Section10.8: Probability
Problem 32E
See similar textbooks
icon
Related questions
Question
B A Figure 3.2 Figure 3.3 (3.6) Р(А+ B) — Р(А) + P(B) — Р(АВ). To see why this is true, consider Figure 3.2. To find P(A+ B) we add the probabil- ities of all the sample points in the region consisting of A or B or both. But if we add P(A) and P(B), we have included the probabilities of all the sample points in AB twice [once in P(A) and once in P(B)]. Thus we must subtract P(AB), which is the sum of the probabilities of all the sample points in AB. This is just what (3.6) says. If the sample space diagram is like the one in Figure 3.3, so that P(AB) = 0, we say that A and B are mutually exclusive. Then (3.6) becomes (3.7) P(A+B) = P(A) + P(B), A and B mutually exclusive.
Transcribed Image Text:B A Figure 3.2 Figure 3.3 (3.6) Р(А+ B) — Р(А) + P(B) — Р(АВ). To see why this is true, consider Figure 3.2. To find P(A+ B) we add the probabil- ities of all the sample points in the region consisting of A or B or both. But if we add P(A) and P(B), we have included the probabilities of all the sample points in AB twice [once in P(A) and once in P(B)]. Thus we must subtract P(AB), which is the sum of the probabilities of all the sample points in AB. This is just what (3.6) says. If the sample space diagram is like the one in Figure 3.3, so that P(AB) = 0, we say that A and B are mutually exclusive. Then (3.6) becomes (3.7) P(A+B) = P(A) + P(B), A and B mutually exclusive.
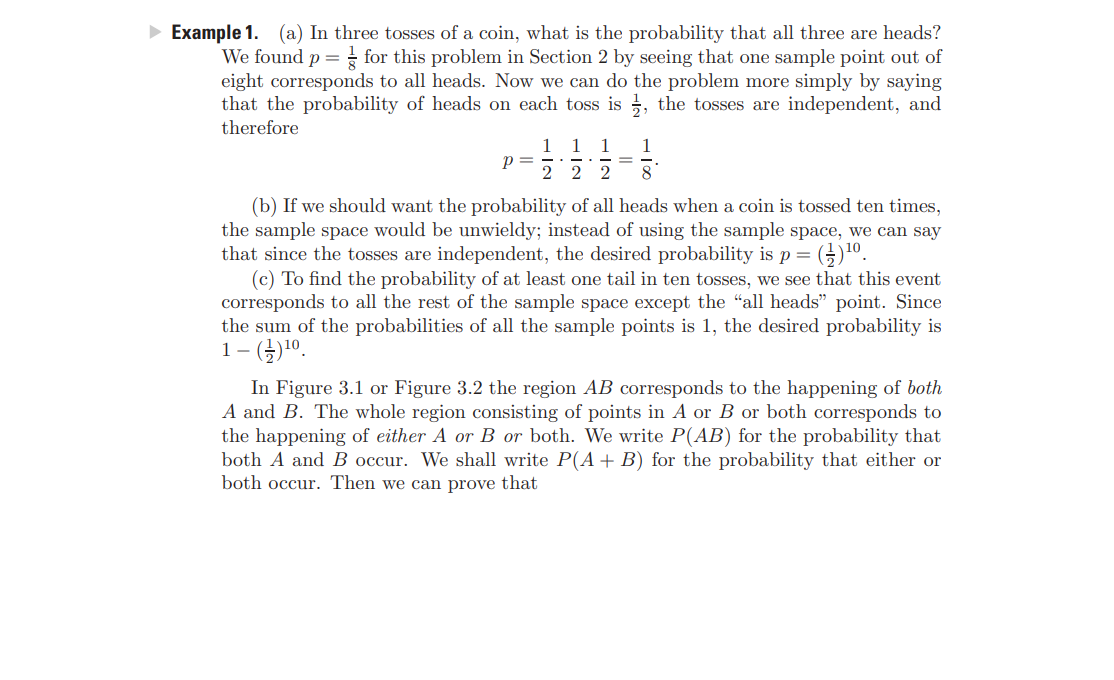
Example 1. (a) In three tosses of a coin, what is the probability that all three are heads? We found p = for this problem in Section 2 by seeing that one sample point out of eight corresponds to all heads. Now we can do the problem more simply by saying that the probability of heads on each toss is ;, the tosses are independent, and therefore 1 1 1 p = 1 I| 2 2 2 8 (b) If we should want the probability of all heads when a coin is tossed ten times, the sample space would be unwieldy; instead of using the sample space, we can say that since the tosses are independent, the desired probability is p= (c) To find the probability of at least one tail in ten tosses, we see that this event corresponds to all the rest of the sample space except the "all heads" point. Since the sum of the probabilities of all the sample points is 1, the desired probability is 1- ()10. In Figure 3.1 or Figure 3.2 the region AB corresponds to the happening of both A and B. The whole region consisting of points in A or B or both corresponds to the happening of either A or B or both. We write P(AB) for the probability that both A and B occur. We shall write P(A+B) for the probability that either or both occur. Then we can prove that
Transcribed Image Text:Example 1. (a) In three tosses of a coin, what is the probability that all three are heads? We found p = for this problem in Section 2 by seeing that one sample point out of eight corresponds to all heads. Now we can do the problem more simply by saying that the probability of heads on each toss is ;, the tosses are independent, and therefore 1 1 1 p = 1 I| 2 2 2 8 (b) If we should want the probability of all heads when a coin is tossed ten times, the sample space would be unwieldy; instead of using the sample space, we can say that since the tosses are independent, the desired probability is p= (c) To find the probability of at least one tail in ten tosses, we see that this event corresponds to all the rest of the sample space except the "all heads" point. Since the sum of the probabilities of all the sample points is 1, the desired probability is 1- ()10. In Figure 3.1 or Figure 3.2 the region AB corresponds to the happening of both A and B. The whole region consisting of points in A or B or both corresponds to the happening of either A or B or both. We write P(AB) for the probability that both A and B occur. We shall write P(A+B) for the probability that either or both occur. Then we can prove that
Expert Solution
trending now

Trending now

This is a popular solution!

steps

Step by step

Solved in 3 steps

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Recommended textbooks for you
Algebra & Trigonometry with Analytic Geometry
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage
SEE MORE TEXTBOOKS