Check if 53 is a primitive root of Z151 by: (a) Theorem 6.8 Suppose that P element modulo Р (6) Euler's Criterion > 2 is a prime and a E Zp". Then a is a primitive (p-1)/a_ ² = 1 (mod p) for all primes a such that a I (p-1- of and only if a
Check if 53 is a primitive root of Z151 by: (a) Theorem 6.8 Suppose that P element modulo Р (6) Euler's Criterion > 2 is a prime and a E Zp". Then a is a primitive (p-1)/a_ ² = 1 (mod p) for all primes a such that a I (p-1- of and only if a
Elements Of Modern Algebra
8th Edition
ISBN:9781285463230
Author:Gilbert, Linda, Jimmie
Publisher:Gilbert, Linda, Jimmie
Chapter2: The Integers
Section2.4: Prime Factors And Greatest Common Divisor
Problem 19E: Prove that if n is a positive integer greater than 1 such that n is not a prime, then n has a...
Related questions
Question

Transcribed Image Text:Check if 53 is a primitive root of Z₁51
by:
(a) Theorem 6.8 - Suppose that p > 2 is a prime and a E Zp". Then a is a primitive
(p-1)/a
element modulo
² = 1 (mod p) for all primes a such that a I (p-1).
P
of and only if a
(6) Euler's Criterion
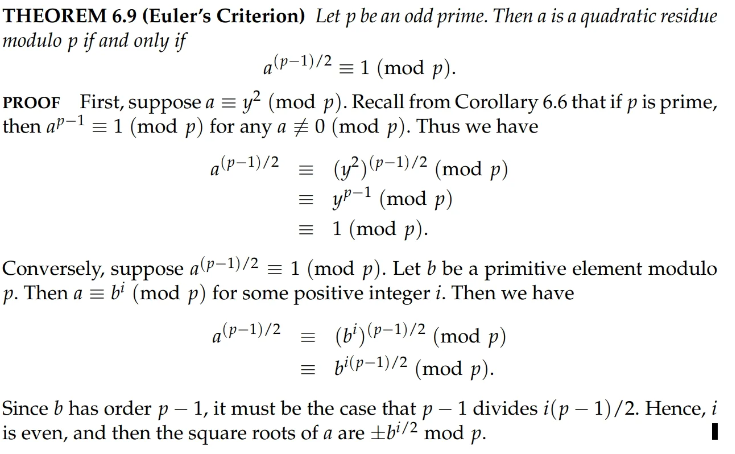
Transcribed Image Text:THEOREM 6.9 (Euler's Criterion) Let p be an odd prime. Then a is a quadratic residue
modulo p if and only if
a(p-1)/2 = 1 (mod p).
PROOF First, suppose a = y² (mod p). Recall from Corollary 6.6 that if p is prime,
then ap−¹ = 1 (mod p) for any a #0 (mod p). Thus we have
a(p-1)/2 =
=
=
(y²)(p-1)/2 (mod p)
yp-¹ (mod p)
1 (mod p).
Conversely, suppose a(p-1)/2 = 1 (mod p). Let b be a primitive element modulo
p. Then a = b¹ (mod p) for some positive integer i. Then we have
a(p-1)/2 =
(bi) (p-1)/2 (mod p)
= fi(p-1)/2 (mod p).
Since b has order p - 1, it must be the case that p - 1 divides i(p-1)/2. Hence, i
is even, and then the square roots of a are ±b¹/2 mod p.
I
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage