Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Related questions
Question
thumb_up100%
Could you explain how to show 7.8 in detail? I also included lists of definitions and theorems in the book as a reference.
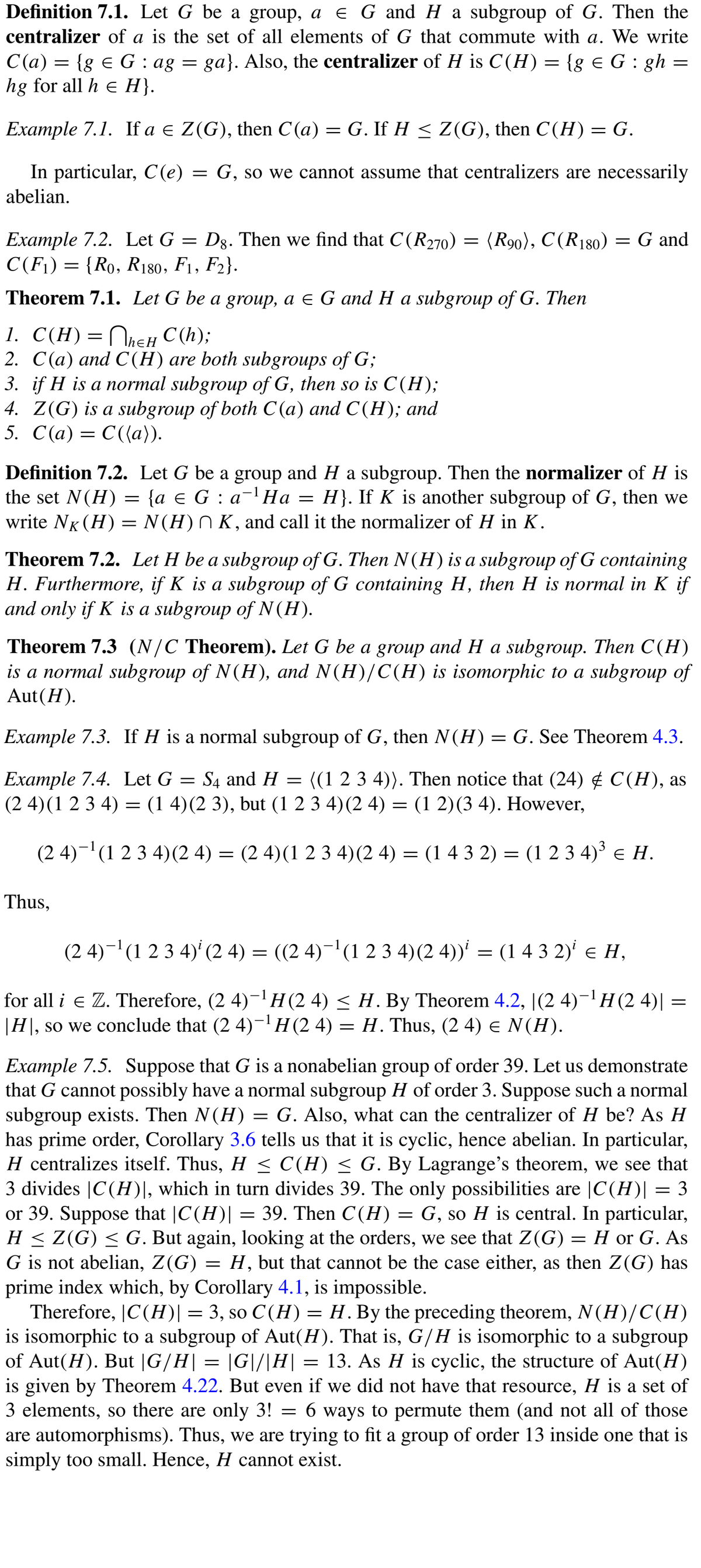
Transcribed Image Text:Definition 7.1. Let G be a group, a e G and H a subgroup of G. Then the
centralizer of a is the set of all elements of G that commute with a. We write
C(a) = {g € G : ag = ga}. Also, the centralizer of H is C(H) = {g e G : gh =
hg for all h e H}.
Example 7.1. If a e Z(G), then C(a) = G. If H < Z(G), then C(H) = G.
In particular, C(e)
= G, so we cannot assume that centralizers are necessarily
abelian.
Example 7.2. Let G = Dg. Then we find that C(R270) = (R90), C(R180) = G and
C(F)) = {Ro, R180, F1, F2}.
Theorem 7.1. Let G be a group, a e G and H a subgroup of G. Then
1. С(Н) 3D Плeн С (h);
2. C(a) and C(H) are both subgroups of G;
3. if H is a normal subgroup of G, then so is C(H);
4. Z(G) is a subgroup of both C(a) and C(H); and
5. С (а) — С(а)).
Definition 7.2. Let G be a group and H a subgroup. Then the normalizer of H is
the set N(H) = {a e G : a-Ha = H}. If K is another subgroup of G, then we
write NK (H) = N(H) N K, and call it the normalizer of H in K.
Theorem 7.2. Let H be a subgroup of G. Then N(H) is a subgroup of G containing
H. Furthermore, if K is a subgroup of G containing H, then H is normal in K if
and only if K is a subgroup of N (H).
Theorem 7.3 (N/C Theorem). Let G be a group and H a subgroup. Then C(H)
is a normal subgroup of N(H), and N(H)/C(H) is isomorphic to a subgroup of
Aut(H).
Example 7.3. If H is a normal subgroup of G, then N(H) = G. See Theorem 4.3.
Example 7.4. Let G = S4 and H = ((1 2 3 4)). Then notice that (24) ¢ C(H), as
(2 4)(1 2 3 4) = (1 4)(2 3), but (1 2 3 4)(2 4) = (1 2)(3 4). However,
(2 4)-(1 2 3 4)(2 4) = (2 4)(1 2 3 4)(2 4) = (1 4 3 2) = (1 2 3 4)³ e H.
Thus,
(2 4)-'(1 2 3 4)' (2 4) = ((2 4)-'(1 2 3 4)(2 4))' = (1 4 3 2)' e H,
for all i e Z. Therefore, (2 4)-H(2 4) < H. By Theorem 4.2, |(2 4)-'H (2 4)||
|H|, so we conclude that (2 4)-H(2 4) = H. Thus, (2 4) e N(H).
Example 7.5. Suppose that G is a nonabelian group of order 39. Let us demonstrate
that G cannot possibly have a normal subgroup H of order 3. Suppose such a normal
subgroup exists. Then N(H) = G. Also, what can the centralizer of H be? As H
has prime order, Corollary 3.6 tells us that it is cyclic, hence abelian. In particular,
H centralizes itself. Thus, H < C(H) < G. By Lagrange's theorem, we see that
3 divides |C(H)I, which in turn divides 39. The only possibilities are |C(H)| = 3
or 39. Suppose that |C(H)| = 39. Then C(H) = G, so H is central. In particular,
H < Z(G) < G. But again, looking at the orders, we see that Z(G) =
G is not abelian, Z(G) = H, but that cannot be the case either, as then Z(G) has
prime index which, by Corollary 4.1, is impossible.
Therefore, |C(H)|= 3, so C(H)= H.By the preceding theorem, N(H)/C(H)
is isomorphic to a subgroup of Aut(H). That is, G/H is isomorphic to a subgroup
of Aut(H). But |G/H| = |G|/|H| = 13. As H is cyclic, the structure of Aut(H)
is given by Theorem 4.22. But even if we did not have that resource, H is a set of
3 elements, so there are only 3! = 6 ways to permute them (and not all of those
are automorphisms). Thus, we are trying to fit a group of order 13 inside one that is
simply too small. Hence, H cannot exist.
H or G. As
Transcribed Image Text:7.2. Which permutations lie in the centralizer of (1 2 3) in S3?
7.8. If a e G and a has odd order, show that C (a) = C(a*).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 2 images
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
- Part three was incorect. I was told you cannot do the computatiopns without substituting in the T and F Values first. Can you do show me how to do it that way?arrow_forwardShow, without a calculator, that 8 sinsinsin = √3arrow_forwardUse the dot method to add the numbers below. If the number needs a dot, put a dot (period) to the right of the number. Otherwise leave it blank.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY 
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,