Calculus: Early Transcendentals
8th Edition
ISBN: 9781285741550
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Related questions
Concept explainers
Question
![**Title: Finding the Point of Intersection of Two Lines in R²**
**Problem Statement:**
The goal is to find the point of intersection of the lines defined by the parametric equations:
\[ \mathbf{r_1}(t) = \langle -1, 1 \rangle + t \langle 8, 4 \rangle \]
and
\[ \mathbf{r_2}(s) = \langle 2, 1 \rangle + s \langle 8, 6 \rangle \]
in \(\mathbb{R}^2\).
**Expression for Point of Intersection:**
\[ (x, y) = \left( \text{input box} \right) \]
**Explanation:**
We need to determine the values of \(t\) and \(s\) for which the two parametric equations yield the same coordinates \((x, y)\).
1. **Equation (1) from \(\mathbf{r_1}(t)\):**
\[
x_1(t) = -1 + 8t
\]
\[
y_1(t) = 1 + 4t
\]
2. **Equation (2) from \(\mathbf{r_2}(s)\):**
\[
x_2(s) = 2 + 8s
\]
\[
y_2(s) = 1 + 6s
\]
To find the intersection point, set \(x_1(t) = x_2(s)\) and \(y_1(t) = y_2(s)\):
\[
-1 + 8t = 2 + 8s \tag{1}
\]
\[
1 + 4t = 1 + 6s \tag{2}
\]
Solving these equations will yield the values of \(t\) and \(s\) for which the lines intersect. The corresponding coordinates can then be obtained by substituting \(t\) back into \(\mathbf{r_1}(t)\) or \(\mathbf{r_2}(s)\).
**Submission Box:**
Here, the coordinates of the intersection point can be entered:
\[ (x, y) = \left( \begin{matrix}
\ \\
\ \\
\end{matrix} \](https://content.bartleby.com/qna-images/question/c3d3bee5-0c39-4b7b-a553-642953afa184/d0ec6207-8ba6-4be5-8ef1-589b916e12c5/0o5ro2m.png)
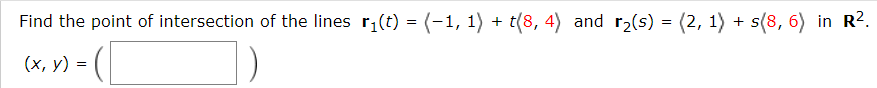
Transcribed Image Text:**Title: Finding the Point of Intersection of Two Lines in R²**
**Problem Statement:**
The goal is to find the point of intersection of the lines defined by the parametric equations:
\[ \mathbf{r_1}(t) = \langle -1, 1 \rangle + t \langle 8, 4 \rangle \]
and
\[ \mathbf{r_2}(s) = \langle 2, 1 \rangle + s \langle 8, 6 \rangle \]
in \(\mathbb{R}^2\).
**Expression for Point of Intersection:**
\[ (x, y) = \left( \text{input box} \right) \]
**Explanation:**
We need to determine the values of \(t\) and \(s\) for which the two parametric equations yield the same coordinates \((x, y)\).
1. **Equation (1) from \(\mathbf{r_1}(t)\):**
\[
x_1(t) = -1 + 8t
\]
\[
y_1(t) = 1 + 4t
\]
2. **Equation (2) from \(\mathbf{r_2}(s)\):**
\[
x_2(s) = 2 + 8s
\]
\[
y_2(s) = 1 + 6s
\]
To find the intersection point, set \(x_1(t) = x_2(s)\) and \(y_1(t) = y_2(s)\):
\[
-1 + 8t = 2 + 8s \tag{1}
\]
\[
1 + 4t = 1 + 6s \tag{2}
\]
Solving these equations will yield the values of \(t\) and \(s\) for which the lines intersect. The corresponding coordinates can then be obtained by substituting \(t\) back into \(\mathbf{r_1}(t)\) or \(\mathbf{r_2}(s)\).
**Submission Box:**
Here, the coordinates of the intersection point can be entered:
\[ (x, y) = \left( \begin{matrix}
\ \\
\ \\
\end{matrix} \
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 4 steps with 4 images
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Recommended textbooks for you
- Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON 
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning
Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON
Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman
Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning