Informal Exercise 16. Draw a picture of a number line representing F. Draw b and c in the above proof, and indicate the sets defined by |r – b| < ɛ and |r – e| < e where e is as in the above proof. Observe that the sets do not intersect so there can be no a; simultaneously in both, which is why we got a contradiction. This explains why we chose ɛ = could have chosen ɛ = (c – b)/4, for instance, and obtained a contradiction. However, e = 2(c – b) does not work. Why not? (c - b)/2. Note, we
Informal Exercise 16. Draw a picture of a number line representing F. Draw b and c in the above proof, and indicate the sets defined by |r – b| < ɛ and |r – e| < e where e is as in the above proof. Observe that the sets do not intersect so there can be no a; simultaneously in both, which is why we got a contradiction. This explains why we chose ɛ = could have chosen ɛ = (c – b)/4, for instance, and obtained a contradiction. However, e = 2(c – b) does not work. Why not? (c - b)/2. Note, we
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN:9781133382119
Author:Swokowski
Publisher:Swokowski
Chapter10: Sequences, Series, And Probability
Section: Chapter Questions
Problem 63RE
Related questions
Question
Informal Exercise 16.
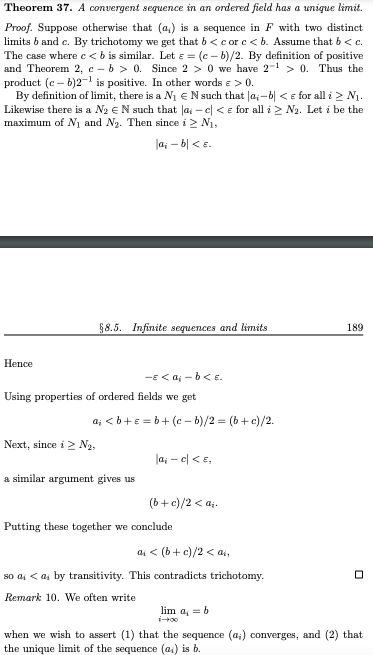
Transcribed Image Text:Theorem 37. A convergent seguence in an ordered field has a unique limit.
Proof. Suppose otherwise that (a,) is a sequence in F with two distinct
limits b and c. By trichotomy we get that b<c ore<b. Assume that b<c.
The case where c < b is similar. Let e = (c – b)/2. By definition of positive
and Theorem 2, c - b > 0. Sinoe 2 > 0 we have 2-1 > 0. Thus the
product (c – b)2-1 is positive. In other words e > 0.
By definition of limit, there is a N1 € N such that la;-b| < e for all i > N1.
Likewise there is a N2 €N such that a – c| < e for all i > Na. Let i be the
maximum of N1 and N3. Then since i2 Ni,
la; - 6| < e.
$8.5. Infinite sequences and limits
189
Hence
-E < a; - b< E.
Using properties of ordered fields we get
a; < b+e = b+ (e – b)/2 = (b+ c)/2.
Next, since i2 Ng,
la; – c| < E,
a similar argument gives us
(b+c)/2 < aj.
Putting these together we conclude
as < (b+ c)/2 < ai,
so a, < a, by transitivity. This contradicts trichotomy.
Remark 10. We often write
lim a, = b
when we wish to assert (1) that the sequence (a;) converges, and (2) that
the unique limit of the sequence (a.) is b.

Transcribed Image Text:Informal Exercise 16. Draw a picture of a number line representing F.
Draw b and c in the above proof, and indicate the sets defined by |r – b| < e
and |r – e| < e where e is as in the above proof. Observe that the sets do
not intersect so there can be no a; simultaneously in both, which is why we
got a contradiction. This explains why we chose e = (c – b)/2. Note, we
could have chosen ɛ = (c- b)/4, for instance, and obtained a contradiction.
However, e = 2(c – b) does not work. Why not?
%3D
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 1 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Recommended textbooks for you

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage