is a bounded sequence in H and since A is a compact operator, there is a subsequence (Yn₁) such that (A(Yn;)) converges in H. For j, k 1, 2, ..., we have = ||A*(xn;) — A*(xnx) || ² = = = (A*(xn, - Ink), A* (In, — Ink)) - (AA*(Znj – Ink), In, nh) (A(yn,) - A(ynk), In, - Ink) ≤ 2a||A(yn,) - A(Ynk)||. Request exple this step
is a bounded sequence in H and since A is a compact operator, there is a subsequence (Yn₁) such that (A(Yn;)) converges in H. For j, k 1, 2, ..., we have = ||A*(xn;) — A*(xnx) || ² = = = (A*(xn, - Ink), A* (In, — Ink)) - (AA*(Znj – Ink), In, nh) (A(yn,) - A(ynk), In, - Ink) ≤ 2a||A(yn,) - A(Ynk)||. Request exple this step
Elements Of Modern Algebra
8th Edition
ISBN:9781285463230
Author:Gilbert, Linda, Jimmie
Publisher:Gilbert, Linda, Jimmie
Chapter5: Rings, Integral Domains, And Fields
Section5.4: Ordered Integral Domains
Problem 1E: Complete the proof of Theorem 5.30 by providing the following statements, where and are arbitrary...
Related questions
Question
100%
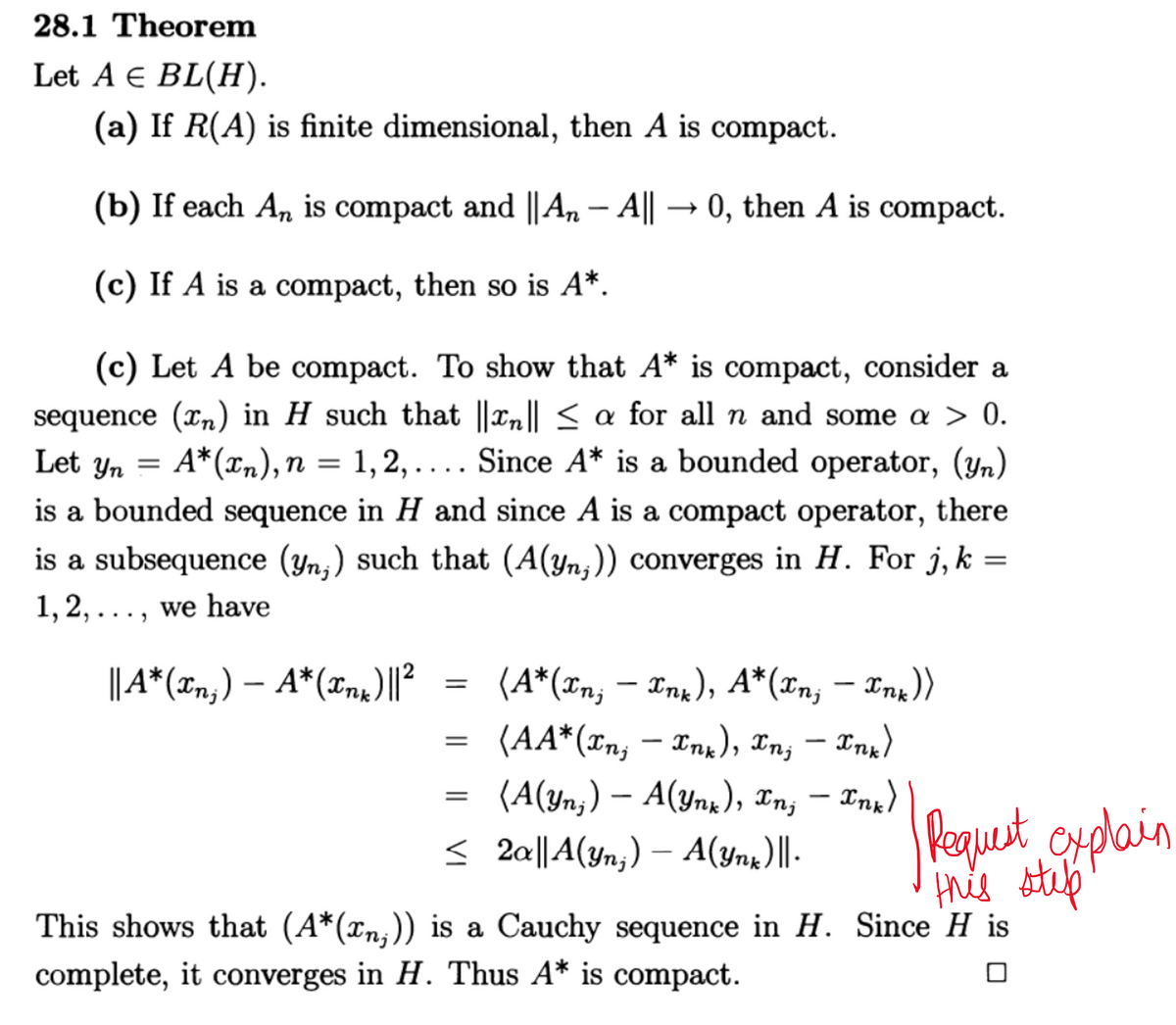
Transcribed Image Text:28.1 Theorem
Let A € BL(H).
(a) If R(A) is finite dimensional, then A is compact.
(b) If each An is compact and || An – A|| → 0, then A is compact.
(c) If A is a compact, then so is A*.
(c) Let A be compact. To show that A* is compact, consider a
sequence (n) in H such that ||xn|| ≤ a for all n and some a > 0.
Let Yn
A*(xn), n = 1,2,.... Since A* is a bounded operator, (yn)
is a bounded sequence in H and since A is a compact operator, there
is a subsequence (yn;) such that (A(Yn;)) converges in H. For j, k
1, 2, ..., we have
||A*(xn;) — A*(xnx)||²
=
-
(A*(xn; — Xnk), A*(Xn; — Xnx))
=
(AA* (In, Ink), In, — Xnx)
-
(A(Yn;) — A(Ynk), xn; — xnx)
≤ 2a||A(yn;) — A(Ynk)||.
explain
Request
This shows that (A*(xn;)) is a Cauchy sequence in H. Since H is
complete, it converges in H. Thus A* is compact.
this stulp
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 2 steps with 1 images

Recommended textbooks for you

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage