File Preview (d) First, we just need one solution to each, then we can form solution sets by adding all the multiples of the basis for the kernel. Since (2,0,0)¯ = 2ā1 +0ã2 + 0ã3 A0 = 0 and so the full solution set to the first is 1 +c 1 : CER For the second equation, notice (0, 1,0)¯ = −ã1 + ã2 + 0ã3 and so A = 0-8 R}. and so the full solution set for the second is + c : CER For the final equation, notice that (2,1,0) is the last column of A and Aе3 and so one solution is (0,0,1) and so the full solution set is +c 1 : CER = ძვ Let A: = 1 1 2 011 0 0 | (a) Find a basis for ran(A). What is dim(ran(A))? (b) Use the Rank-Nullity theorem to find dim (ker (A)). (c) Find a basis for ker(A). (d) Use the basis from (c) to write the solution sets to each of the following: Ax = 0 Ay= |1 Az= Solution. Let ₁ be the first column of A, 2 the second, and a3 the third column of A. (a) Since σ ₁ and 2 are not multiples of one another, they are linearly independent, but ã₁ + a2 = ã3 and so a basis for ran(A) is {ā₁, ā2} and so dim(ran(A)) = 2. (b) The rank-nullity theorem gives 3 = dim(ran(A)) + dim(ker(A)) and since dim(ran(A)) = 2, dim(ker(A)) = 1. (c) Since dim(ker(A)) = 1, we just need one nonzero element of the kernel. Since a1a2a3 = 0, so a basis for ker(A) is (1, 1, −1). = = α1 + α2 − α3 = 0
File Preview (d) First, we just need one solution to each, then we can form solution sets by adding all the multiples of the basis for the kernel. Since (2,0,0)¯ = 2ā1 +0ã2 + 0ã3 A0 = 0 and so the full solution set to the first is 1 +c 1 : CER For the second equation, notice (0, 1,0)¯ = −ã1 + ã2 + 0ã3 and so A = 0-8 R}. and so the full solution set for the second is + c : CER For the final equation, notice that (2,1,0) is the last column of A and Aе3 and so one solution is (0,0,1) and so the full solution set is +c 1 : CER = ძვ Let A: = 1 1 2 011 0 0 | (a) Find a basis for ran(A). What is dim(ran(A))? (b) Use the Rank-Nullity theorem to find dim (ker (A)). (c) Find a basis for ker(A). (d) Use the basis from (c) to write the solution sets to each of the following: Ax = 0 Ay= |1 Az= Solution. Let ₁ be the first column of A, 2 the second, and a3 the third column of A. (a) Since σ ₁ and 2 are not multiples of one another, they are linearly independent, but ã₁ + a2 = ã3 and so a basis for ran(A) is {ā₁, ā2} and so dim(ran(A)) = 2. (b) The rank-nullity theorem gives 3 = dim(ran(A)) + dim(ker(A)) and since dim(ran(A)) = 2, dim(ker(A)) = 1. (c) Since dim(ker(A)) = 1, we just need one nonzero element of the kernel. Since a1a2a3 = 0, so a basis for ker(A) is (1, 1, −1). = = α1 + α2 − α3 = 0
Linear Algebra: A Modern Introduction
4th Edition
ISBN:9781285463247
Author:David Poole
Publisher:David Poole
Chapter4: Eigenvalues And Eigenvectors
Section: Chapter Questions
Problem 9RQ
Related questions
Question
please explain part d because i am confused by the second and third part of part d.
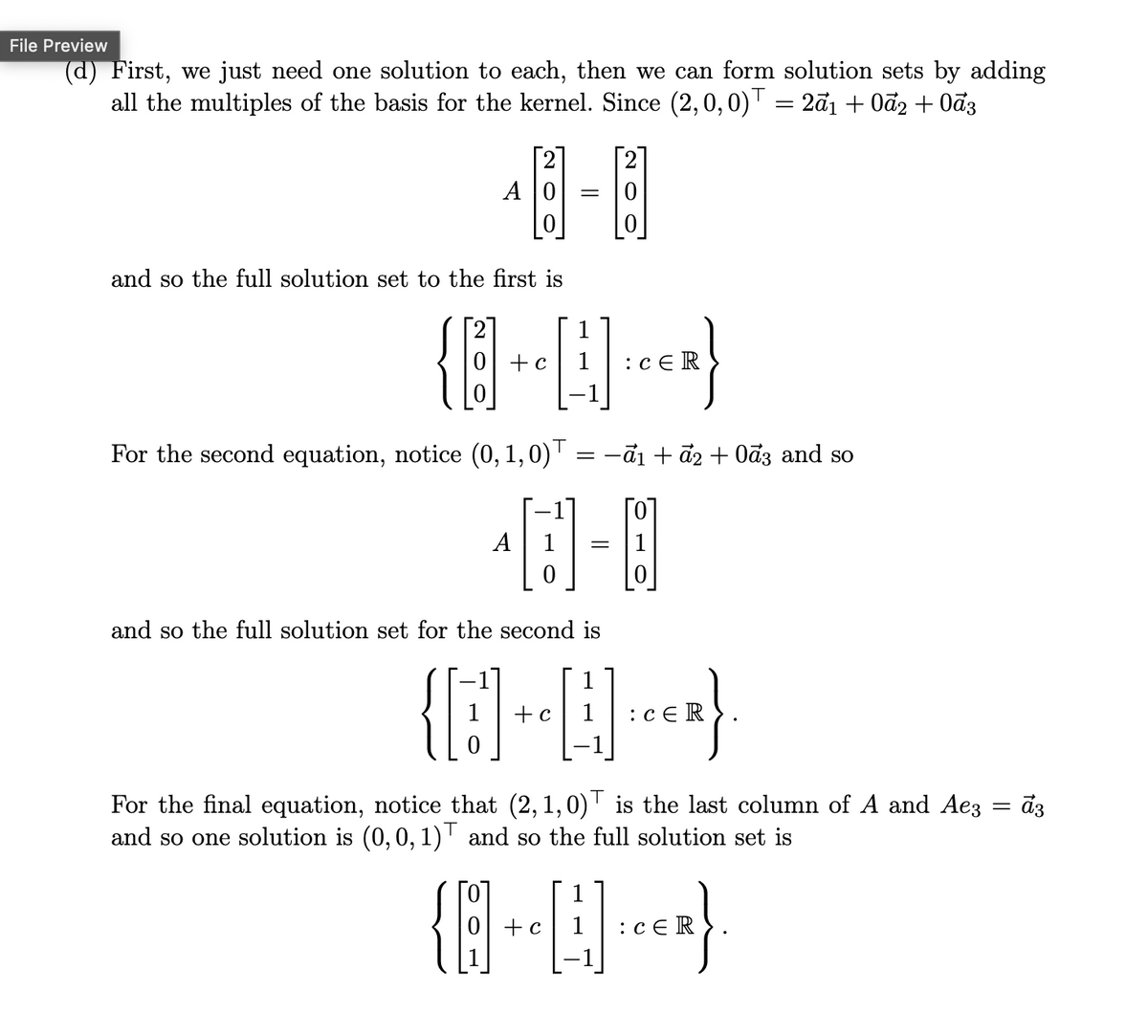
Transcribed Image Text:File Preview
(d) First, we just need one solution to each, then we can form solution sets by adding
all the multiples of the basis for the kernel. Since (2,0,0)¯ = 2ā1 +0ã2 + 0ã3
A0 =
0
and so the full solution set to the first is
1
+c 1
: CER
For the second equation, notice (0, 1,0)¯ = −ã1 + ã2 + 0ã3 and so
A
=
0-8
R}.
and so the full solution set for the second is
+ c
: CER
For the final equation, notice that (2,1,0) is the last column of A and Aе3
and so one solution is (0,0,1) and so the full solution set is
+c 1
: CER
=
ძვ
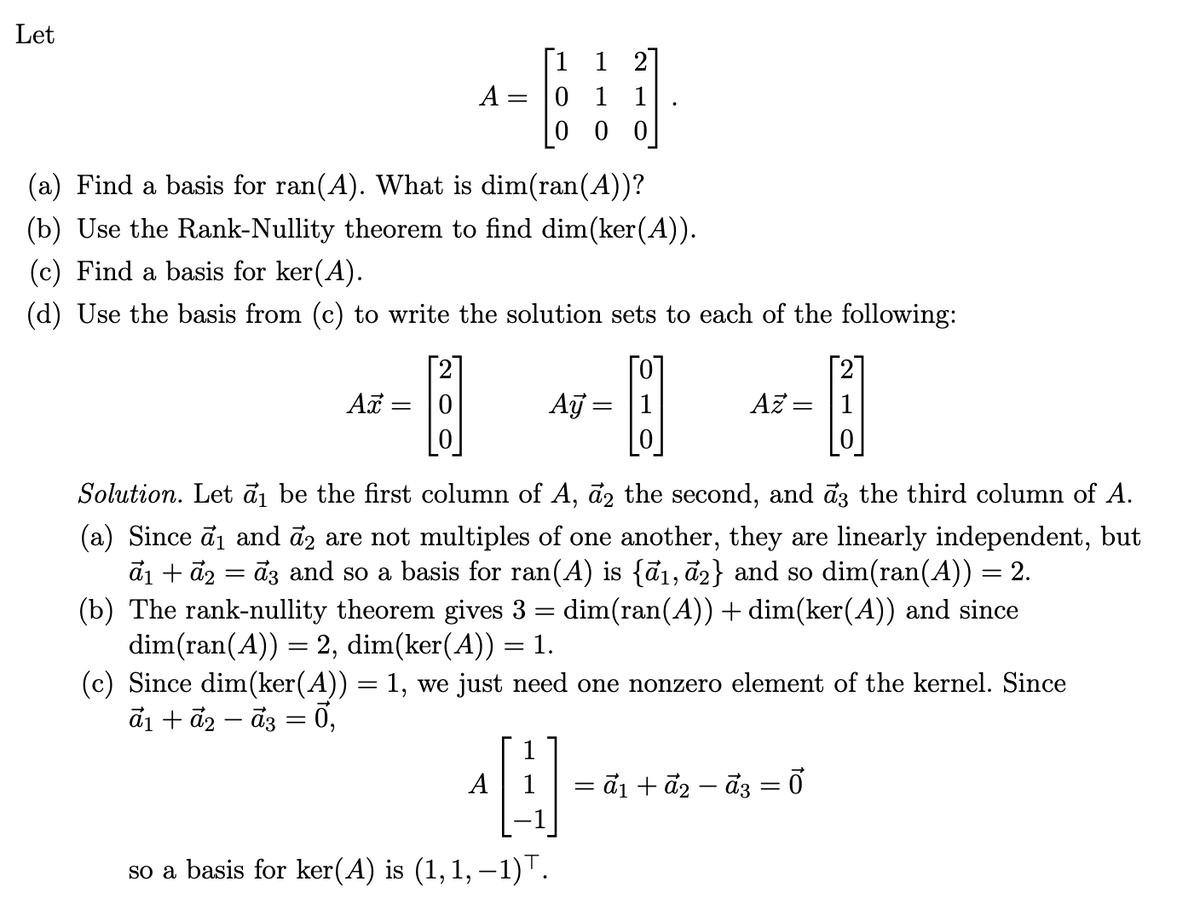
Transcribed Image Text:Let
A:
=
1 1 2
011
0
0 |
(a) Find a basis for ran(A). What is dim(ran(A))?
(b) Use the Rank-Nullity theorem to find dim (ker (A)).
(c) Find a basis for ker(A).
(d) Use the basis from (c) to write the solution sets to each of the following:
Ax = 0
Ay= |1
Az=
Solution. Let ₁ be the first column of A, 2 the second, and a3 the third column of A.
(a) Since σ ₁ and 2 are not multiples of one another, they are linearly independent, but
ã₁ + a2 = ã3 and so a basis for ran(A) is {ā₁, ā2} and so dim(ran(A)) = 2.
(b) The rank-nullity theorem gives 3 = dim(ran(A)) + dim(ker(A)) and since
dim(ran(A)) = 2, dim(ker(A)) = 1.
(c) Since dim(ker(A)) = 1, we just need one nonzero element of the kernel. Since
a1a2a3 = 0,
so a basis for ker(A) is (1, 1, −1).
=
= α1 + α2 − α3 = 0
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 2 steps with 4 images

Recommended textbooks for you

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:
9781305658004
Author:
Ron Larson
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:
9781305658004
Author:
Ron Larson
Publisher:
Cengage Learning