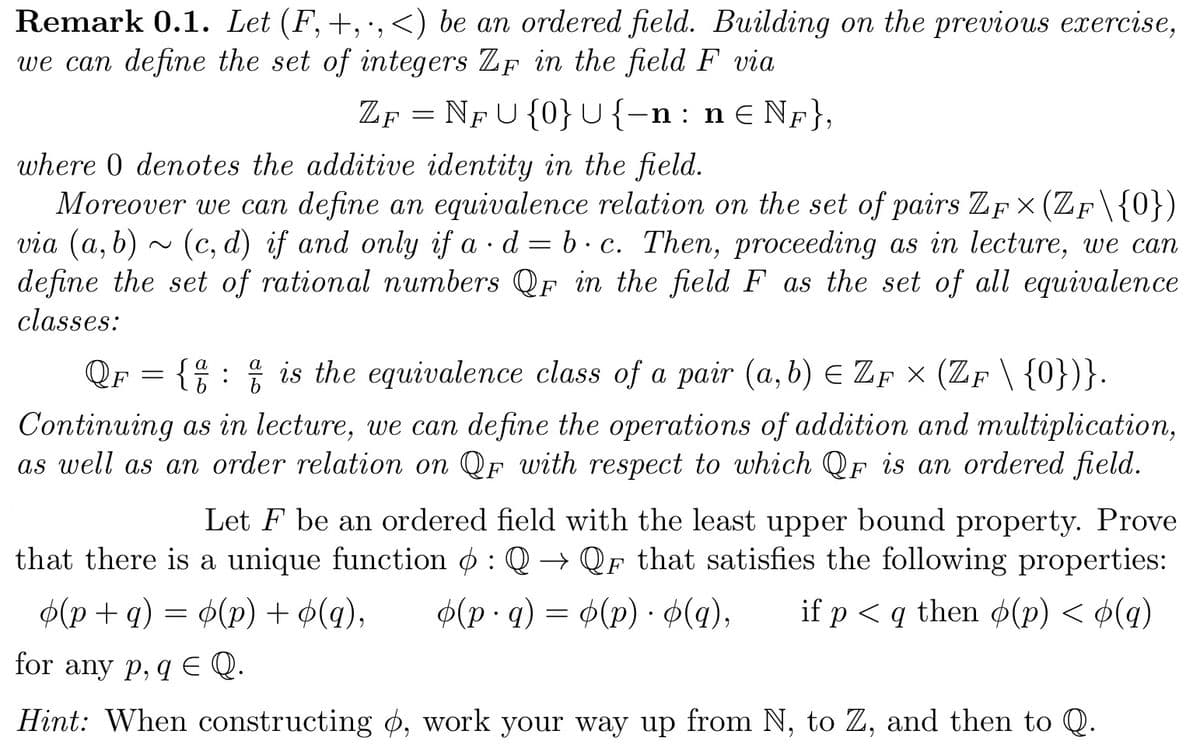
Remark 0.1. Let (F,+,·,<) be an ordered field. Building on the previous exercise,we can define the set of integers ZF in the field F viaZp = NF U{0}U{-n : n e NF},where 0 denotes the additive identity in the field.Moreover we can define an equivalence relation on the set of pairs ZFx (ZF\{0})via (a, b)define the set of rational numbers QF in the field F as the set of all equivalence~ (c, d) if and only if a · d = b. c. Then, proceeding as in lecture, we canclasses:QF ={ : ; is the equivalence class of a pair (a, b) E Zp X (ZF\ {0})}.Continuing as in lecture, we can define the operations of addition and multiplication,as well as an order relation on QF with respect to which QF is an ordered field.Let F be an ordered field with the least upper bound property. Provethat there is a unique function o : Q→ QF that satisfies the following properties:Ф(р + 9) — Ф(р) + ф(q),$(p · q) = ¢(p) · ø(g),if p < q then o(p) < ¢(q)for any p, q E Q.Hint: When constructing ø, work your way up from N, to Z, and then to Q.

An ordered field F with least upper bound property To prove that exist a unique function s.t. (i)…

Let F be an ordered field with the least upper bound property. Prove that there is a unique function o : Q → QF that satisfies the following properties: o(p q) = 0(p) · ở(q), $(p +q) = ¢(p) + ¢(q), if p < q then ø(p) < ¢(q) %3D for any p, q E Q. Hint: When constructing o, work your way up from N, to Z, and then to Q.

Let F be an ordered field with the least upper bound property. Prove that there is a unique function o : Q → QF that satisfies the following properties: o(p q) = 0(p) · ở(q), $(p +q) = ¢(p) + ¢(q), if p < q then ø(p) < ¢(q) %3D for any p, q E Q. Hint: When constructing o, work your way up from N, to Z, and then to Q.

Elements Of Modern Algebra

8th Edition

ISBN:9781285463230

Author:Gilbert, Linda, Jimmie

Publisher:Gilbert, Linda, Jimmie

Chapter7: Real And Complex Numbers

Section7.1: The Field Of Real Numbers

Problem 22E: Prove that if F is an ordered field with F+ as its set of positive elements, then F+nen+, where e...

See similar textbooks

Similar questions

Examples 5 and 6 of Section 5.1 showed that P(U) is a commutative ring with unity. In Exercises 4 and 5, let U={a,b}. Is P(U) a field? If not, find all nonzero elements that do not have multiplicative inverses. [Type here][Type here]
Suppose S is a subset of an field F that contains at least two elements and satisfies both of the following conditions: xS and yS imply xyS, and xS and y0S imply xy1S. Prove that S is a field. This S is called a subfield of F. [Type here][Type here]
If a0 in a field F, prove that for every bF the equation ax=b has a unique solution x in F. [Type here][Type here]
Prove that if R and S are fields, then the direct sum RS is not a field. [Type here][Type here]
Prove that if F is an ordered field with F+ as its set of positive elements, then F+nen+, where e denotes the multiplicative identity in F. (Hint: See Theorem 5.34 and its proof.) Theorem 5.34: Well-Ordered D+ If D is an ordered integral domain in which the set D+ of positive elements is well-ordered, then e is the least element of D+ and D+=nen+.
[Type here] Examples 5 and 6 of Section 5.1 showed that is a commutative ring with unity. In Exercises 4 and 5, let . 4. Is an integral domain? If not, find all zero divisors in . [Type here]
[Type here] 23. Let be a Boolean ring with unity. Prove that every element ofexceptandis a zero divisor. [Type here]
Exercises 10. Prove Theorem 5.4:A subset of the ring is a subring of if and only if these conditions are satisfied: is nonempty. and imply that and are in .
Prove that if R is a field, then R has no nontrivial ideals.
Let R be a commutative ring with unity whose only ideals are {0} and R Prove that R is a field.(Hint: See Exercise 30.)
Let be a field. Prove that if is a zero of then is a zero of
Prove that any field that contains an intergral domain D must contain a subfield isomorphic to the quotient field Q of D.