< Yn < Zn for all n > N; Encreasing for all n>N. that {yn} is a positive solution of equation ( {Zn} € So. Then z, is positive, zn 2 Yn, and Yn = Zn - Pnyo(n) 2 (1– p)zn, n>N> no
< Yn < Zn for all n > N; Encreasing for all n>N. that {yn} is a positive solution of equation ( {Zn} € So. Then z, is positive, zn 2 Yn, and Yn = Zn - Pnyo(n) 2 (1– p)zn, n>N> no
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN:9781133382119
Author:Swokowski
Publisher:Swokowski
Chapter5: Inverse, Exponential, And Logarithmic Functions
Section: Chapter Questions
Problem 43RE
Related questions
Question
Show me the steps of determine blue and all information is here step by step

Transcribed Image Text:Lemma 4. Let {yn} be a positive solution of equation (1.1) with the corresponding
sequence {z„} e So for n > N. Then:
(i) (1– p)zn < yn < Zn for all n > N;
(ii) {zn¢n} is increasing for all n > N.
Proof. Assume that {yn} is a positive solution of equation (1.1) with the corres-
ponding sequence {zn} E So. Then z, is positive, Zn 2 yn, and
Yn = Zn – Pnyo(n) 2 (1– p)zn, n>N> no,
so (i) is proved.
It easy to see that z, E So implies
lim b,(Az,)“ = 0;
n00
otherwise we would eventually have Az, > 0 contradicting z, E So. Similarly,
lim a„A(b,(Azn)“) = 0.
A summation of equation (1.1) then yields
9szs+1
Zn+1 LIs.
s=n
s=n
s=n
Summing once more, we obtain
as
t=s
s=n
or
Azn 2-Zn+1Qn.
Hence,
A(zn&n)
= PnAzn + Zn+1Ao, > Zn+1(A0n – OnQn) =0
since {0,} is a solution of the difference equation (Ao, – Qnºn) = 0. Therefore,
{z,9n} is increasing and this completes the proof of the lemma.
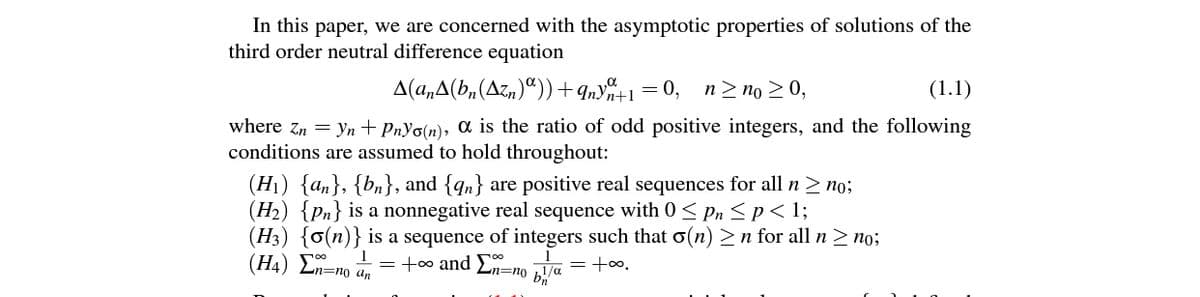
Transcribed Image Text:In this paper, we are concerned with the asymptotic properties of solutions of the
third order neutral difference equation
A(a,A(b,(Azn)“)) +9ny%+1=0,
n> no > 0,
(1.1)
where zn = yn+PnYo(n), ɑ is the ratio of odd positive integers, and the following
conditions are assumed to hold throughout:
(H1) {an}, {bn}, and {qn} are positive real sequences for all n > no;
(H2) {Pn} is a nonnegative real sequence with 0< Pn <p< 1;
(H3) {o(n)} is a sequence of integers such that o(n) > n for all n > no;
(H4) Ln=no an
= +00 and E=no Va =+∞.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 2 steps with 3 images

Recommended textbooks for you

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage