Homework Help is Here – Start Your Trial Now!
Math
Advanced Math
sin z et f(z) = 23(z – 3i)² sin (a) Find all the singularities of the function. Are they isolated? (b) singularity in case of a pole. Classify all the isolated singularity. Indicate the order of

sin z et f(z) = 23(z – 3i)² sin (a) Find all the singularities of the function. Are they isolated? (b) singularity in case of a pole. Classify all the isolated singularity. Indicate the order of

Linear Algebra: A Modern Introduction
Linear Algebra: A Modern Introduction
4th Edition
ISBN:9781285463247
Author:David Poole
Publisher:David Poole
Chapter7: Distance And Approximation
Section7.2: Norms And Distance Functions
Problem 41EQ
See similar textbooks
icon
Related questions
Question

[Complex Variables] How do you solve the first question? Thanks

Laurent* Series We know that a function that is analytic in the disc |z –- zol < R may be expanded there in a power series and that, conversely, if a function has a power series valid in the disc |z – zol < R, it is analytic in this disc. But what about a function f that is analytic only in the punctured disc 0 < |z – zol < R or, even worse, analytic only in the annulus 0<r < ]z – zol< R? We shall show here that something almost as good as a power series can be given to represent f(z), r < |z – zol < R. Namely, we shall show that f(2) = f1(2) + f2(2), r < |z – zol < R, * Pierre Alphonse Laurent, 1813-1854. 142 Chapter 2 Basic Properties of Analytic Functions where f, is analytic on the disc |z – zol < R and f2 is analytic on the region r< Iz – zol, including at o. f, has a power series in the variable z – z, which is valid for |z – zol < R, while f, has a power series in the variable (z – zo)-1 which is valid for r < |z – zol (see Exercise 23, Section 4). Consequently, f(z) = E a(z – zo)* + E ba(z – 20)-*, k=0 k=1 or f(2) = E a(z – zo)*, a-k = br, k = 1, 2, ..., r < |z – zol < R. k=-00
Transcribed Image Text:Laurent* Series We know that a function that is analytic in the disc |z –- zol < R may be expanded there in a power series and that, conversely, if a function has a power series valid in the disc |z – zol < R, it is analytic in this disc. But what about a function f that is analytic only in the punctured disc 0 < |z – zol < R or, even worse, analytic only in the annulus 0<r < ]z – zol< R? We shall show here that something almost as good as a power series can be given to represent f(z), r < |z – zol < R. Namely, we shall show that f(2) = f1(2) + f2(2), r < |z – zol < R, * Pierre Alphonse Laurent, 1813-1854. 142 Chapter 2 Basic Properties of Analytic Functions where f, is analytic on the disc |z – zol < R and f2 is analytic on the region r< Iz – zol, including at o. f, has a power series in the variable z – z, which is valid for |z – zol < R, while f, has a power series in the variable (z – zo)-1 which is valid for r < |z – zol (see Exercise 23, Section 4). Consequently, f(z) = E a(z – zo)* + E ba(z – 20)-*, k=0 k=1 or f(2) = E a(z – zo)*, a-k = br, k = 1, 2, ..., r < |z – zol < R. k=-00
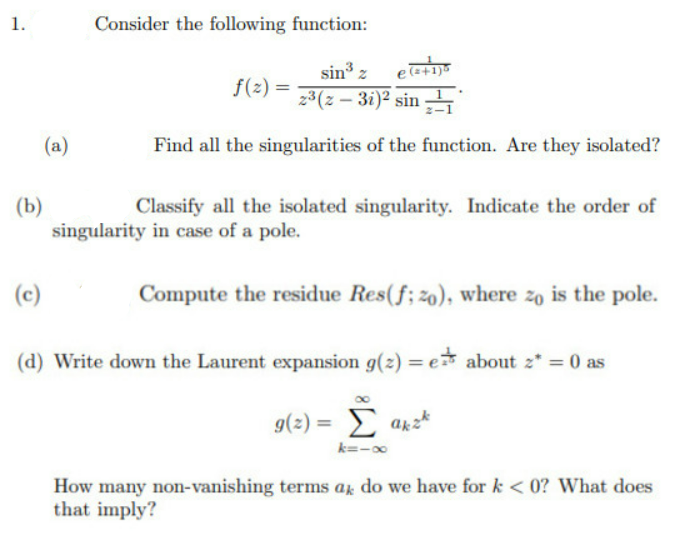
1. Consider the following function: sin3 23(z- 3i)2 sin f(z) = (a) Find all the singularities of the function. Are they isolated? (b) singularity in case of a pole. Classify all the isolated singularity. Indicate the order of (c) Compute the residue Res(f; zo), where zo is the pole. (d) Write down the Laurent expansion g(z) = e about z* = 0 as g(2) = arz* k=-0 How many non-vanishing terms a do we have for k < 0? What does that imply?
Transcribed Image Text:1. Consider the following function: sin3 23(z- 3i)2 sin f(z) = (a) Find all the singularities of the function. Are they isolated? (b) singularity in case of a pole. Classify all the isolated singularity. Indicate the order of (c) Compute the residue Res(f; zo), where zo is the pole. (d) Write down the Laurent expansion g(z) = e about z* = 0 as g(2) = arz* k=-0 How many non-vanishing terms a do we have for k < 0? What does that imply?
Expert Solution
steps

Step by step

Solved in 2 steps with 1 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Recommended textbooks for you
Linear Algebra: A Modern Introduction
Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage
SEE MORE TEXTBOOKS