Explain how the Limit Comparison Test works. THEOREM 10.15 Limit Comparison TestLet Ea, and Eb, be series with positive terms and letlimL.1. If 0 < L < ∞ (that is, L is a finite positive number), then Ea, and Eb,either both converge or both diverge.2. If L = 0 and Eb, converges, then Ea, converges.3. If L = ∞ and Eb, diverges, then Ea, diverges.

Let ∑ak and ∑bk be two positive term series, and limk→∞akbk=L. 1. If 0<L<∞(i.e. L is a finite…

Answered: THEOREM 10.15 Limit Comparison Test Let…

Algebra & Trigonometry with Analytic Geometry

13th Edition

ISBN:9781133382119

Author:Swokowski

Publisher:Swokowski

Chapter10: Sequences, Series, And Probability

Section10.2: Arithmetic Sequences

Problem 67E

See similar textbooks

Related questions

Question

Explain how the Limit Comparison Test works.

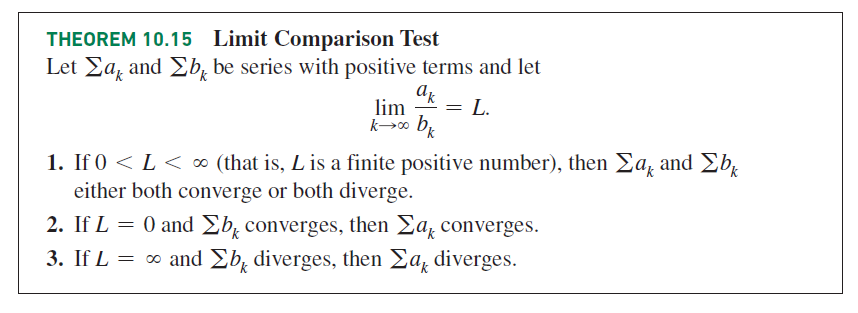
THEOREM 10.15 Limit Comparison Test
Let Ea, and Eb, be series with positive terms and let
lim
L.
1. If 0 < L < ∞ (that is, L is a finite positive number), then Ea, and Eb,
either both converge or both diverge.
2. If L = 0 and Eb, converges, then Ea, converges.
3. If L = ∞ and Eb, diverges, then Ea, diverges.

Expert Solution

Step 1

Let $\sum a_{k}$ and $\sum b_{k}$ be two positive term series, and $\lim_{k \to \infty} \frac{a_{k}}{b_{k}} = L$ .

1. If $0 < L < \infty$ (i.e. L is a finite positive number) Then $\sum a_{k}$ and $\sum b_{k}$ both converge or diverge together.

2. If $L = 0$ and $\sum b_{k}$ converges, then $\sum a_{k}$ also converges.

3. $L = \infty$ and $\sum b_{k}$ diverges, then $\sum a_{k}$ also diverges.

Step 2

1. Since $a_{k} > 0$ , $b_{k} > 0$

$\lim_{k \to \infty} \frac{a_{k}}{b_{k}} = L > 0$

But $\lim_{k \to \infty} \frac{a_{k}}{b_{k}} = L \neq 0$

Now $\lim_{k \to \infty} \frac{a_{k}}{b_{k}} = L$

$\Rightarrow$ given $\in > 0$ , $\exists$ a positive integer m such that

$|\frac{a_{k}}{b_{k}} - L| < \in \forall k > m$

$\Rightarrow L - \in < \frac{a_{k}}{b_{k}} < L + \in \forall k > m$

Choose $\in > 0$ such that $L - \in > 0$ .

Let $L - \in = H, L + \in = K$ where $H, K > 0$

$∴ H b_{k} < a_{k} < K b_{k} \forall k > m . . . (*)$

Case-1: When $\sum a_{k}$ is convergent

From $(*)$ $H b_{k} < a_{k} \forall k > m$

$\Rightarrow b_{k} < \frac{1}{H} a_{k} \forall k > m (∴ H > 0)$

Since $\sum a_{k}$ is convergent, $\sum b_{k}$ is also convergent.

Case.II: When $\sum a_{k}$ is divergent.

From $(*)$ , $a_{k} < K b_{k} \forall k > m$

$\Rightarrow \frac{a_{k}}{K} < b_{k} \forall k > m (∵ K > 0)$

Since $\sum a_{k}$ is divergent, $\sum b_{k}$ is also divergent.

Case.III: When $\sum b_{k}$ is convergent.

From (*), $a_{k} < K b_{k} \forall k > m$

Since $\sum b_{k}$ is convergent, $\sum a_{k}$ is also convergent.

Case:IV: When $\sum b_{k}$ is divergent.

From (*), $H b_{k} < a_{k} \forall k > m$

Since $\sum b_{k}$ is divergent, $\sum a_{k}$ is also divergent.

Hence $\sum a_{k}$ and $\sum b_{k}$ converge or diverge together.

Step by step

Solved in 4 steps

Knowledge Booster

$Inequality$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.

Recommended textbooks for you

Algebra & Trigonometry with Analytic Geometry

Algebra

ISBN:

9781133382119

Author:

Swokowski

Publisher:

Cengage

Algebra & Trigonometry with Analytic Geometry

Algebra

ISBN:

9781133382119

Author:

Swokowski

Publisher:

Cengage

SEE MORE TEXTBOOKS