Theorem 8 Let p ≤ (0, ³). Then the equilibrium point ã of Eq.(8) is locally asymptotically stable. Proof. From (15), we have Note that Thus |91| + |92|+... ·|9m| = Р Hence, we get from p > 0, 191 +92 +9m| 3 (2p+1-√4p+ 2p 2p+1-√4p+1 2p 4p+3-3√4p + 1 2p = 3p < 1, 3p < 0. 4p- 1) (√4p+1 C(√4P+1-1 So, we obtain 0 < p < . Therefore, the proof of Theorem 8 is completed. <1, / -2)< 0.
Theorem 8 Let p ≤ (0, ³). Then the equilibrium point ã of Eq.(8) is locally asymptotically stable. Proof. From (15), we have Note that Thus |91| + |92|+... ·|9m| = Р Hence, we get from p > 0, 191 +92 +9m| 3 (2p+1-√4p+ 2p 2p+1-√4p+1 2p 4p+3-3√4p + 1 2p = 3p < 1, 3p < 0. 4p- 1) (√4p+1 C(√4P+1-1 So, we obtain 0 < p < . Therefore, the proof of Theorem 8 is completed. <1, / -2)< 0.
Linear Algebra: A Modern Introduction
4th Edition
ISBN:9781285463247
Author:David Poole
Publisher:David Poole
Chapter4: Eigenvalues And Eigenvectors
Section4.6: Applications And The Perron-frobenius Theorem
Problem 70EQ
Related questions
Question
Show me the determine pruple and inf is here
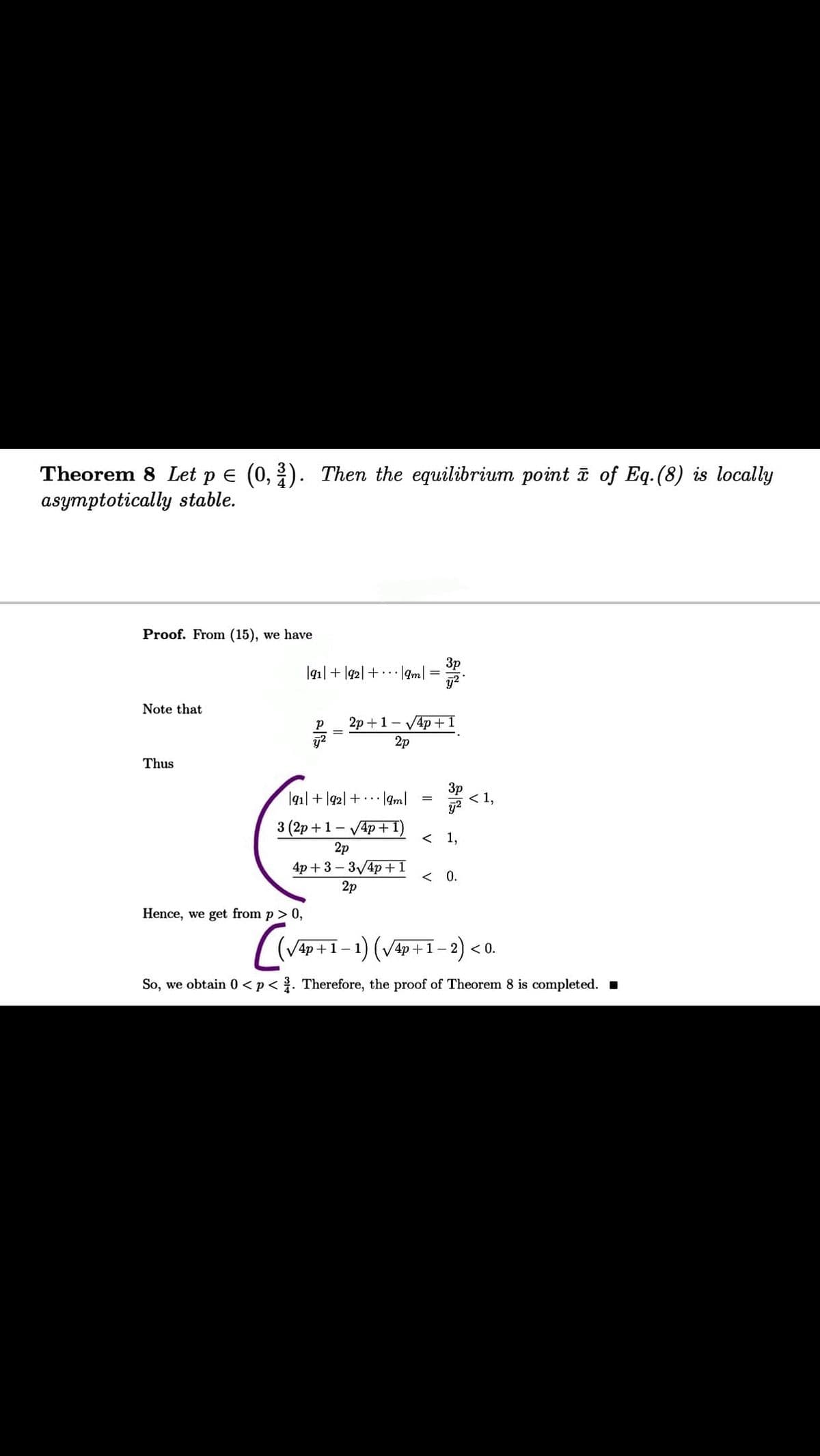
Transcribed Image Text:Theorem 8 Let p e (0, ). Then the equilibrium point i of Eq. (8) is locally
asymptotically stable.
Proof. From (15), we have
lg1|+ |92|+ am|
Note that
2p +1- V4p +1
2p
Thus
3p
< 1,
y2
l91|+ 192| +
3 (2p +1- V4p+ 1)
< 1,
2p
4p + 3 — З/4p+1
2p
< 0.
Hence, we get from p > 0,
4p + 1 – 1) (V4p +1– 2) < 0.
So, we obtain 0 <p< . Therefore, the proof of Theorem 8 is completed. I

Transcribed Image Text:Yn+1 = 1+P-m
4 Stability of Eq.(8)
In this here, we study the stability of Eq.(8). Firstly we handle the linearized
equation of Eq.(8) about its unique positive equilibrium point. Let I be some
interval of real numbers and let
f : Im+1
be a continuously differentiable function such that f is defined by
Yn
f (yn, Yn-1,* , Yn-m) = 1+p
Уп-т
Therefore we have
af
qo =
Əyn
%3D
q1 = q2
...= qm-1= 0,
af
Əyn-m
2p
Im
-
Hence we obtain the linearized equation of Eq.(8) about its unique positive
equilibrium point ỹ as follow:
2p
2n-m = 0.
(15)
Zn+1
Zn +
Therefore, the characteristic equation of Eq.(8) is
2p
m+1
(16)
|
Hence we obtain the linearized equation of Eq.(8) about its unique positive
equilibrium point j as follow:
2p
Zn+1
Zn +
Zn-m
= 0.
(15)
Motivated by the above studies, we study the dynamics of following higher
order difference equation
In+1
In
A+B-
(2)
%3D
"n-m
where A, B are positive real numbers and the initial conditions are positive
numbers. Additionally, we investigate the boundedness, periodicity, oscillation
behaviours, global asymptotically stability and rate of convergence of related
higher order difference equations.
Firstly, we take the change of the variables for Eq.(2) as follows yn =
From this, we obtain the following difference equation
Yn
.2
(8)
where p = . From now on, we handle the difference equation (8). The unique
positive equilibrium point of Eq.(8) is
1+ VI+ 4p
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 2 steps

Recommended textbooks for you

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning