Theorem. Let < be a preorder on a set X. Define the relation =, where x = y holds if and only if x < y and y < x. Then = is an equivalence relation on X.
Theorem. Let < be a preorder on a set X. Define the relation =, where x = y holds if and only if x < y and y < x. Then = is an equivalence relation on X.
Elements Of Modern Algebra
8th Edition
ISBN:9781285463230
Author:Gilbert, Linda, Jimmie
Publisher:Gilbert, Linda, Jimmie
Chapter1: Fundamentals
Section1.7: Relations
Problem 14E: In each of the following parts, a relation is defined on the set of all human beings. Determine...
Related questions
Question
Please Help ASAP!!!
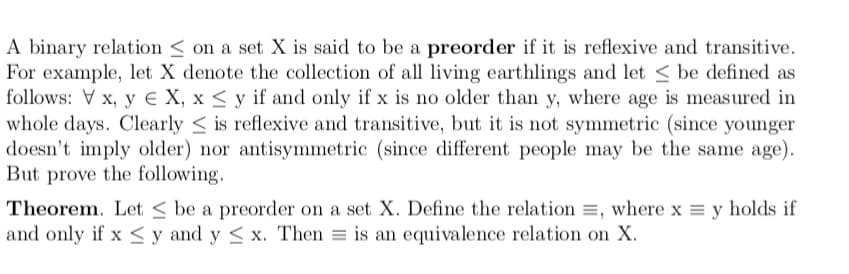
Transcribed Image Text:A binary relation < on a set X is said to be a preorder if it is reflexive and transitive.
For example, let X denote the collection of all living earthlings and let < be defined as
follows: V x, y € X, x < y if and only if x is no older than y, where age is measured in
whole days. Clearly < is reflexive and transitive, but it is not symmetric (since younger
doesn't imply older) nor antisymmetric (since different people may be the same age).
But prove the following.
Theorem. Let < be a preorder on a set X. Define the relation =, where x = y holds if
and only if x <y and y < x. Then = is an equivalence relation on X.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,