Use the equivalent optimization problem above to find the point on the ellipse that is the furtherest away from the origin. • The point: • The distance: Finally, find the point on the ellipse that is the closest from the origin. • The point: • The distance:
Use the equivalent optimization problem above to find the point on the ellipse that is the furtherest away from the origin. • The point: • The distance: Finally, find the point on the ellipse that is the closest from the origin. • The point: • The distance:
Elementary Geometry For College Students, 7e
7th Edition
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Alexander, Daniel C.; Koeberlein, Geralyn M.
Chapter10: Analytic Geometry
Section10.1: The Rectangular Coordinate System
Problem 44E: By definition, a hyperbola is the locus of points whose positive difference of distances from two...
Related questions
Question
please answer the 4 parts in part 2.
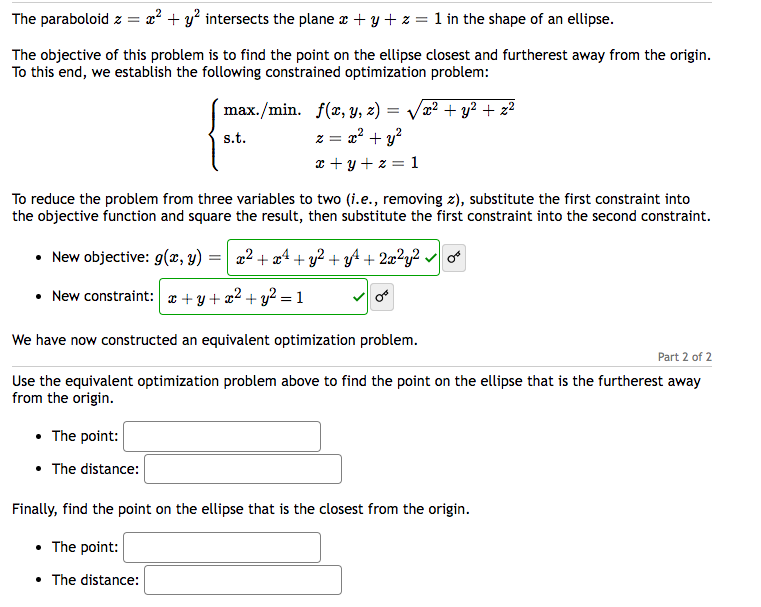
Transcribed Image Text:=x² + y² intersects the plane x + y + z = 1 in the shape of an ellipse.
The paraboloid z =
The objective of this problem is to find the point on the ellipse closest and furtherest away from the origin.
To this end, we establish the following constrained optimization problem:
max./min.
s.t.
f(x, y, z) = √√√x² + y² + x²
z = x² + y²
x+y+z=1
To reduce the problem from three variables to two (i.e., removing z), substitute the first constraint into
the objective function and square the result, then substitute the first constraint into the second constraint.
• The point:
• The distance:
• New objective: g(x, y)
=
New constraint: + y + x² + y² = 1
We have now constructed an equivalent optimization problem.
x² + x² + y² + y² + 2x²y²✓
Part 2 of 2
Use the equivalent optimization problem above to find the point on the ellipse that is the furtherest away
from the origin.
Finally, find the point on the ellipse that is the closest from the origin.
• The point:
• The distance:
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 2 steps

Recommended textbooks for you

Elementary Geometry For College Students, 7e
Geometry
ISBN:
9781337614085
Author:
Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:
Cengage,

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

College Algebra
Algebra
ISBN:
9781305115545
Author:
James Stewart, Lothar Redlin, Saleem Watson
Publisher:
Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:
9781337614085
Author:
Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:
Cengage,

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

College Algebra
Algebra
ISBN:
9781305115545
Author:
James Stewart, Lothar Redlin, Saleem Watson
Publisher:
Cengage Learning

Algebra for College Students
Algebra
ISBN:
9781285195780
Author:
Jerome E. Kaufmann, Karen L. Schwitters
Publisher:
Cengage Learning
