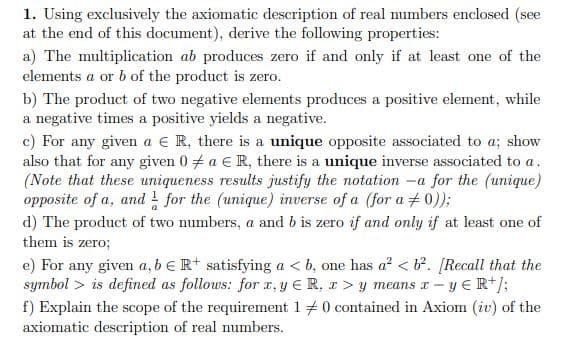
i need Q1 answer other pic is just for reference (end of the document) 1. Using exclusively the axiomatic description of real numbers enclosed (seeat the end of this document), derive the following properties:a) The multiplication ab produces zero if and only if at least one of theelements a or b of the product is zero.b) The product of two negative elements produces a positive element, whilea negative times a positive yields a negative.c) For any given a e R, there is a unique opposite associated to a; showalso that for any given 0 a € R, there is a unique inverse associated to a.(Note that these uniqueness results justify the notationopposite of a, and ! for the (unique) inverse of a (for a + 0));-a for the (unique)d) The product of two numbers, a and b is zero if and only if at least one ofthem is zero;e) For any given a, bERt satisfying a < b, one has a' < b². [Recall that thesymbol > is defined as follows: for I, y ER, r > y means r – y ER+);f) Explain the scope of the requirement 1 +0 contained in Axiom (iv) of theaxiomatic description of real numbers. We define R to be the set in which two operations are defined,+ : Rx R + R,andx : Rx R + R.and such that (R, +, x) satisfies the following axioms:Field arioms:(i) the commutative property of the addition and multiplication operations:a +b = b+ a; ab = ba;(ü) the associative property of the addition and multiplication operations:(a + b) +c = a+ (b+c); (ab) c = a (be);(ii) the distributive property of products over sums(a + 6) c = ac + be, a (b+ c) = ab+ ac;(iv) the existence of an additive identity 0 and a distinct multiplicative iden-tity, that is, 1 + 0, satisfying a +0 = a for all a E R and a x1 = a for alla € R;(v) for each element a € R, the existence of an additive inverse b, also calledopposite, i.e an element b such that a + b = 0;(vi) for each element a + 0 there exists a multiplicative inverse b, ie. anelement b such that a x b = 1;Ordering arioms:There exists a subset of R, which we denote R* which does not contain 0,which satisfies the following properties:(vii) R* is closed under the operations of sum and multiplication, that is:a, be R+ = a+ b, ab € Rt;(viii) for any a ER, either a eR+, or -a e R*, or a = 0(ir) for a, b, e e R, a -be Rt and 6-CER* = a -cERt.Besides the field axioms ((i)-(vi) and the ordering axioms (vii)-(iz), whichare also satisfied by the set of rational mumbers Q, the real numbers alsosatisfy(x) the completeness ariom.

As per our guidelines I am solving only 1st three for others kindly resubmit the question mentioning…

1. Using exclusively the axiomatic description of real numbers enclosed (see at the end of this document), derive the following properties: a) The multiplication ab produces zero if and only if at least one of the elements a or b of the product is zero. b) The product of two negative elements produces a positive element, while a negative times a positive yields a negative. c) For any given a e R, there is a unique opposite associated to a; show also that for any given 0 # a € R, there is a unique inverse associated to a. (Note that these uniqueness results justify the notation opposite of a, and ! for the (unique) inverse of a (for a +0)); -a for the (unique) d) The product of two numbers, a and b is zero if and only if at least one of them is zero; e) For any given a, beRt satisfying a < b, one has a? < b?. [Recall that the symbol > is defined as follows: for I, y ER, r > y means r – y ER+); f) Explain the scope of the requirement 1 +0 contained in Axiom (iv) of the axiomatic description of real numbers.

1. Using exclusively the axiomatic description of real numbers enclosed (see at the end of this document), derive the following properties: a) The multiplication ab produces zero if and only if at least one of the elements a or b of the product is zero. b) The product of two negative elements produces a positive element, while a negative times a positive yields a negative. c) For any given a e R, there is a unique opposite associated to a; show also that for any given 0 # a € R, there is a unique inverse associated to a. (Note that these uniqueness results justify the notation opposite of a, and ! for the (unique) inverse of a (for a +0)); -a for the (unique) d) The product of two numbers, a and b is zero if and only if at least one of them is zero; e) For any given a, beRt satisfying a < b, one has a? < b?. [Recall that the symbol > is defined as follows: for I, y ER, r > y means r – y ER+); f) Explain the scope of the requirement 1 +0 contained in Axiom (iv) of the axiomatic description of real numbers.

Elements Of Modern Algebra

8th Edition

ISBN:9781285463230

Author:Gilbert, Linda, Jimmie

Publisher:Gilbert, Linda, Jimmie

Chapter3: Groups

Section3.1: Definition Of A Group

Problem 34E: 34. Let be the set of eight elements with identity element and noncommutative multiplication...

See similar textbooks