Answered: A symmetric random walk in two… | bartleby

Algebra & Trigonometry with Analytic Geometry

Algebra & Trigonometry with Analytic Geometry

13th Edition

ISBN:9781133382119

Author:Swokowski

Publisher:Swokowski

Chapter10: Sequences, Series, And Probability

Section10.8: Probability

Problem 31E

See similar textbooks

Related questions

Question

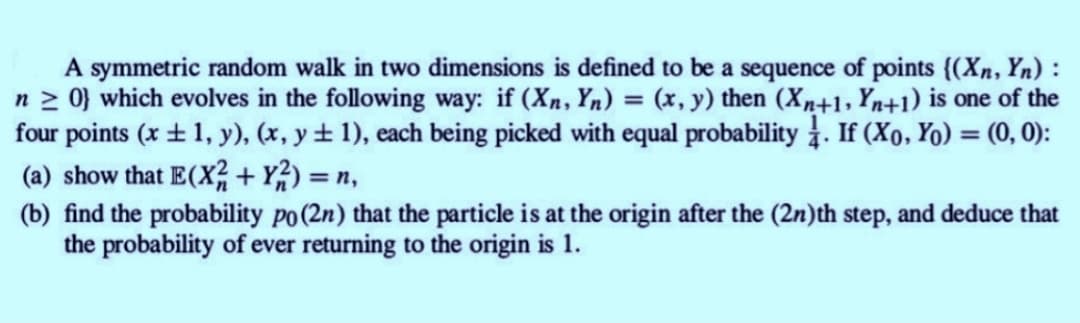
A symmetric random walk in two dimensions is defined to be a sequence of points {(Xn, Yn):
n≥ 0} which evolves in the following way: if (Xn, Yn) = (x, y) then (Xn+1, Yn+1) is one of the
four points (x ±1, y), (x, y ± 1), each being picked with equal probability. If (Xo, Yo) = (0,0):
(a) show that E(X2 + Y2²) = n,
(b) find the probability po (2n) that the particle is at the origin after the (2n)th step, and deduce that
the probability of ever returning to the origin is 1.

Expert Solution

Step by step

Solved in 4 steps with 4 images

SEE SOLUTION Check out a sample Q&A here

Blurred answer

Similar questions

Let X1,X2,... be a sequence of identically distributed random variables with E|X1|<∞ and let Yn = n−1max1≤i≤n|Xi|. Show that limnE(Yn) = 0
Let X1, X2, ... , Xn be a random sample from N(μ, σ2). Find the Moment Generating Function of X̅. If n = 16 and σ = 2, compute P(-1 ≤ X̅ - μ ≤ 1).
Let X1, X2, X3, . . . be a sequence of independent Poisson distributed random variables with parameter 1. For n ≥ 1 let Sn = X1 + · · · + Xn. (a) Show that GXi(s) = es−1.(b) Deduce from part (a) that GSn(s) = ens−n.
Show that the random process X(t) =cos(2π fot + θ) Where θ is an random variable uniformly distributed in the range {0, π/2, π, π/3} is a wide sense stationary process .
LetX1,X2,...,Xn be a sequence of independent and identically distributed random variables having the Exponential(λ) distribution,λ >0, fXi(x) ={λe−λx, x >0 0, otherwise Define the random variable Y=X1+X2+···+Xn. Find E(Y),Var(Y)and the moment generating function ofY.
Suppose X1, X2, ... , Xn is a random sample and Xi = {1, with probability p 0, with probability 1-p} for every i = 1, 2, ... , n. Find the Moment Generating Function of ∑i=1n Xi . What is the distribution of ∑i=1n Xi ?
The starting time of a class is uniformly distributed between 10:00 and 10:05. If a student arrives early and has to wait t minutes for the class to start, then the student incurs a penalty of At, which accounts for the waste in the student's time. On the other hand, if the student arrives t minutes after the class has started, then the student incurs a penalty of A2t, which accounts for the information the student has missed. If the student arrives at x minutes after 10:00, what is the expected penalty incurred by the student? What value of x minimizes the expected penalty?
Let {Xt : t > 0} be a compound Poisson process of parameter λ. Suppose that each of the summands of this process is constant equal to k ∈ IN . Find the distribution of Xt.
Show that for the simple random walk starting at zero,P(first visit to S^(2n) occurs at t = 2k) = P(S_(2k) = 0) · P(S_(2n−2k) = 0)for 0 ≤ k ≤ n.
Let T1 and T2 be random times for a company to complete two steps in a certain process. Say T1 and T2 are discrete random variables that are measured in days, and they have the joint PMF that is uniform over the space: t1,t2 ∈N,1≤ t1 ≤ 10, 2≤ t2 ≤ 6, t1+2t2 ≤ 14. Compute P (T1 + T2 ≥ 10).
Let {N_1(t)} and {N_2(t)} be two independent Poisson processes with rates λ1=1 and λ2=2, respectively. Find the probability that the second arrival in N_1(t) occurs before the third arrival in N_2(t). Round answer to 4 decimals.
What is the simple random walk on integers? Does it always return to the origin if p = ½ ? if p ≠ ½?

SEE MORE QUESTIONS

Recommended textbooks for you

Algebra & Trigonometry with Analytic Geometry

Algebra & Trigonometry with Analytic Geometry

Algebra

ISBN:

9781133382119

Author:

Swokowski

Publisher:

Cengage

Linear Algebra: A Modern Introduction

Linear Algebra: A Modern Introduction

Algebra

ISBN:

9781285463247

Author:

David Poole

Publisher:

Cengage Learning

Algebra & Trigonometry with Analytic Geometry

Algebra & Trigonometry with Analytic Geometry

Algebra

ISBN:

9781133382119

Author:

Swokowski

Publisher:

Cengage

Linear Algebra: A Modern Introduction

Linear Algebra: A Modern Introduction

Algebra

ISBN:

9781285463247

Author:

David Poole

Publisher:

Cengage Learning

SEE MORE TEXTBOOKS