Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN:9781133382119
Author:Swokowski
Publisher:Swokowski
Chapter2: Equations And Inequalities
Section2.6: Inequalities
Problem 78E
Related questions
Question
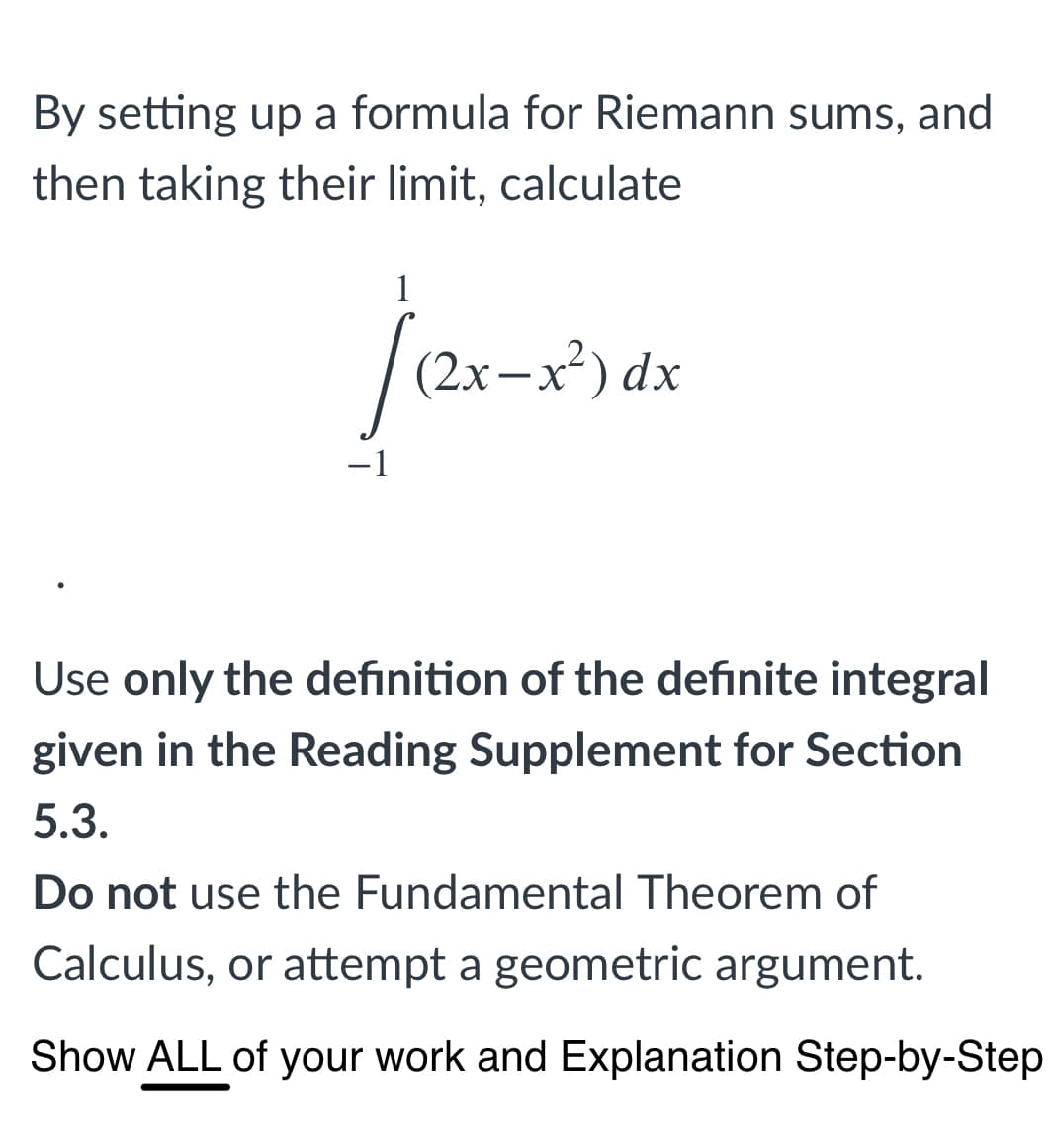
Transcribed Image Text:By setting up a formula for Riemann sums, and
then taking their limit, calculate
1
|(2x-x³).
-1
Use only the definition of the definite integral
given in the Reading Supplement for Section
5.3.
Do not use the Fundamental Theorem of
Calculus, or attempt a geometric argument.
Show ALL of your work and Explanation Step-by-Step
![In this section we consider the limit of general Riemann sums as the norm of the partitions
of a closed interval [a, b] approaches zero. This limiting process leads us to the definition
of the definite integral of a function over a closed interval [a, b].
5.3 The Definite Integral
319
Definition of the Definite Integral
When the definite integral exișts, we say that the Riemann sums of ƒ on [a, b] converge
to the definite integral J = ["f(x) dx and that f is integrable over [a, b].
In the cases where the subintervals all have equal width Ax = (b – a)/n, the Rie-
The definition of the definite integral is based on the fact that for some functions, as the
norm of the partitions of [ a, b] approaches zero, the values of the corresponding Riemann
mann sums have the form
= E f(c) Ax, =
Ax = Ar = (b – a)/n for all k
k-1
k-1
where c is chosen in the kth subinterval. If the definite integral exists, then these Riemann
sums converge to the definite integral of f over [a, b], so
ь — а
sums approach a limiting value J. We introduce the symbol ɛ as a small positive number
that specifies how close to J the Riemann sum must be, and the symbol 8 as a second
small positive number that specifies how small the norm of a partition must be in order for
convergence to happen. We now define this limit precisely.
For equal-width subintervals,
||P| 0 is the same as n- 0.
J =
f(x)dx = lim
k-1
If we pick the point c to be the right endpoint of the kth subinterval, so that
C = a + kAx =a + k(b – a)/n, then the formula for the definite integral becomes
DEFINITION Let f(x) be a function defined on a closed interval [a, b]. We
say that a number J is the definite integral of f over [a, b] and that J is the limit
of the Riemann sums E- f(c) Ax, if the following condition is satisfied:
Given any number e > 0 there is a corresponding number 8 > 0 such
that for every partition P = {xg, X1. . .. ,x,} of [ a, b] with || P || < 8 and any
choice of c in [X-1. X], we have
A Formula for the Riemann Sum with Equal-Width Subintervals
f(x) dx = lim
b
+ k
(1)
flo) Ax, -
-1
Equation (1) gives one explicit formula that can be used to compute definite integrals. As
long as the definite integral exists, the Riemann sums corresponding to other choices of
partitions and locations of points c̟ will have the same limit as n–∞, provided that the
norm of the partition approaches zero.
The value of the definite integral of a function over any particular interval depends on
the function, not on the letter we choose to represent its independent variable. If we decide
to use t or u instead of x, we simply write the integral as
The definition involves a limiting process in which the norm of the partition goes to zero.
We have many choices for a partition P with norm going to zero, and many choices of
points c for each partition. The definite integral exists when we always get the same limit
J, no matter what choices are made. When the limit exists we write
lim
Efla) Ax,
f(1) dt
f(u) du
instead of
or
I we say that the definite integral exists. The limit of any Riemann sum is always taken
as the norm of the partitions approaches zero and the number of subintervals goes to infin-
ity, and furthermore the same limit J must be obtained no matter what choices
and
No matter how we write the integral, it is still the same number, the limit of the Riemann
sums as the norm of the partition approaches zero. Since it does not matter what letter we
use, the variable of integration is called a dummy variable. In the three integrals given
above, the dummy variables are 1, u, and x.
make for
the points c-
Leibniz introduced a notation for the definite integral that captures its construction as
a limit of Riemann sums. He envisioned the finite sums E- f(c) Ax, becoming an infi-
nite sum of function values f(x) multiplied by "infinitesimal" subinterval widths dx. The
sum symbol E is replaced in the limit by the integral symbol /, whose origin is in the
letter "S" (for sum). The function values f(c) are replaced by a continuous selection of
function values f(x). The subinterval widths Ax, become the differential dx. It is as if we
are summing all products of the form f(x)· dx as x goes from a to b. While this notation
captures the process of constructing an integral, it is Riemann's definition that gives a pre-
cise meaning to the definite integral.
If the definite integral exists, then instead of writing J we write
f(x) dx.
We read this as "the integral from a to b of f of x dee x" or sometimes as "the integral from
a to b of f of x with respect to x." The component parts in the integral symbol also have
names:
The function f(x) is the integrand.
Upper limit of integration
b'
x is the variable of integration.
Integral sign
f(x) dx
Ja
When you find the value
of the integral, you have
evaluated the integral.
Lower limit of integration
Integral of f from a to b](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F9779159c-e093-4274-82bf-229d4c839a5e%2Fefedd538-c1fe-4f9d-993c-3816ad4ffd60%2Fvw2tmb_processed.jpeg&w=3840&q=75)
Transcribed Image Text:In this section we consider the limit of general Riemann sums as the norm of the partitions
of a closed interval [a, b] approaches zero. This limiting process leads us to the definition
of the definite integral of a function over a closed interval [a, b].
5.3 The Definite Integral
319
Definition of the Definite Integral
When the definite integral exișts, we say that the Riemann sums of ƒ on [a, b] converge
to the definite integral J = ["f(x) dx and that f is integrable over [a, b].
In the cases where the subintervals all have equal width Ax = (b – a)/n, the Rie-
The definition of the definite integral is based on the fact that for some functions, as the
norm of the partitions of [ a, b] approaches zero, the values of the corresponding Riemann
mann sums have the form
= E f(c) Ax, =
Ax = Ar = (b – a)/n for all k
k-1
k-1
where c is chosen in the kth subinterval. If the definite integral exists, then these Riemann
sums converge to the definite integral of f over [a, b], so
ь — а
sums approach a limiting value J. We introduce the symbol ɛ as a small positive number
that specifies how close to J the Riemann sum must be, and the symbol 8 as a second
small positive number that specifies how small the norm of a partition must be in order for
convergence to happen. We now define this limit precisely.
For equal-width subintervals,
||P| 0 is the same as n- 0.
J =
f(x)dx = lim
k-1
If we pick the point c to be the right endpoint of the kth subinterval, so that
C = a + kAx =a + k(b – a)/n, then the formula for the definite integral becomes
DEFINITION Let f(x) be a function defined on a closed interval [a, b]. We
say that a number J is the definite integral of f over [a, b] and that J is the limit
of the Riemann sums E- f(c) Ax, if the following condition is satisfied:
Given any number e > 0 there is a corresponding number 8 > 0 such
that for every partition P = {xg, X1. . .. ,x,} of [ a, b] with || P || < 8 and any
choice of c in [X-1. X], we have
A Formula for the Riemann Sum with Equal-Width Subintervals
f(x) dx = lim
b
+ k
(1)
flo) Ax, -
-1
Equation (1) gives one explicit formula that can be used to compute definite integrals. As
long as the definite integral exists, the Riemann sums corresponding to other choices of
partitions and locations of points c̟ will have the same limit as n–∞, provided that the
norm of the partition approaches zero.
The value of the definite integral of a function over any particular interval depends on
the function, not on the letter we choose to represent its independent variable. If we decide
to use t or u instead of x, we simply write the integral as
The definition involves a limiting process in which the norm of the partition goes to zero.
We have many choices for a partition P with norm going to zero, and many choices of
points c for each partition. The definite integral exists when we always get the same limit
J, no matter what choices are made. When the limit exists we write
lim
Efla) Ax,
f(1) dt
f(u) du
instead of
or
I we say that the definite integral exists. The limit of any Riemann sum is always taken
as the norm of the partitions approaches zero and the number of subintervals goes to infin-
ity, and furthermore the same limit J must be obtained no matter what choices
and
No matter how we write the integral, it is still the same number, the limit of the Riemann
sums as the norm of the partition approaches zero. Since it does not matter what letter we
use, the variable of integration is called a dummy variable. In the three integrals given
above, the dummy variables are 1, u, and x.
make for
the points c-
Leibniz introduced a notation for the definite integral that captures its construction as
a limit of Riemann sums. He envisioned the finite sums E- f(c) Ax, becoming an infi-
nite sum of function values f(x) multiplied by "infinitesimal" subinterval widths dx. The
sum symbol E is replaced in the limit by the integral symbol /, whose origin is in the
letter "S" (for sum). The function values f(c) are replaced by a continuous selection of
function values f(x). The subinterval widths Ax, become the differential dx. It is as if we
are summing all products of the form f(x)· dx as x goes from a to b. While this notation
captures the process of constructing an integral, it is Riemann's definition that gives a pre-
cise meaning to the definite integral.
If the definite integral exists, then instead of writing J we write
f(x) dx.
We read this as "the integral from a to b of f of x dee x" or sometimes as "the integral from
a to b of f of x with respect to x." The component parts in the integral symbol also have
names:
The function f(x) is the integrand.
Upper limit of integration
b'
x is the variable of integration.
Integral sign
f(x) dx
Ja
When you find the value
of the integral, you have
evaluated the integral.
Lower limit of integration
Integral of f from a to b
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage


Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage


Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,