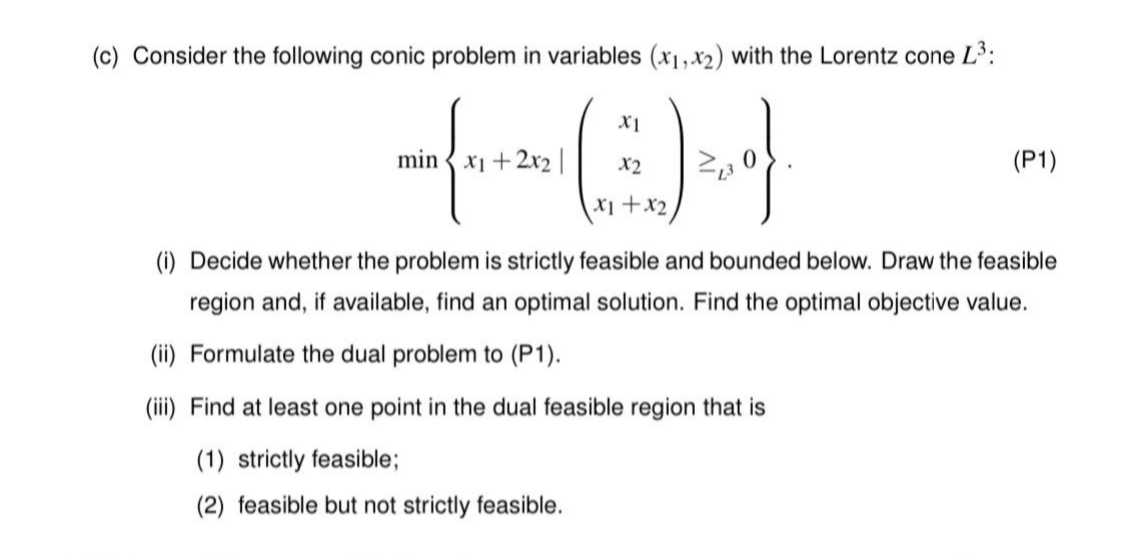
Solve c part optimization problem (c) Consider the following conic problem in variables (x1,x2) with the Lorentz cone L3:X1min { x1 +2x2 |X2(P1)X1+x2(i) Decide whether the problem is strictly feasible and bounded below. Draw the feasibleregion and, if available, find an optimal solution. Find the optimal objective value.(ii) Formulate the dual problem to (P1).(iii) Find at least one point in the dual feasible region that is(1) strictly feasible;(2) feasible but not strictly feasible. 1.(a) Let K CR" be a proper cone. Prove that the relation a - bEK is reflexive, antisymmetricand transitive on R".(b) Consider the following two sets of symmetric matrices:C = {A € S" | x"Ax > 0 Vx €R", x> 0}%3DandkP = {A € S" | A = £ ®(z®)", z® eR", ¿0 > 0, i = 1,..,k} .%3Di=1Prove:(i) C and P are cones.(ii) Show that C C P*, where P* is the dual cone to P.(c) Consider the following conic problem in variables (x1,x2) with the Lorentz cone L3:X1min { x1 +2x2 |X2(P1)x1+x2(i) Decide whether the problem is strictly feasible and bounded below. Draw the feasibleregion and, if available, find an optimal solution. Find the optimal objective value.(ii) Formulate the dual problem to (P1).(iii) Find at least one point in the dual feasible region that is(1) strictly feasible;(2) feasible but not strictly feasible.LM Advanced Management MathematicsTurn over

(c) Consider the following conic problem in variables (x1,x2) with the Lorentz cone L³: min { x1 +2x2 | X2 (P1) X1 +x2 (i) Decide whether the problem is strictly feasible and bounded below. Draw the feasible region and, if available, find an optimal solution. Find the optimal objective value. (ii) Formulate the dual problem to (P1). (iii) Find at least one point in the dual feasible region that is (1) strictly feasible; (2) feasible but not strictly feasible.

(c) Consider the following conic problem in variables (x1,x2) with the Lorentz cone L³: min { x1 +2x2 | X2 (P1) X1 +x2 (i) Decide whether the problem is strictly feasible and bounded below. Draw the feasible region and, if available, find an optimal solution. Find the optimal objective value. (ii) Formulate the dual problem to (P1). (iii) Find at least one point in the dual feasible region that is (1) strictly feasible; (2) feasible but not strictly feasible.

Algebra for College Students

10th Edition

ISBN:9781285195780

Author:Jerome E. Kaufmann, Karen L. Schwitters

Publisher:Jerome E. Kaufmann, Karen L. Schwitters

Chapter12: Algebra Of Matrices

Section12.CR: Review Problem Set

Problem 35CR: Maximize the function fx,y=7x+5y in the region determined by the constraints of Problem 34.

See similar textbooks

Similar questions

Find the maximum value of the function z = 8x-2y with the restrictions as shown by the feasible region above
Suppose that in a certain year, the Phoenix/Zweig Advisors Zweig Total Return fund (ZTR) was expected to yield 4%, and the Madison Asset Management Madison Strategic Sector Premium fund (MSP) was expected to yield 9%. You would like to invest a total of up to $60,000 and earn at least $4500 in interest. Draw the feasible region that shows how much money you can invest in each fund (based on the given yields). (Place ZTR on the x-axis and MSP on the y-axis.). Find the corner points of the region. (Order your answers from smallest to largest x, then from smallest to largest y. If an answer does not exist, enter DNE.) (x, y)= ( ) (x, y)= ( ) (x, y)= ( )
Consider the following problem. Minimize Z=3X1+2X2+4X3 Subject to 2X1+X2+3X3=60 3X1+3X2+5X3≥120 and X1, X2, X3≥0 Using the two-phase method, work through the simplex method to solve the problem
1. Consider the parametric linear program Maximize (4 + 4λ)x1+(4 + 8λ)x2+(8 + 4λ)x3Subject to x1+3x2-x3 ≤ 10-x1+x2+2x3 ≤ 152x1+x2+x3 ≤ 20xi ≥ 0, i = 1, 2, 3. An optimal simplex tableau of the LP for λ = 0 is given below.x1 x2 x3 x4 x5 x6 RHS BV0 17/5 0 1 3/5 -1/5 15 x40 3/5 1 0 2/51/5 10 x31 1/5 0 0 -1/52/5 5 x10 8/5 0 0 12/516/5 100 ⇐ OBJ (a) Compute the characteristic interval for the optimal basis and identify the slope of f(λ), the optimal objective function value as a function of λ, for λ belongs to this interval. (b) Compute the new optimal solution if the RHS vector is replaced by (10, 20, 30)T and λ = 0
This extreme value problem has a solution with both a maximum value and a minimum value. Use Lagrange multipliers to find the extreme values of the function subject to the given constraint. f(x, y) = 8x + 2y, x2 + y2 = 17
Use the method of Lagrange Multipliers to find the maximum and minimumvaluesof x2+y2 subject to the constraint x4+ y4=2
Use two-phase simplex Method to solve the following LP Minimize z= 6 x1 + 3 x2 Subject to x1+x2 ≥ 1 2x1- x2 ≥ 1 3x2 ≤ 2 x1,x2 ≥ 0
Suppose that a temperature of a metal plate is given by T(x,y)=x2+2x+y2, for points (x,y) on the elliptic plate defined by 6x2+5y2≤60. Find the maximum and minimum temperatures on the plate. Use Lagrange Multipliers to determine the absolute extrema of f on the indicated constraint.
Solve max f( x,y , z) 3x2+y problem by means of Lagrange multipliers under the constraints of 4x-3y=9 and x2+z2=9. Explain what the values of Lagrange multipliers mean?
Use Lagrange multipliers to find the maximum and minimum values of f subject to thegiven constraint:f(x, y) = xyz Given x2 + y2 + z2 = 3.