Homework Help is Here – Start Your Trial Now!
Science
Physics
Consider a thin, spherical shell of radius R and total mass M as shown in the Figure below. Given that the surface area of a sphere is 4nR^2, the mass per unit area of the sphere is 4x R Rde a) Consider a thin ring on the surface of the spherical shell of width Rdo as shown in blue. Show that the mass of the ring dM is given by M sin ødø 2 dM = b) Show that the gravitational field due to the single ring at a point P distance r from the centre of the sphere is given by j = GM cos 0 sin ødø î 2s2 c) Using trigonometric identities (or deriving the geometry from first principles), show that this field can be re-written as -GM (s² +r² – R²) -ds î 4Rr²s²

Consider a thin, spherical shell of radius R and total mass M as shown in the Figure below. Given that the surface area of a sphere is 4nR^2, the mass per unit area of the sphere is 4x R Rde a) Consider a thin ring on the surface of the spherical shell of width Rdo as shown in blue. Show that the mass of the ring dM is given by M sin ødø 2 dM = b) Show that the gravitational field due to the single ring at a point P distance r from the centre of the sphere is given by j = GM cos 0 sin ødø î 2s2 c) Using trigonometric identities (or deriving the geometry from first principles), show that this field can be re-written as -GM (s² +r² – R²) -ds î 4Rr²s²

University Physics Volume 1
University Physics Volume 1
18th Edition
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:William Moebs, Samuel J. Ling, Jeff Sanny
Chapter13: Gravitation
Section: Chapter Questions
Problem 82CP: Following the technique used in Gravitation Near Earth’s Surface, find the value of g as a function...
See similar textbooks
icon
Related questions
Question
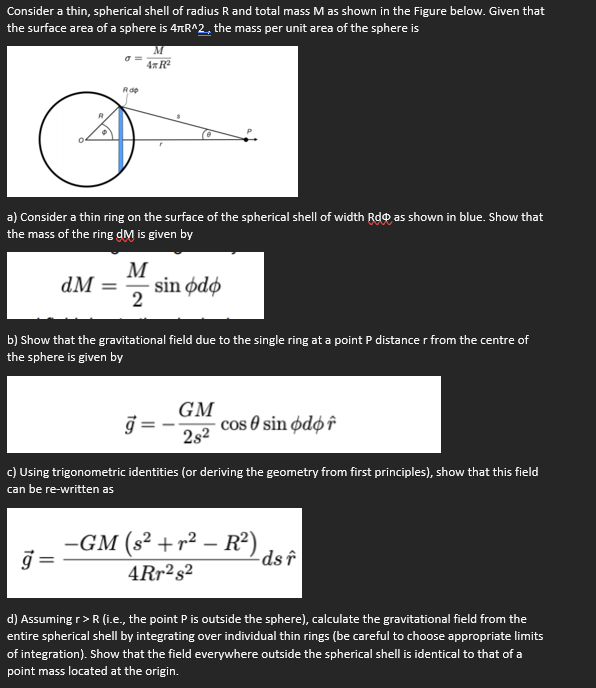
Consider a thin, spherical shell of radius R and total mass M as shown in the Figure below. Given that the surface area of a sphere is 4nR^2, the mass per unit area of the sphere is 4x R Rde a) Consider a thin ring on the surface of the spherical shell of width Rdo as shown in blue. Show that the mass of the ring dM is given by M sin ødø 2 dM = b) Show that the gravitational field due to the single ring at a point P distance r from the centre of the sphere is given by j = GM cos 0 sin ødø î 2s2 c) Using trigonometric identities (or deriving the geometry from first principles), show that this field can be re-written as -GM (s² +r² – R²) -ds î 4Rr²s² d) Assuming r>R (i.e., the point P is outside the sphere), calculate the gravitational field from the entire spherical shell by integrating over individual thin rings (be careful to choose appropriate limits of integration). Show that the field everywhere outside the spherical shell is identical to that of a point mass located at the origin.
Transcribed Image Text:Consider a thin, spherical shell of radius R and total mass M as shown in the Figure below. Given that the surface area of a sphere is 4nR^2, the mass per unit area of the sphere is 4x R Rde a) Consider a thin ring on the surface of the spherical shell of width Rdo as shown in blue. Show that the mass of the ring dM is given by M sin ødø 2 dM = b) Show that the gravitational field due to the single ring at a point P distance r from the centre of the sphere is given by j = GM cos 0 sin ødø î 2s2 c) Using trigonometric identities (or deriving the geometry from first principles), show that this field can be re-written as -GM (s² +r² – R²) -ds î 4Rr²s² d) Assuming r>R (i.e., the point P is outside the sphere), calculate the gravitational field from the entire spherical shell by integrating over individual thin rings (be careful to choose appropriate limits of integration). Show that the field everywhere outside the spherical shell is identical to that of a point mass located at the origin.
Expert Solution
steps

Step by step

Solved in 4 steps with 1 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Similar questions
  • SEE MORE QUESTIONS
Recommended textbooks for you
University Physics Volume 1
University Physics Volume 1
Physics
ISBN:
9781938168277
Author:
William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:
OpenStax - Rice University
Astronomy
Astronomy
Physics
ISBN:
9781938168284
Author:
Andrew Fraknoi; David Morrison; Sidney C. Wolff
Publisher:
OpenStax
The Solar System
The Solar System
Physics
ISBN:
9781337672252
Author:
The Solar System
Publisher:
Cengage
Foundations of Astronomy (MindTap Course List)
Foundations of Astronomy (MindTap Course List)
Physics
ISBN:
9781337399920
Author:
Michael A. Seeds, Dana Backman
Publisher:
Cengage Learning
Stars and Galaxies (MindTap Course List)
Stars and Galaxies (MindTap Course List)
Physics
ISBN:
9781337399944
Author:
Michael A. Seeds
Publisher:
Cengage Learning
SEE MORE TEXTBOOKS