Fill in the blanks in the following proof: Theorem 1. The set of prime numbers is infinite. Proof. Suppose not. Then there is a complete, finite, which con- tains all of the prime numbers. P1 = 2, P2 = 3, P3 = 5, P4 = 7, ..., Pn Consider the integer formed by taking the product of all of these primes and then adding 1. N = (piP2P3P4. .- Pn) +1 This number is . than any of the primes in our list, in particular it cannot be prime itself and must therefore be divisible by at least one of the primes (say p;). On the other hand, N is one greater than a multiple of p;, so p; cannot divide N. This is a since we have shown that N is both divisible by p; and not divisible by pj. Hence the supposition is and the theorem is true.
Fill in the blanks in the following proof: Theorem 1. The set of prime numbers is infinite. Proof. Suppose not. Then there is a complete, finite, which con- tains all of the prime numbers. P1 = 2, P2 = 3, P3 = 5, P4 = 7, ..., Pn Consider the integer formed by taking the product of all of these primes and then adding 1. N = (piP2P3P4. .- Pn) +1 This number is . than any of the primes in our list, in particular it cannot be prime itself and must therefore be divisible by at least one of the primes (say p;). On the other hand, N is one greater than a multiple of p;, so p; cannot divide N. This is a since we have shown that N is both divisible by p; and not divisible by pj. Hence the supposition is and the theorem is true.
Elements Of Modern Algebra
8th Edition
ISBN:9781285463230
Author:Gilbert, Linda, Jimmie
Publisher:Gilbert, Linda, Jimmie
Chapter2: The Integers
Section2.2: Mathematical Induction
Problem 1E: Prove that the statements in Exercises are true for every positive integer .
1.
Related questions
Topic Video
Question
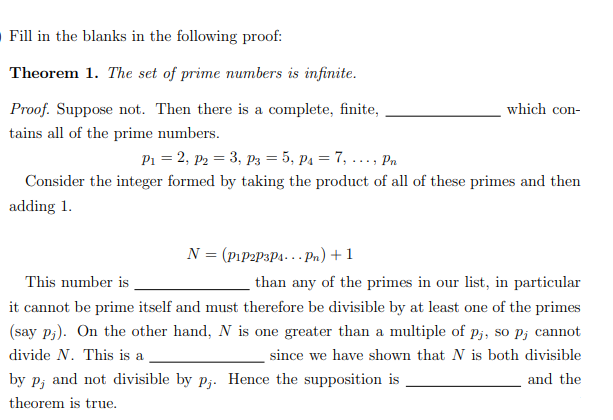
Transcribed Image Text:O Fill in the blanks in the following proof:
Theorem 1. The set of prime numbers is infinite.
Proof. Suppose not. Then there is a complete, finite,
which con-
tains all of the prime numbers.
P1 = 2, p2 = 3, P3 = 5, p1 = 7, ..., Pn
Consider the integer formed by taking the product of all of these primes and then
adding 1.
N = (PıP2P3P4... Pn) +1
This number is
than any of the primes in our list, in particular
it cannot be prime itself and must therefore be divisible by at least one of the primes
(say P;). On the other hand, N is one greater than a multiple of p;, so P; cannot
divide N. This is a
since we have shown that N is both divisible
by p; and not divisible by pj. Hence the supposition is
and the
theorem is true.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 2 steps

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Recommended textbooks for you

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,


College Algebra (MindTap Course List)
Algebra
ISBN:
9781305652231
Author:
R. David Gustafson, Jeff Hughes
Publisher:
Cengage Learning

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,


College Algebra (MindTap Course List)
Algebra
ISBN:
9781305652231
Author:
R. David Gustafson, Jeff Hughes
Publisher:
Cengage Learning