Theorem 13.Let {xn} be a solution of Eq.(1). Then the following statements are true: (i) N>0, the intial conditions Suppose b < d and for some XN-1+1, XN-k+1,•…•, XN-1, XN E ...) are valid, then for b+ e and ď + be, we have the inequality $(A+B+C+D)+ S Xn S (A+B+C+D)+• b (df–be) (b-e) (44) for all n N. (ii) N>0, the intial conditions Suppose b > d and for some XN-1+1,·…, XN–k+1, •……, XN–1, XN E 6. are valid, then for b + e and d # be, we have the inequality (A+B+C+D)+ s Xns (A+B+C+D)+ (f-be)' (45) for all n> N. Proof.First of all, if for some N>0, < XN S1 and b#e, we have bxN-k XN+1 = AXN+ BXN–k+CxN–1+Dxŋ-o+ dxN-k- exN-1 bxN-k
Theorem 13.Let {xn} be a solution of Eq.(1). Then the following statements are true: (i) N>0, the intial conditions Suppose b < d and for some XN-1+1, XN-k+1,•…•, XN-1, XN E ...) are valid, then for b+ e and ď + be, we have the inequality $(A+B+C+D)+ S Xn S (A+B+C+D)+• b (df–be) (b-e) (44) for all n N. (ii) N>0, the intial conditions Suppose b > d and for some XN-1+1,·…, XN–k+1, •……, XN–1, XN E 6. are valid, then for b + e and d # be, we have the inequality (A+B+C+D)+ s Xns (A+B+C+D)+ (f-be)' (45) for all n> N. Proof.First of all, if for some N>0, < XN S1 and b#e, we have bxN-k XN+1 = AXN+ BXN–k+CxN–1+Dxŋ-o+ dxN-k- exN-1 bxN-k
Linear Algebra: A Modern Introduction
4th Edition
ISBN:9781285463247
Author:David Poole
Publisher:David Poole
Chapter4: Eigenvalues And Eigenvectors
Section4.6: Applications And The Perron-frobenius Theorem
Problem 70EQ
Related questions
Question
show me the steps of determine blue and the inf is here. And that's it
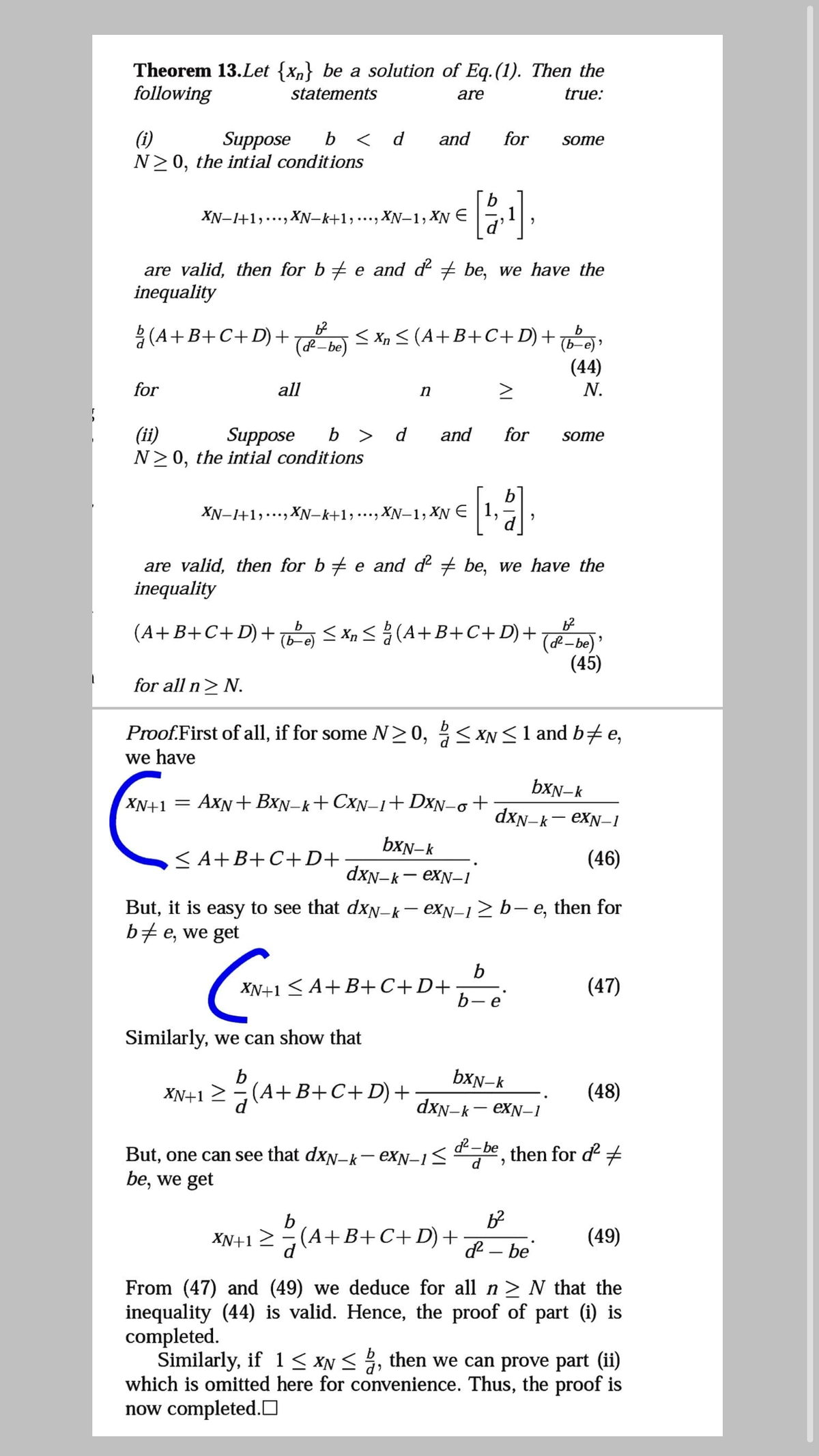
Transcribed Image Text:Theorem 13.Let {xn} be a solution of Eq.(1). Then the
following
statements
are
true:
(i)
N>0, the intial conditions
Suppose
b <
d
and
for
some
XN-1+1,..., XN-k+1, ..., XN-1, XN E
are valid, then for b + e and d + be, we have the
inequality
(A+B+C+D) +
b
(b-e)'
(44)
(df– be)
< Xn < (A+B+C+D)+
for
all
N.
(ii)
N>0, the intial conditions
Suppose
b >
d
and
for
some
XN-1+1,.. XN-k+1, ·…,XN-1, XN E |1,
are valid, then for b + e and d + be, we have the
inequality
(A+B+C+D)+ a s Xm s%(A+B+C+D)+ T
(f-be)
(45)
for all n> N.
Proof.First of all, if for some N>0, < XN <1 and b#e,
we have
bxN-k
XN+1
= AXN+ BXN-k+Cxn–1+DxN-o +
dxN-k- exN-1
bxN-k
<A+B+ С+D+
(46)
dxN-k- exN-1
But, it is easy to see that dxN-k- exn-12 b- e, then for
b+ e, we get
XN+1 <A+B+C+D+
(47)
b- e
Similarly, we can show that
b.
;(A+B+C+D)+
bxN-k
(48)
XN+1 2
dxN-k- exN-1
d² – be then for ď +
But, one can see that dxN-k- exN-1<
be, we get
d
XN+1 2(A
(A+B+C+D)+
d – be
(49)
From (47) and (49) we deduce for all n> N that the
inequality (44) is valid. Hence, the proof of part (i) is
completed.
Similarly, if 1 < XN <, then we can prove part (ii)
which is omitted here for convenience. Thus, the proof is
now completed.O
![The objective of this article is to investigate some
qualitative behavior of the solutions of the nonlinear
difference equation
bxn-k
Xn+1 = Axn+ Bx–k+Cxn-1+ Dxn-o+
dxn-k– exŋ-
n= 0,1,2,.....
(1)
where the coefficients A, B, C, D, b, d, e E (0,0), while
k, 1 and o are positive integers. The initial conditions
X_g,..., X_1,.., X_k, ….., X_1, X are arbitrary positive real
numbers such that k <1< 0. Note that the special cases
of Eq.(1) have been studied in [1] when B=C= D=0,
and k= 0,1= 1, b is replaced by – b and in [27] when
B=C= D=0, and k= 0, b is replaced by
[33] when B = C = D = 0, 1= 0 and in [32] when
A=C= D=0, 1=0, b is replaced by – b.
b and in
%3|](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F7ecaae78-467a-4f8b-9627-a81f9986c070%2F3c63a8ac-8d07-4e8c-a9e8-a1306e2acf0e%2F0shoyhc_processed.jpeg&w=3840&q=75)
Transcribed Image Text:The objective of this article is to investigate some
qualitative behavior of the solutions of the nonlinear
difference equation
bxn-k
Xn+1 = Axn+ Bx–k+Cxn-1+ Dxn-o+
dxn-k– exŋ-
n= 0,1,2,.....
(1)
where the coefficients A, B, C, D, b, d, e E (0,0), while
k, 1 and o are positive integers. The initial conditions
X_g,..., X_1,.., X_k, ….., X_1, X are arbitrary positive real
numbers such that k <1< 0. Note that the special cases
of Eq.(1) have been studied in [1] when B=C= D=0,
and k= 0,1= 1, b is replaced by – b and in [27] when
B=C= D=0, and k= 0, b is replaced by
[33] when B = C = D = 0, 1= 0 and in [32] when
A=C= D=0, 1=0, b is replaced by – b.
b and in
%3|
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 4 steps

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Recommended textbooks for you

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage