Theorem 5. Suppose that {xn} is the solution of the difference equation (1) and the initial values ,l = 0,1, 2, ....k. are arbitrary nonzero real numbers .Let x k+l = a_k+l where X-k+l = a-k+l 1 = 0,1, 2, ..k. Then ,by using the notations(IX), the solutions of the difference equation (1) are given by: (7) a-k+l A ) – n-1 a-k+l 9-2 1(7) An' i =1 II (4ª")"* where T 1,2, ...k +1 ,1= 0,1, 2, ..k and n > 2. Proof. We can the mathematical induction as in theorems(2.1) and (2.2) , to prove this theorem .
Theorem 5. Suppose that {xn} is the solution of the difference equation (1) and the initial values ,l = 0,1, 2, ....k. are arbitrary nonzero real numbers .Let x k+l = a_k+l where X-k+l = a-k+l 1 = 0,1, 2, ..k. Then ,by using the notations(IX), the solutions of the difference equation (1) are given by: (7) a-k+l A ) – n-1 a-k+l 9-2 1(7) An' i =1 II (4ª")"* where T 1,2, ...k +1 ,1= 0,1, 2, ..k and n > 2. Proof. We can the mathematical induction as in theorems(2.1) and (2.2) , to prove this theorem .
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
Show me the steps and proof for the determine blue and inf is here
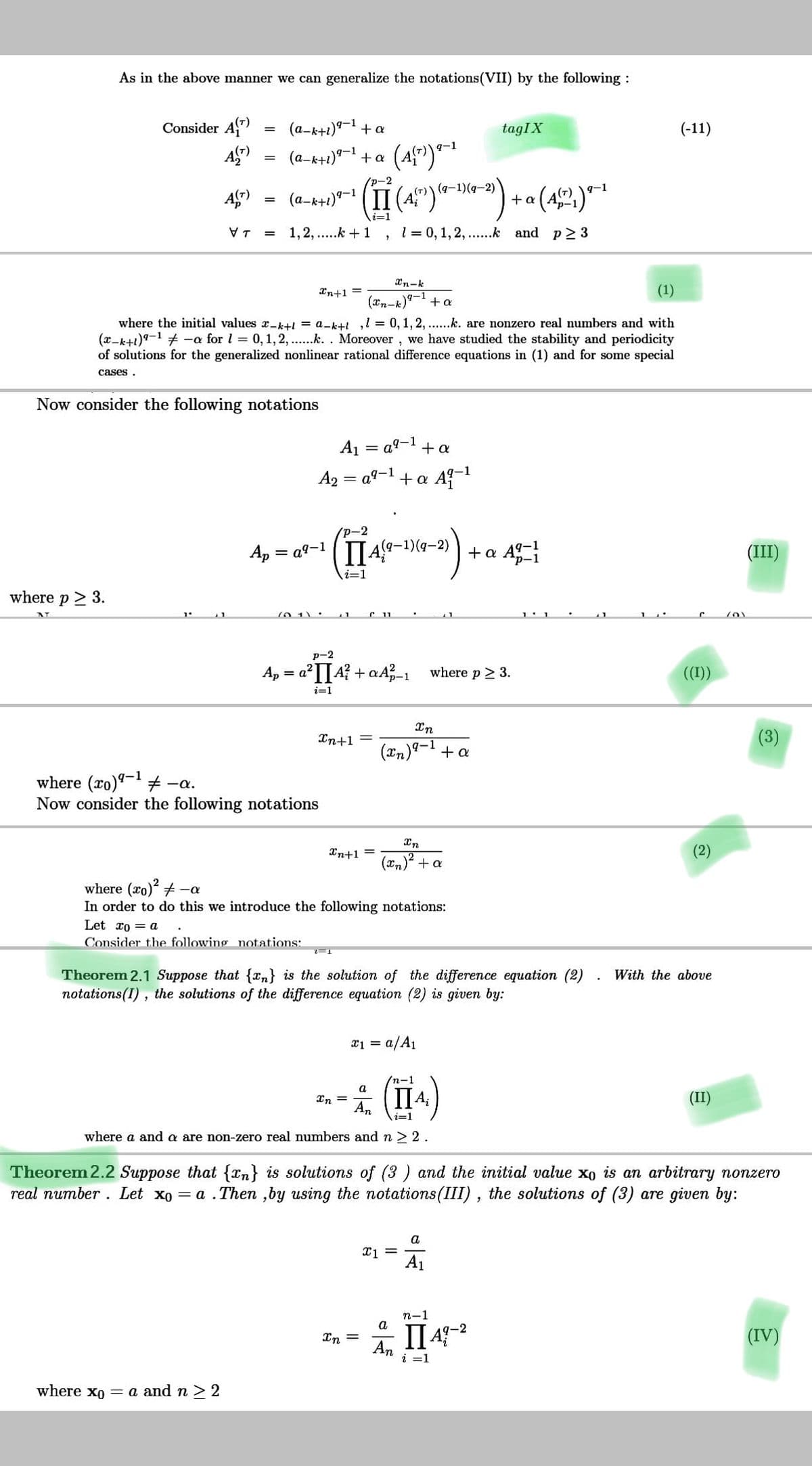
Transcribed Image Text:As in the above manner we can generalize the notations(VII) by the following:
(a-k+1)-1+ a
(a-k+1) +a
Consider AT
tagIX
(-11)
q-1
9-1
(p-2
(q-1)(q-2)
(a-k+1)9-1
(42)**
+ a
%3D
i=1
1,2, ..k + 1
1 = 0, 1,2, ..k and p2 3
%3D
Xn-k
In+1 =
(1)
19-1
(xn-k) + a
where the initial values r-k+! = a-k+! ,l = 0,1, 2, ..k. are nonzero real numbers and with
(x-k+1)9-1 + -a for l = 0, 1, 2, ..k. . Moreover , we have studied the stability and periodicity
of solutions for the generalized nonlinear rational difference equations in (1) and for some special
cases .
Now consider the following notations
A1 = a4-1 + a
A2 = a"-1 +a A-
(p-2
I[49–1)(q-2)
(III)
Ap =
= a9-1
+ a A9-!
p-1
i=1
where p> 3.
р-2
Ap =
a'II4? + aA-1
where p > 3.
((I))
i=1
In
Xn+1
(3)
q-1
(xn)"- + a
where (ro)- # -a.
Now consider the following notations
Xn+1
(xn) + a
where (xo) + -a
In order to do this we introduce the following notations:
Let xo = a
Consider the following otations:
Theorem 2.1 Suppose that {xn} is the solution of the difference equation (2)
notations(I), the solutions of the difference equation (2) is given by:
With the above
a/A1
n-1
a
Xn =
An
(II)
i=1
where a and a are non-zero real numbers and n > 2.
Theorem 2.2 Suppose that {xn} is solutions of (3 ) and the initial value Xo is an arbitrary nonzero
real number. Let xo = a .Then ,by using the notations(III) , the solutions of (3) are given by:
a
A1
n-1
a
In =
An
i =1
II 49-3
(IV)
where xo = a and n > 2
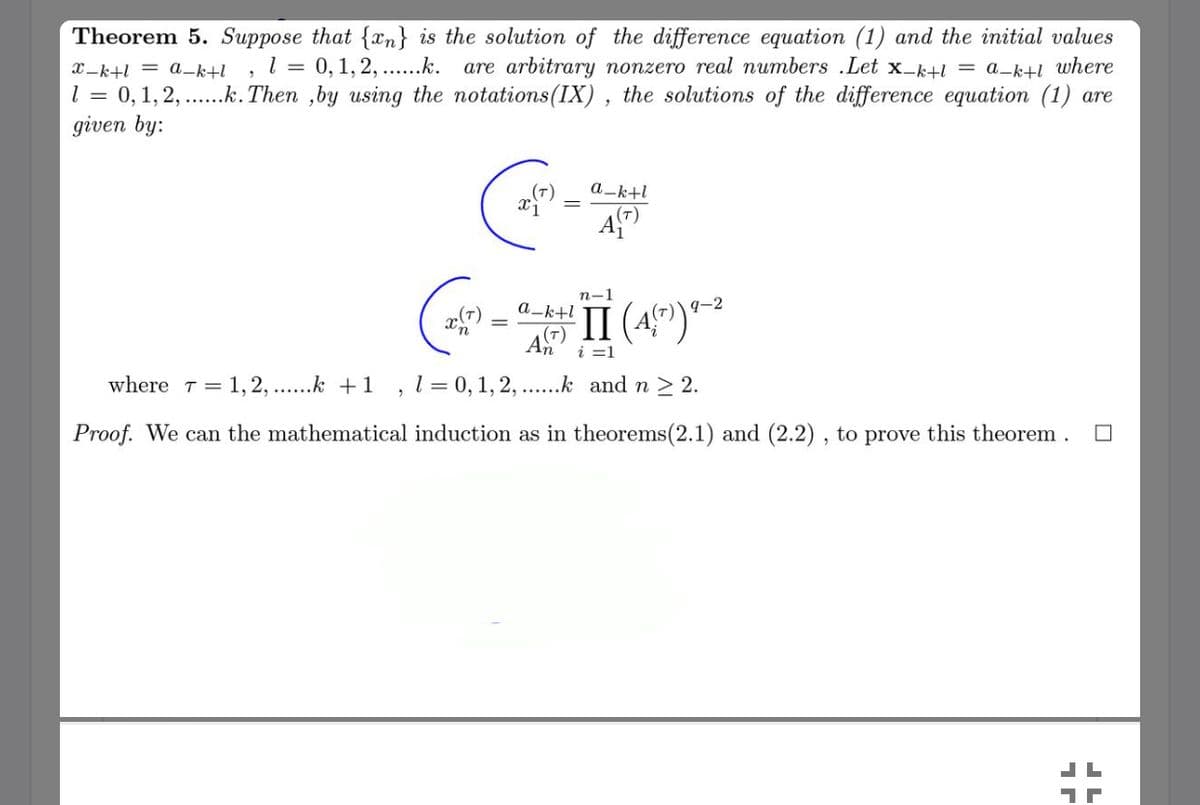
Transcribed Image Text:Theorem 5. Suppose that {xn} is the solution of the difference equation (1) and the initial values
x-k+l = a_k+l
l = 0,1, 2, ....k.
are arbitrary nonzero real numbers .Let x_k+l = a_ktI where
l = 0,1, 2, ..k.Then ,by using the notations(IX), the solutions of the difference equation (1) are
given by:
(7)
a_k+l
(7)
n-1
a_k+l
q-2
II (4")**
(T)
An
i =1
where T = 1, 2, ...k +1
l = 0, 1, 2, ....k and n > 2.
Proof. We can the mathematical induction as in theorems(2.1) and (2.2) , to prove this theorem.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 4 steps
Recommended textbooks for you
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
