In this paper, we are concerned with the asymptotic properties of solutions of the third order neutral difference equation A(a,A(b,(Azn)“)+ 9ny+1 = 0, n> no 20, (1.1) where zn = yn + Pnyo(n), a is the ratio of odd positive integers, and the following conditions are assumed to hold throughout: (H1) {an}, {bn}, and {qn} are positive real sequences for all n> no; (H2) {Pn} is a nonnegative real sequence with 0 < Pn Sp< 1; (H3) {o(n)} is a sequence of integers such that o(n) >n for all n > no; +0o and Ln=no Va =+00, (H4) En=no un b
In this paper, we are concerned with the asymptotic properties of solutions of the third order neutral difference equation A(a,A(b,(Azn)“)+ 9ny+1 = 0, n> no 20, (1.1) where zn = yn + Pnyo(n), a is the ratio of odd positive integers, and the following conditions are assumed to hold throughout: (H1) {an}, {bn}, and {qn} are positive real sequences for all n> no; (H2) {Pn} is a nonnegative real sequence with 0 < Pn Sp< 1; (H3) {o(n)} is a sequence of integers such that o(n) >n for all n > no; +0o and Ln=no Va =+00, (H4) En=no un b
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
show me the steps determine blue and all information is here
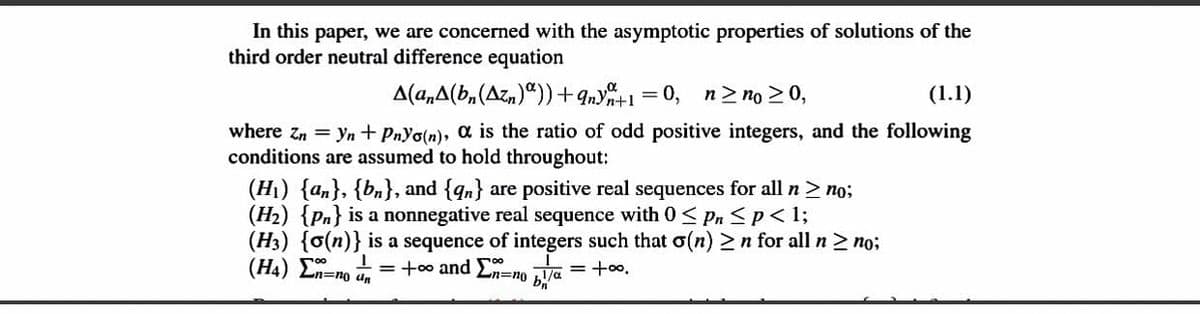
Transcribed Image Text:In this paper, we are concerned with the asymptotic properties of solutions of the
third order neutral difference equation
A(a,A(b,(Azn)“)) +9ny%+1 =0, n> no > 0,
(1.1)
where zn = yn + PnYo(n), a is the ratio of odd positive integers, and the following
conditions are assumed to hold throughout:
(H1) {an}, {bn}, and {qn} are positive real sequences for all n> no;
(H2) {Pn} is a nonnegative real sequence with 0 < Pn Sp< 1;
(H3) {o(n)} is a sequence of integers such that o(n) >n for all n > no;
(H4) En=no dn
= +0o and En=no Va
'00+ = D/1
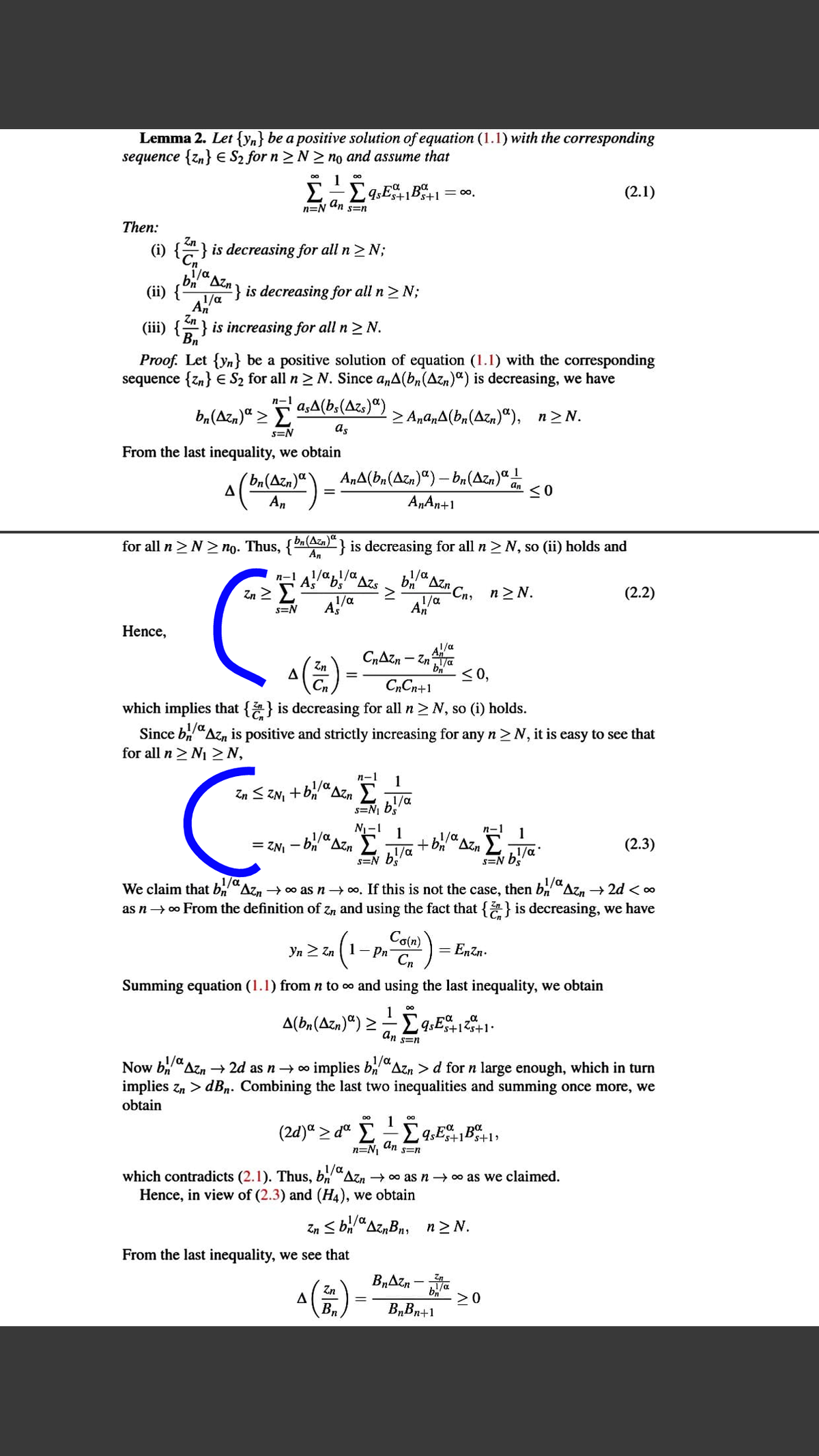
Transcribed Image Text:Lemma 2. Let {yn} be a positive solution of equation (1.1) with the corresponding
sequence {zn} E S2 for n > N > no and assume that
1
E-Eq,E+1B5+1= 0.
(2.1)
n=N Un s=n
Then:
(i) {} is decreasing for all n> N;
*Azn
(ii) {
} is decreasing for all n> N;
Zn
(iii) {} is increasing for all n >N.
Bn
Proof. Let {yn} be a positive solution of equation (1.1) with the corresponding
sequence {zn} € S2 for all n > N. Since a,A(b,(Azn)") is decreasing, we have
n-1
ba(Azn)“ > E
aşA(b,(Azs)“)
> AnanA(bn(Azn)"), n>N.
s=N
as
From the last inequality, we obtain
´bn(Azn)ª
AnA(bn(Azn)") – a(Azn)“
1
An
A„An+1
for all n>N > no.
Thus,
ba(Azn)"}
is decreasing for all n > N, so (ii) holds and
An
п-1
1/a1/a
Azs
bn
1/a
Azn
Zn 2 E
Cn, n N.
1/a
An
(2.2)
1/a
AS
s=N
Hence,
1/a
(2)
CrAzn - Zna
<0,
Zn
C„Cn+1
which implies that {} is decreasing for all n> N, so (i) holds.
1/a
Since bi"Azn is positive and strictly increasing for any n >N, it is easy to see that
for all n > N1 >N,
n-1
1
Zn < ZN, + BCAZ.
Σ
1/a
s=N bs
1/a
N-1
1
= ZNI - bi"Azn E
s=N b!/a
1/a
1/a
1
Azn
(2.3)
s=N b/a
1/a
We claim that b,
as n→ o From the definition of Zn and using the fact that { } is decreasing, we have
Azn → 00 as n→ 0, If this is not the case, then b,Az, → 2d < ∞
Co(n)
Yn 2 Zn 1- Pn
Cn
= Enzn.
Summing equation (1.1) from n to o and using the last inequality, we obtain
1
A(bn(Azn)ª) >-Ë
An s=n
/a
Now b"Azn → 2d as n → o implies b"Azn > d for n large enough, which in turn
/a
implies zn > dBn. Combining the last two inequalities and summing once more, we
obtain
1
(2d)ª > dª E £
n=N, an
Ps+1,
S=n
which contradicts (2.1). Thus, b
Hence, in view of (2.3) and (H4), we obtain
Azn → 00 as n → 00 as we claimed.
Zn S b/a
From the last inequality, we see that
"AznBn, n2 N.
B„Azn
Zn
>0
Bn
B„Bn+1
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 3 steps

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

