Solve quetion 3.5.7.9
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
Solve quetion 3.5.7.9
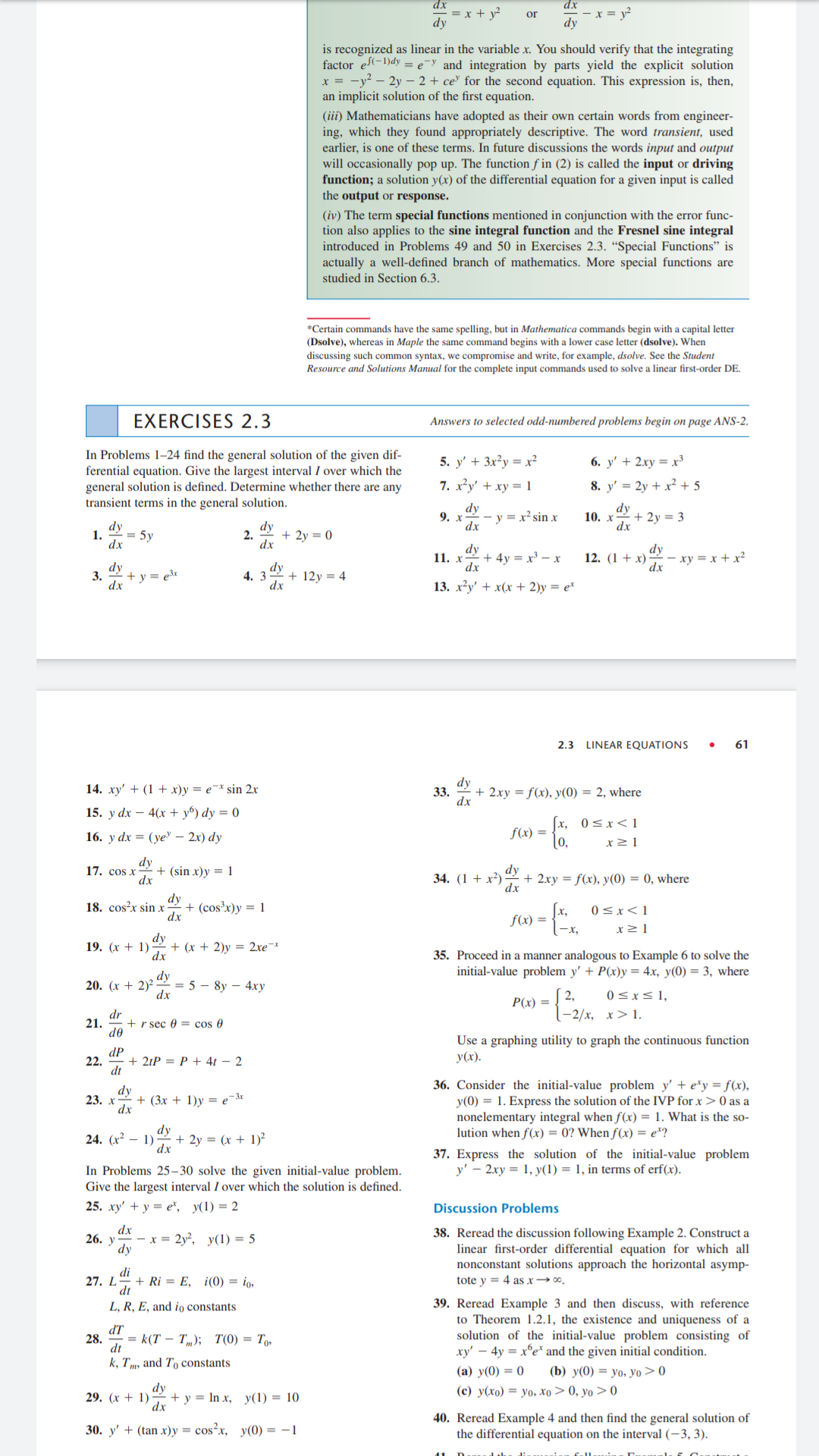
Transcribed Image Text:dx
= x + y?
dy
dx
- x = y
dy
or
is recognized as linear in the variable x. You should verify that the integrating
factor e-1)dy = e¯y and integration by parts yield the explicit solution
x = -y² – 2y - 2 + ce" for the second equation. This expression is, then,
an implicit solution of the first equation.
(iii) Mathematicians have adopted as their own certain words from engineer-
ing, which they found appropriately descriptive. The word transient, used
earlier, is one of these terms. In future discussions the words input and output
will occasionally pop up. The function f in (2) is called the input or driving
function; a solution y(x) of the differential equation for a given input is called
the output or response.
(iv) The term special functions mentioned in conjunction with the error func-
tion also applies to the sine integral function and the Fresnel sine integral
introduced in Problems 49 and 50 in Exercises 2.3. "Special Functions" is
actually a well-defined branch of mathematics. More special functions are
studied in Section 6.3.
*Certain commands have the same spelling, but in Mathematica commands begin with a capital letter
(Dsolve), whereas in Maple the same command begins with a lower case letter (dsolve). When
discussing such common syntax, we compromise and write, for example, dsolve. See the Student
Resource and Solutions Manual for the complete input commands used to solve a linear first-order DE.
EXERCISES 2.3
Answers to selected odd-numbered problems begin on page ANS-2.
In Problems 1-24 find the general solution of the given dif-
ferential equation. Give the largest interval I over which the
general solution is defined. Determine whether there are any
transient terms in the general solution.
5. y' + 3x²y = x²
6. y' + 2xy = x³
7. х?у + ху 3 1
8. y' = 2y + x² + 5
dy
dy
9. х
y = x² sin x
10. х
+ 2y = 3
dy
dy
dx
dx
1.
= 5y
2.
+ 2y = 0
dx
dx
dy
dy
12. (1 + x) – xy = x + x²
dx
11. x
+ 4y = x³ – x
dy
dy
´dx
3.
+ y = e3r
4. 3
+ 12y = 4
dx
dx
13. x?y' + x(x + 2)y = e*
2.3 LINEAR EQUATIONS
61
dy
14. xy' + (1 + x)y = e¬* sin 2x
33.
+ 2xy = f(x), y(0) = 2, where
dx
15. у dx — 4(х + yб) dy 3D 0
(x, 0<x<1
f(x) =
[0,
16. у dx %3D (yеУ — 2х) dy
x21
dy
+ (sin x)y = 1
dx
17. cos x
dy
+ 2xy = f(x), y(0) = 0, where
dx
34. (1 + x?)
dy
+ (cos'x)y = 1
dx
18. cos?x sin x
Sx,
f(x) =
0 <x<1
dy
+ (x + 2)y = 2xe¯*
dx
19. (х + 1) —
35. Proceed in a manner analogous to Example 6 to solve the
initial-value problem y' + P(x)y = 4x, y(0) = 3, where
dy
3 5 — 8у — 4ху
dx
20. (x + 2)
2,
P(x) =
0 <x<1,
—2/х, х> 1.
dr
21.
+ r sec 0 = cos 0
de
Use a graphing utility to graph the continuous function
y(x).
dP
22.
+ 2tP = P + 4t – 2
dt
36. Consider the initial-value problem y' + e*y = f(x),
y(0) = 1. Express the solution of the IVP for x>0 as a
nonelementary integral when f(x) = 1. What is the so-
lution when f(x) = 0? When f(x) = e*?
dy
23. х
+ (3x + 1)y = e¯3x
dx
dy
24. (x² – 1)
+ 2y = (x + 1)²
dx
37. Express the solution of the initial-value problem
y' – 2xy = 1, y(1) = 1, in terms of erf(x).
In Problems 25– 30 solve the given initial-value problem.
Give the largest interval I over which the solution is defined.
25. ху' + у %3D e', у(1) %3 2
Discussion Problems
dx
38. Reread the discussion following Example 2. Construct a
linear first-order differential equation for which all
nonconstant solutions approach the horizontal asymp-
tote y = 4 as x→ 0.
26. У
- x =
2y², y(1) = 5
dy
di
+ Ri = E, i(0) = io,
dt
27. L
39. Reread Example 3 and then discuss, with reference
to Theorem 1.2.1, the existence and uniqueness of a
solution of the initial-value problem consisting of
xy' – 4y = x°e* and the given initial condition.
(b) у(0) 3D yо, yo > 0
L, R, E, and io constants
LP
= k(T – T„); T(0) = T0,
dt
28.
k, Tm, and To constants
(а) у(0) — 0
dy
(c) y(xo) = yo, xo > 0, yo > 0
+ y = In x, y(1) = 10
dx
29. (х + 1)
40. Reread Example 4 and then find the general solution of
the differential equation on the interval (–3, 3).
30. y' + (tan x)y = cos²x, y(0) = -1
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 6 steps with 5 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

