College Physics
11th Edition
ISBN: 9781305952300
Author: Raymond A. Serway, Chris Vuille
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Related questions
Question
![### Use of the Parallel Axis Theorem to Determine Moment of Inertia
**Problem Statement:**
Using the parallel axis theorem, what is the moment of inertia of the rod of mass \( m \) about the axis shown below? (Use the following as necessary: \( m \) and \( L \).)
**Diagram Description:**
The diagram displays a horizontal rod with a length of \( L \). The rod is horizontally aligned, and a vertical axis of rotation is shown passing through the left end of the rod. The sections along the rod are labeled as follows:
- The first section from the axis to a point \( \frac{L}{3} \) from the left end.
- The remaining section from the \( \frac{L}{3} \) point to the far right end, labeled \( \frac{2L}{3} \).
An arrow above the rod illustrates the rotational direction about the vertical axis through the left end.
**Equation:**
To solve the moment of inertia \( I \) for this scenario, use the parallel axis theorem, which is given by:
\[ I = I_{\text{CM}} + md^2 \]
Where:
- \( I_{\text{CM}} \) is the moment of inertia about the center of mass of the rod.
- \( m \) is the mass of the rod.
- \( d \) is the distance between the new axis and the axis through the center of mass.
Generally, for a uniform rod of mass \( m \) and length \( L \), the moment of inertia about its center of mass \( I_{\text{CM}} \) is:
\[ I_{\text{CM}} = \frac{1}{12}mL \]
For this problem, the distance \( d \) from the center of mass to the new axis at the left end is \( \frac{L}{2} \).
So,
\[ d = \frac{L}{2} \]
Plugging these values into the parallel axis theorem,
\[ I = \frac{1}{12}mL^2 + m\left(\frac{L}{2}\right)^2 \]
Simplify,
\[ I = \frac{1}{12}mL^2 + m\frac{L^2}{4} \]
\[ I = \frac{1}{12}mL^2 + \frac{1}{4}mL^2 \](https://content.bartleby.com/qna-images/question/d0afef86-41bd-49f7-9deb-bb70dd2f5172/c8619fea-b4f8-46e0-b101-9446bb2614a0/bgoadtj.png)
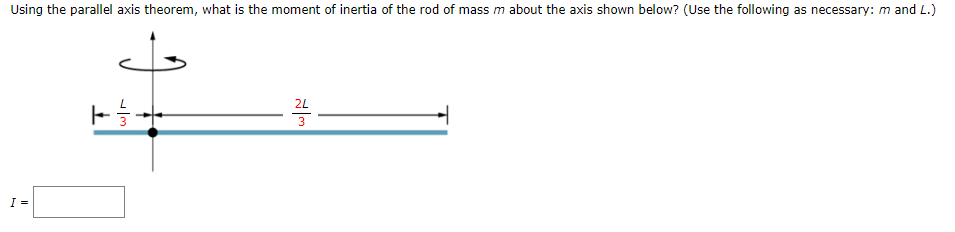
Transcribed Image Text:### Use of the Parallel Axis Theorem to Determine Moment of Inertia
**Problem Statement:**
Using the parallel axis theorem, what is the moment of inertia of the rod of mass \( m \) about the axis shown below? (Use the following as necessary: \( m \) and \( L \).)
**Diagram Description:**
The diagram displays a horizontal rod with a length of \( L \). The rod is horizontally aligned, and a vertical axis of rotation is shown passing through the left end of the rod. The sections along the rod are labeled as follows:
- The first section from the axis to a point \( \frac{L}{3} \) from the left end.
- The remaining section from the \( \frac{L}{3} \) point to the far right end, labeled \( \frac{2L}{3} \).
An arrow above the rod illustrates the rotational direction about the vertical axis through the left end.
**Equation:**
To solve the moment of inertia \( I \) for this scenario, use the parallel axis theorem, which is given by:
\[ I = I_{\text{CM}} + md^2 \]
Where:
- \( I_{\text{CM}} \) is the moment of inertia about the center of mass of the rod.
- \( m \) is the mass of the rod.
- \( d \) is the distance between the new axis and the axis through the center of mass.
Generally, for a uniform rod of mass \( m \) and length \( L \), the moment of inertia about its center of mass \( I_{\text{CM}} \) is:
\[ I_{\text{CM}} = \frac{1}{12}mL \]
For this problem, the distance \( d \) from the center of mass to the new axis at the left end is \( \frac{L}{2} \).
So,
\[ d = \frac{L}{2} \]
Plugging these values into the parallel axis theorem,
\[ I = \frac{1}{12}mL^2 + m\left(\frac{L}{2}\right)^2 \]
Simplify,
\[ I = \frac{1}{12}mL^2 + m\frac{L^2}{4} \]
\[ I = \frac{1}{12}mL^2 + \frac{1}{4}mL^2 \
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 2 images
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- A thing solid cylinder has a mass 6.98kg and a length 1.66 m. What is its moment of inertia (in unit of kg.m2) when it is rotating as shown above? (please search your physics text book or other sources for the correct formula for this situation, you lab manual does not necessarily have the formula.)arrow_forwardA pulley with moment of inertia I = 0.75 kg-m² and radius R = 15 cm is mounted on a wall. A light string is wrapped around the pulley with a mass m = 2.0 kg attached to the end. The pulley rotates as the mass falls. Use Newton's second law to calculate the acceleration of the mass. Check that your formula gives the expected behavior when I →0. => To help get started, the free body diagrams m and the pulley is shown below. T m Write down Newton's second law for each diagram. Use (and explain) the relationship R a = RO TIL R Tarrow_forwardPlease help mearrow_forward
- Rank the following in order of increasing moments of inertia about the midpoint of the rod. m R 1 m 2m R/2 2 m m/2 2R 3 m/2arrow_forwardFind the moment of inertia for a uniform rod (mass m and length L) about an axis at the position shown in the figurearrow_forwardCalculate the moment of inertia of a skater given the following information. a) The 70-kg skater is approximated as a cylinder that has a 0.144-m radius. kg m? b) The skater with arms extended is approximately a cylinder that is 50.1 kg, has a 0.144-m radius, and has two 0.973-m-long arms which are 3.92 kg each and extend straight out from the cylinder like rods rotated about their ends. kg m?arrow_forward
- Consider two objects with m₁ > m2 connected by a light string that passes over a pulley having a moment of inertia of I about its axis of rotation as in the figure below. The string does not slip on the pulley or stretch. The pulley turns without friction. The two objects are released from rest separated by a vertical distance 2h. (Use any variable or symbol stated above along with the following as necessary: g and R.) m1 2h m2 (a) Use the principle of conservation of energy to find the translational speeds of the masses as they pass each other. v = (b) Find the angular speed of the pulley at this time. =3arrow_forwardA metal plate is fixed at point B. Several forces are loaded onto the plate Question 5 as shown. Calculate the total moment about point B due to these loads. FA = 20 N FB = 30 N Fc = 20 N FD = 30 N 0 = 30°arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press 
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
University Physics (14th Edition)
Physics
ISBN:9780133969290
Author:Hugh D. Young, Roger A. Freedman
Publisher:PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:9781107189638
Author:Griffiths, David J., Schroeter, Darrell F.
Publisher:Cambridge University Press
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:9780321820464
Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:Addison-Wesley
College Physics: A Strategic Approach (4th Editio...
Physics
ISBN:9780134609034
Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:PEARSON