1. Any function y = f(x) can be expressed as x = f(y) in standard mathematical methods for solving areas between %3D %3! curves.
1. Any function y = f(x) can be expressed as x = f(y) in standard mathematical methods for solving areas between %3D %3! curves.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
Which of the following statements are correct?
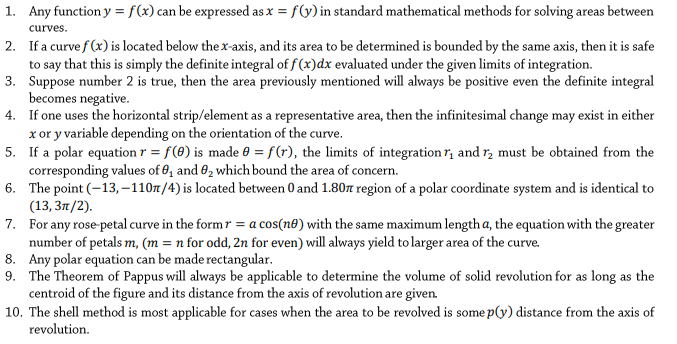
Transcribed Image Text:1. Any function y = f(x) can be expressed as x = f(y) in standard mathematical methods for solving areas between
curves.
2. If a curve f (x) is located below the x-axis, and its area to be determined is bounded by the same axis, then it is safe
to say that this is simply the definite integral of f (x)dx evaluated under the given limits of integration.
3. Suppose number 2 is true, then the area previously mentioned will always be positive even the definite integral
becomes negative.
4. If one uses the horizontal strip/element as a representative area, then the infinitesimal change may exist in either
x or y variable depending on the orientation of the curve.
5. If a polar equation r = f(0) is made 0 = f(r), the limits of integration r, and r, must be obtained from the
corresponding values of 8, and 62 which bound the area of concern.
6. The point (-13,–110/4) is located between 0 and 1.80n region of a polar coordinate system and is identical to
(13, 31/2).
7. For any rose-petal curve in the form r = a cos(n®) with the same maximum length a, the equation with the greater
number of petals m, (m = n for odd, 2n for even) will always yield to larger area of the curve.
8. Any polar equation can be made rectangular.
9. The Theorem of Pappus will always be applicable to determine the volume of solid revolution for as long as the
centroid of the figure and its distance from the axis of revolution are given.
10. The shell method is most applicable for cases when the area to be revolved is some p(y) distance from the axis of
revolution.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 2 steps

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

