Y - K"(LE) The economy has a capital share of 0.20, a saving rate of 45 percent, a depreciation rate of 3.75 percent, a rate of population growth of 5.00 percent, and a rate of labor-augmenting technological change of 3.5 percent. It is in steady state. b. Solve for capital per effective worker (k"), output per elffective worker (y"), and the marginal product of capital. k' - y* = marginal product of capital =
Y - K"(LE) The economy has a capital share of 0.20, a saving rate of 45 percent, a depreciation rate of 3.75 percent, a rate of population growth of 5.00 percent, and a rate of labor-augmenting technological change of 3.5 percent. It is in steady state. b. Solve for capital per effective worker (k"), output per elffective worker (y"), and the marginal product of capital. k' - y* = marginal product of capital =
Essentials of Economics (MindTap Course List)
8th Edition
ISBN:9781337091992
Author:N. Gregory Mankiw
Publisher:N. Gregory Mankiw
Chapter17: Production And Growth
Section: Chapter Questions
Problem 4CQQ
Related questions
Question
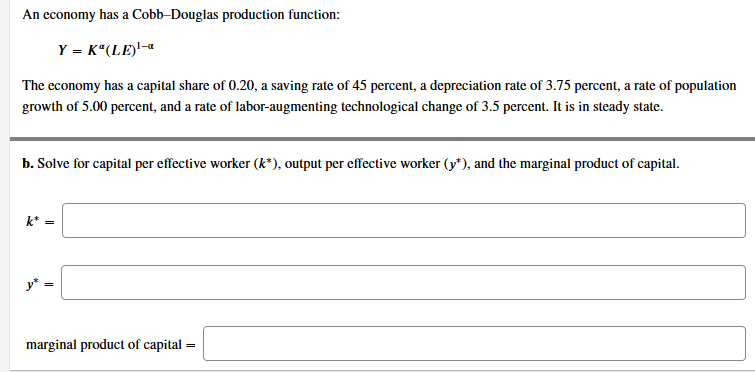
Transcribed Image Text:Y - K"(LE)
The economy has a capital share of 0.20, a saving rate of 45 percent, a depreciation rate of 3.75 percent, a rate of population
growth of 5.00 percent, and a rate of labor-augmenting technological change of 3.5 percent. It is in steady state.
b. Solve for capital per effective worker (k"), output per elffective worker (y"), and the marginal product of capital.
k' -
y* =
marginal product of capital =
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 4 steps with 6 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, economics and related others by exploring similar questions and additional content below.Recommended textbooks for you

Essentials of Economics (MindTap Course List)
Economics
ISBN:
9781337091992
Author:
N. Gregory Mankiw
Publisher:
Cengage Learning

Brief Principles of Macroeconomics (MindTap Cours…
Economics
ISBN:
9781337091985
Author:
N. Gregory Mankiw
Publisher:
Cengage Learning

Principles of Economics 2e
Economics
ISBN:
9781947172364
Author:
Steven A. Greenlaw; David Shapiro
Publisher:
OpenStax

Essentials of Economics (MindTap Course List)
Economics
ISBN:
9781337091992
Author:
N. Gregory Mankiw
Publisher:
Cengage Learning

Brief Principles of Macroeconomics (MindTap Cours…
Economics
ISBN:
9781337091985
Author:
N. Gregory Mankiw
Publisher:
Cengage Learning

Principles of Economics 2e
Economics
ISBN:
9781947172364
Author:
Steven A. Greenlaw; David Shapiro
Publisher:
OpenStax