
Algebra and Trigonometry (6th Edition)
6th Edition
ISBN: 9780134463216
Author: Robert F. Blitzer
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Question
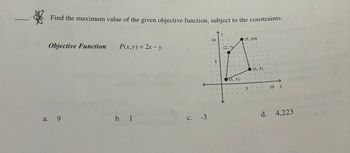
Transcribed Image Text:-
Find the maximum value of the given objective function, subject to the constraints.
Objective Function
a. 9
P(x, y) = 2x - y
b. 1
c. -3
- 10
5
y
(2,7)
.
(1,1).
(5, 10)
5
(6,3)
· 10 x
d. 4,223
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 7 images

Knowledge Booster
Similar questions
- The graph shows a region of feasible solutions. Use this region to find maximum and minimum values of the objective function. z=0.25x+0.60y Select the correct choice below and, if necessary, fill in the answer box to complete your choice. A. The maximum value is B. There is no maximum value. Ay 10- 04- 0 Q The coordinates of the corner points are (0,0), (0,7), (3,10), (7,8), and (9,0).arrow_forwardFind the maximum of the objective function C 2x + 9y subject to the feasibility region below. 12 10 7- 5- 4 10 11 12 The maximum occurs at the point:| to 2.arrow_forwardFind the absolute maximum and minimum values of the function, subject to the given constraints. 2 2 k(x,y)= -x -y +6x+6y; 0≤x≤ 4, y ≥0, and x+y≤8 The minimum value of k is 1. (Simplify your answer.) The maximum value of k is (Simplify your answer.)arrow_forward
- Find the maximum value of the objective function z = 8x + 2y, subject to the following constraints. (See Example 2.) 5x + y ≤ 35 3x + y ≤ 27 x ≥ 0, y ≥ 0 The maximum value is z = at (x, y) = Additional Materials BeBookarrow_forwardA rectangular tank with a square base, an open top, and a volume of 8,788 ft³ is to be constructed of sheet steel. Find the dimensions of the tank that has the minimum surface area. Let s be the length of one of the sides of the square base and let A be the surface area of the tank. Write the objective function. A = (Type an expression.) The interval of interest of the objective function is. (Simplify your answer. Type your answer in interval notation.) The tank with the minimum surface area has a height of ft and a square base with a sidelength of ft. H (...) Q Search acer *arrow_forwardCan you help me solve?arrow_forward
- Find the values of x and y that maximize the objective function P=3x+2yP=3x+2y for the graph. What is the maximum value?arrow_forwardUse the slider to drag the graph of the objective function M = 8x + 7y The maximum value of the objective function obtained within the feasible region (shaded pink) is 26 24 22 M=0 18 16 14 (0, 12) 12 10 (9, 9) |(14, 4) (16, 0) M=8x+ 7y 14arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning

Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON

Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press

College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education