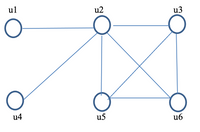
If A is NP-complete, and A has a polynomial time algorithm, then there is a polynomial time algorithm to find the largest clique in a directed. A clique in a graph G(V, E) is a subset of vertices V’ such that there is an edge (u,v) in E between every two vertices u and v in V’. For example, the four vertices u2, u3,u5, and u6 form the largest clique in the graph below.

Let G be a graph, where each edge has a weight. A spanning tree is a set of edges that connects all the vertices together, so that there exists a path between any pair of vertices in the graph. A minimum-weight spanning tree is a spanning tree whose sum of edge weights is as small as possible. Last week we saw how Kruskal's Algorithm can be applied to any graph to generate a minimum-weight spanning tree. In this question, you will apply Prim's Algorithm on the graph below. You must start with vertex A. H 4 4 1 3 J 2 C 10 4 8 B 9 F 18 8 There are nine edges in the spanning tree produced by Prim's Algorithm, including AB, BC, and IJ. Determine the exact order in which these nine edges are added to form the minimum-weight spanning tree. 3.

Most graph algorithms that take an n×n adjacency-matrix representation as input require at least time O(n^2), but there are some exceptions. Show how to determine whether a simple directed graph G contains a universal sink, that is, a vertex with in degree n − 1 and out-degree 0, in time O(n) given an n × n adjacency matrix for G. (A vertex v has indegree k if there are precisely k edges of the form (u, v), and has outdegree k if there are precisely k edges of the form (v, u).)

Given a digraph, find a bitonic shortest path from s to every other vertex (if one exists). A path is bitonic if there is an intermediate vertex v suchthat the edges on the path from s to v are strictly increasing and the edges on the pathfrom v to t are strictly decreasing. The path should be simple (no repeated vertices).

Shortest paths. Let G = (V,E) be an acyclic weighted directed graph and let s ∈ V be an arbitrary vertex. Describe an algorithm which in time O(|V | + |E|) finds shortest paths from s to all (reachable from s) vertices in the graph G, represented by an adjacency list.

Discrete mathematics. Let G = (V, E) be a simple graph4 with n = |V| vertices, and let A be its adjacency matrix of dimension n × n. We want to count the L-cycles : such a cycle, denoted by C = u0u1 · · · uL with  uL = u0 contains L distinct vertices u0, . . . , uL-1 et L edges E(C) = {uiui+1 | 0 ≤ i ≤ L − 1} ⊆ E. Two cycles are distinct if the edge sets are different : C = C' if and only if E(C) = E(C'). We define the matrices D, T, Q, the powers of A by matrix multiplication : D = A · A = A2,   T = A · D = A3,   Q = A · T = A4. Consider the values on the diagonals. Prove that the number of 3-cycles N3 in the whole graph is N3 = 1/6 ∑ u∈V   Tu,u

Let G be a graph with V vertices and E edges. One can implement Kruskal's Algorithm to run in O(E log V) time, and Prim's Algorithm to run in O(E + V log V) time. If G is a dense graph with an extremely large number of vertices, determine which algorithm would output the minimum-weight spanning tree more quickly. Clearly justify your answer.