Theorem 1. Every positive integer n > 2 can be written as a product of primes. In other words, for each n E Z4 with n > 2, there is r e Z4 and primes p1, P2, -..., Pr such that П Pi = P1P2 · ·• Pr. n = i=1
Theorem 1. Every positive integer n > 2 can be written as a product of primes. In other words, for each n E Z4 with n > 2, there is r e Z4 and primes p1, P2, -..., Pr such that П Pi = P1P2 · ·• Pr. n = i=1
Linear Algebra: A Modern Introduction
4th Edition
ISBN:9781285463247
Author:David Poole
Publisher:David Poole
Chapter2: Systems Of Linear Equations
Section2.4: Applications
Problem 53EQ
Related questions
Question
can someone please show all the work for this? i have no idea how to do this
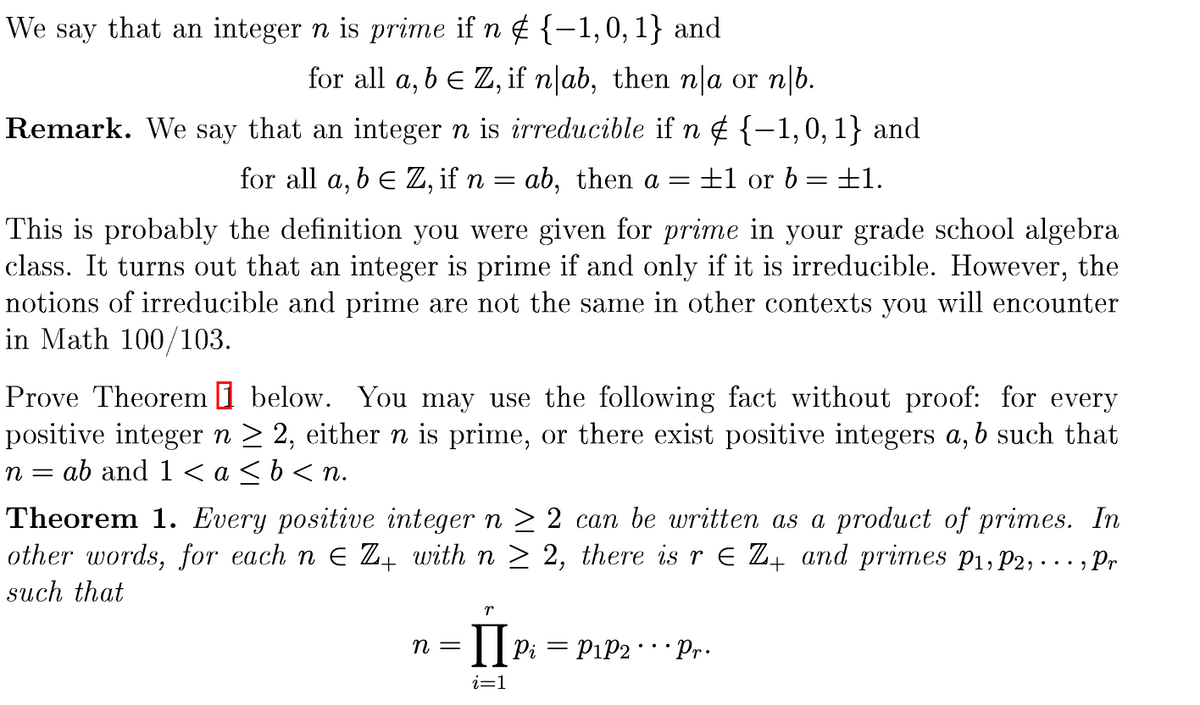
Transcribed Image Text:We say that an integer n is prime if n ¢ {-1,0,1} and
6.
for all a, b e Z, if n|ab, then n|a or n|b.
Remark. We say that an integer n is irreducible if n ¢ {-1,0,1} and
for all a, b E Z, if n = ab, then a
±1 or b =±E1.
This is probably the definition you were given for prime in your grade school algebra
class. It turns out that an integer is prime if and only if it is irreducible. However, the
notions of irreducible and prime are not the same in other contexts you will encounter
in Math 100/103.
Prove Theorem I below. You may use the following fact without proof: for every
positive integer n > 2, either n is prime, or there exist positive integers a, b such that
ab and 1 < a <b<n.
n =
Theorem 1. Every positive integer n > 2 can be written as a product of primes. In
other words, for each n E Z, with n > 2, there is r E Z4 and primes P1, P2, . .. , Pr
such that
п —
|| Pi = PiP2 Pr.
i=1
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 2 steps

Recommended textbooks for you

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage

Elements Of Modern Algebra
Algebra
ISBN:
9781285463230
Author:
Gilbert, Linda, Jimmie
Publisher:
Cengage Learning,
