What is a Domain?
In simple mathematics terms, a domain is defined as the space on which all the possible inputs for the function will lie. It can also be expressed as a set of departures for the required function.
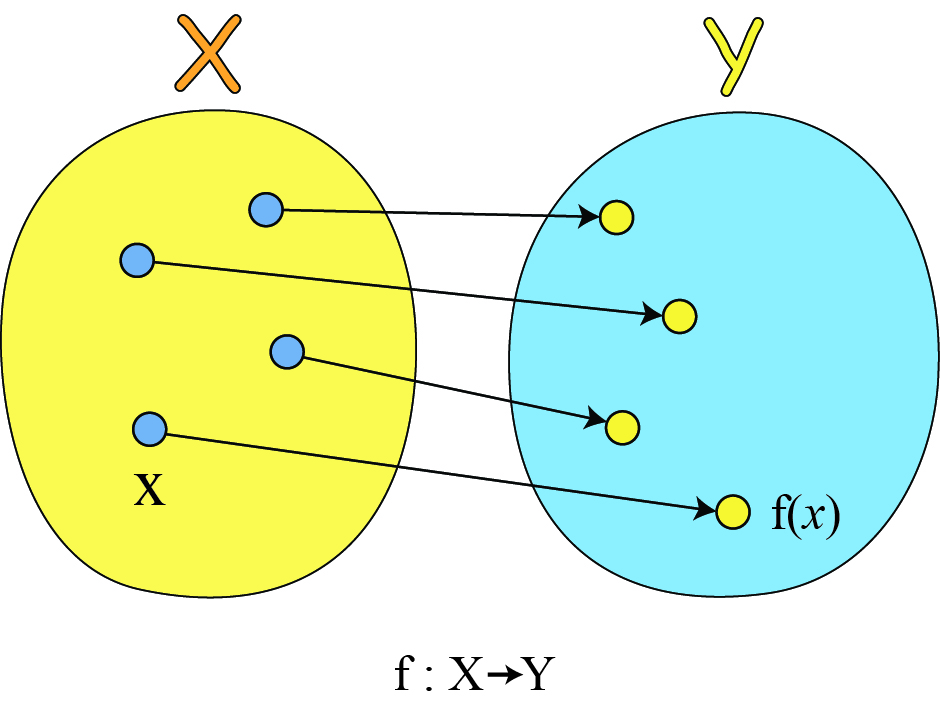
How does Domain Work?
Usually, two different sets are considered for a domain. The data is mapped from one of the sets to the other set with the help of the functions.
It should be noted that the function which is considered can be defined on the domain itself. In other words, every point of the domain satisfies the required function. That is how the domain is chosen. As a result, the domain of the definition of a function and the domain of the function coincide. This coincidence does not happen for partial functions though. This is because when a partial function is considered, its domain has at least all the elements that are present in the domain of definition. In other words, the domain acts as a superset for the partial function’s domain of definition.
Formula
If there are two sets A and B, such that the data is mapped from A to B, then it is written as:
f: A → B
Here, ‘f’ is the function that maps the elements from set A to set B.
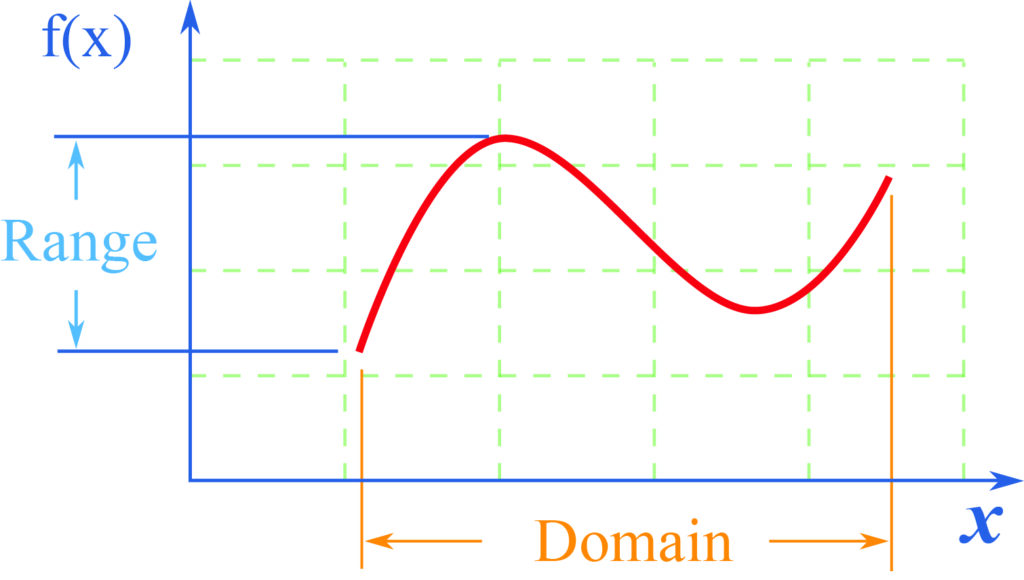
How is Domain Defined?
When a function is considered, it can be said to consist of a domain if the function can be defined on every point of an external data set. Also, the domain, codomain, and graph are all part of the function if the function is written in the form (X,Y,G), where X is the domain, Y is the codomain and G is the graph of the function.
If a function can be simply defined in the graphical form, then the domain can or cannot be the function’s part.
For instance, if we consider the cosine curve, then the set of real numbers, R, can be said to be the domain of the function. Similarly, if we consider the square root function, then its domain will be equal to the set of all numbers that are equal or greater than zero, considering that complex numbers have been excluded in both cases.
Usually, domains can be represented on the axes as well. If the Cartesian coordinate system is being used to represent a function, which has a domain of real number subset, then the x-axis can be used to represent the domain.
What is a Natural Domain?
The natural domain of a function consists of all the values on which the function can be properly defined. Usually, such domains can be taken for real numbers in all cases. In certain situations, complex numbers and integers can be taken as well. If the square root function is considered again, its natural domain will be consisting of the non-negative real numbers. This is when a real number function has been considered.
What is the Range?
If natural domains are being considered, then whatever values the function can attain, the set of all such values is defined as the range.
What is Category Theory?
In domains, the data is being mapped with the help of the functions, and they are represented by arrows. These arrows are also known as morphisms. When the morphisms are considered other than the functions, then the category theory comes into place. The formulation of the ideas regarding domains with respect to the category theory is constructed in a much more abstract manner. The object from which an arrow starts in a morphism is said to be the domain of the morphism.
Where is the Domain Found?
As stated before, the domain is the set of all possible values that act as inputs to the required function. The independent variables are also possible due to all of the values that lie in the domain.
Usually, the domain can be found in two main places. If the considered function is a fraction, then the domain is usually found with the denominator, by making it unequal to zero. The domain can also be found on the square root with the positive digit lying under it.
How to Find the Function’s Domain?
The domain of a function can be found out by many methods. But before proceeding to that, it is important to note the values that are taken by the independent variables can be used as per requirements. The most common cases include not having a negative number under the square root and not having a denominator of the fraction as zero.
In usual cases, the real number set, R, is the universal domain of all functions, except when the functions come with some specific types of restrictions. They are as follows:
- If the function is a normal linear equation or a quadratic equation, then the real number set R is the domain of such functions.
- If the function is in a fractional form, then the numbers, for which the denominator is zero, are excluded from the real number set R, and this new set is the domain.
- If the functions come with pre-specified intervals, the upper bound and the lower bound of the interval itself can be taken as the input, which is nothing but the domain.
- If there are values for which the said function is not possible to define, then those particular values are restricted to lie in the domain of the real number set R as well.
What are the Various Applications of Domain?
Domain has various applications in the field of mathematics. Apart from Calculus, domains are equally useful in other branches. For instance, the domain is defined in topology. In topology, the connected open set is a domain. It is also present in real analysis and complex analysis. In both cases, the open connected subset that lies in the vector space, be it real or complex, is its domain. If PDEs, or Partial Differential Equations are studied, then the open connected subset that lies on the Euclidean space, or Rn, can be said to be the domain. It is in this place that the unknown functions of the problems can be defined or posed.
Practice Problem
Find the domain for the function-
⇒ Since the given function is a fraction, the denominator is equated to non-zero.
Hence,
Hence, the set of all real numbers R excluding 1 is the domain of the given function.
Context and Applications
Domains are an integral part of the Calculus syllabus in mathematics. It is an important topic for school exams, board exams, college entrance exams, CSIR, NEET, IITJEE, ISI, CMI, and so on.
This topic is significant in the professional exams for both undergraduate and graduate courses, especially for
- Bachelor of Science in Mathematics
- Master of Science in Mathematics
Want more help with your calculus homework?
*Response times may vary by subject and question complexity. Median response time is 34 minutes for paid subscribers and may be longer for promotional offers.
Domain Homework Questions from Fellow Students
Browse our recently answered Domain homework questions.