What are Trigonometric Ratios?
Trigonometric ratios give values of trigonometric functions. It always deals with triangles that have one angle measuring 90 degrees. These triangles are right-angled. We take the ratio of sides of these triangles.
Where is Trigonometric Ratio found in Nature?
Suppose that you are going to visit the tallest building in the world. You are looking at the top point of the building from the ground a few meters away from the building. If someone asks you the height of the building, can you tell them even without measuring it? This is interesting. Since the building is straight, it would be perpendicular (90 degrees) to the ground. Now you can think of a right-angled triangle.
Suppose now you are standing on the top of the building and you know the height. Your friend is standing on the ground a few meters away from the building. Can you tell at what distance he is away from the building?
This is where trigonometry is applied. Trigonometry is the relation between the sides and angles of the triangle. Who discovered trigonometry? The Greeks were the first to construct trigonometry. Hipparchus first discovered trigonometric functions. Trigonometry was used for calculating the distance between the earth, stars, and the moon. By knowing the trigonometric ratios, we can answer all these questions. In the right triangles, one angle is 90. There are three angles in a triangle. What about the other two angles? We know that when we add all these angles the sum should be 180. So the other 2 angles when added should give 90. This means that these 2 angles will be less than 90 and they are called acute angles. Here for finding trigonometric ratios, we will solve for acute angles.
How to use Trigonometric Ratios to find Side Lengths?
Consider that there is a pot hanging from a stick placed on the ground. In this case, we have 3 sides. One is the distance of the stick from the ground to the place in the ground above which the pot is hanging. The second is the length of the stick from the ground to the point having the hook that bears the pot. The third is the distance from the hook having the pot to the ground. Now we will name these sides. Let us use trigonometry here.
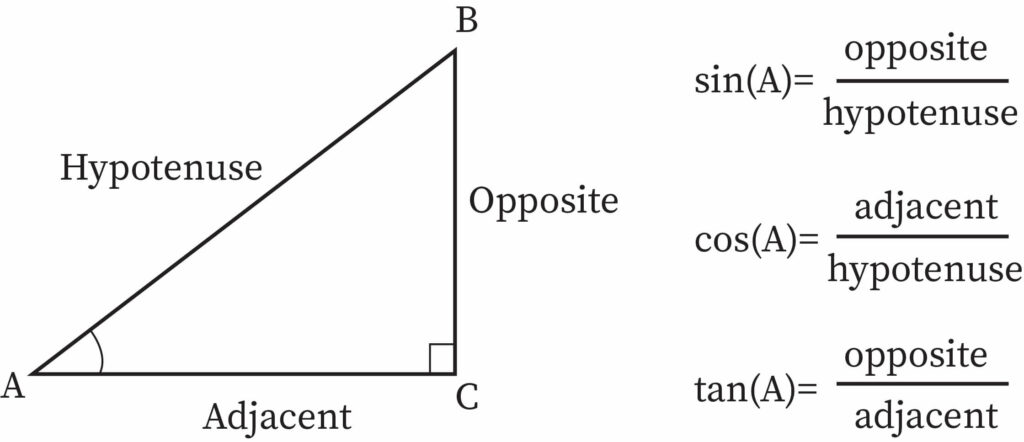
Now if you replace all these with lines and mark an angle between the stick and ground, you will get a new picture. This is a right triangle.
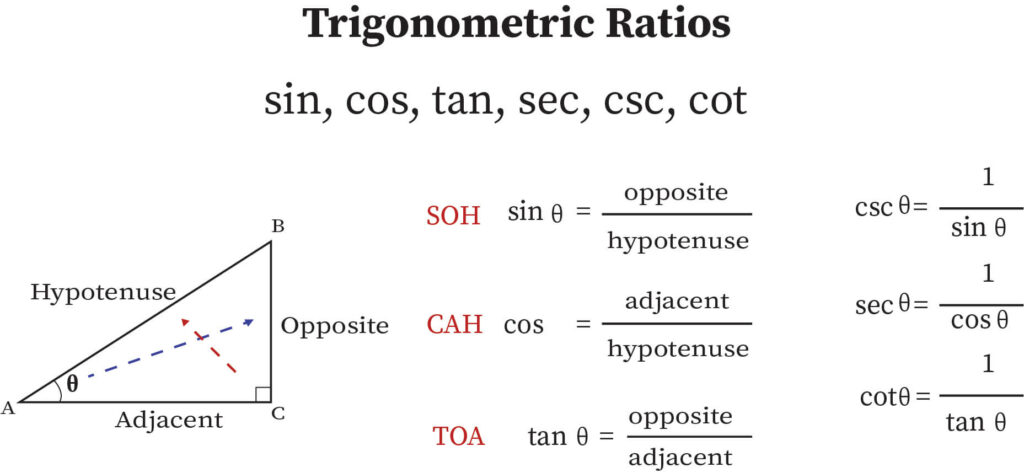
How to remember trigonometric ratios? We will see now. Can you tell from the picture which is the longest length? This is the side AC. We will call this hypotenuse. Place a torch at the angle we have marked and lit it. Then light falls on the pot. That is the opposite side to the angle. The last side is called the adjacent side. It is adjacent to angle A. Now you might have understood that trigonometry is used in finding sides of a triangle.
Now let us change the position of the torch.
Can we place this torch in B? But that is 90 degrees. Why cannot the trigonometric ratios be used on the right angle of a triangle? This is because some values become zero and infinite. This is one restriction in trigonometry.
How to Find Trigonometric Ratios of any Angle?
Let us place the torch at point C and mark an angle there. Here also the longest side is AC. But if you lit the torch, the light will fall on AB. So this is the opposite side to the angle here. And the side BC is the adjacent side. It is adjacent to the angle.
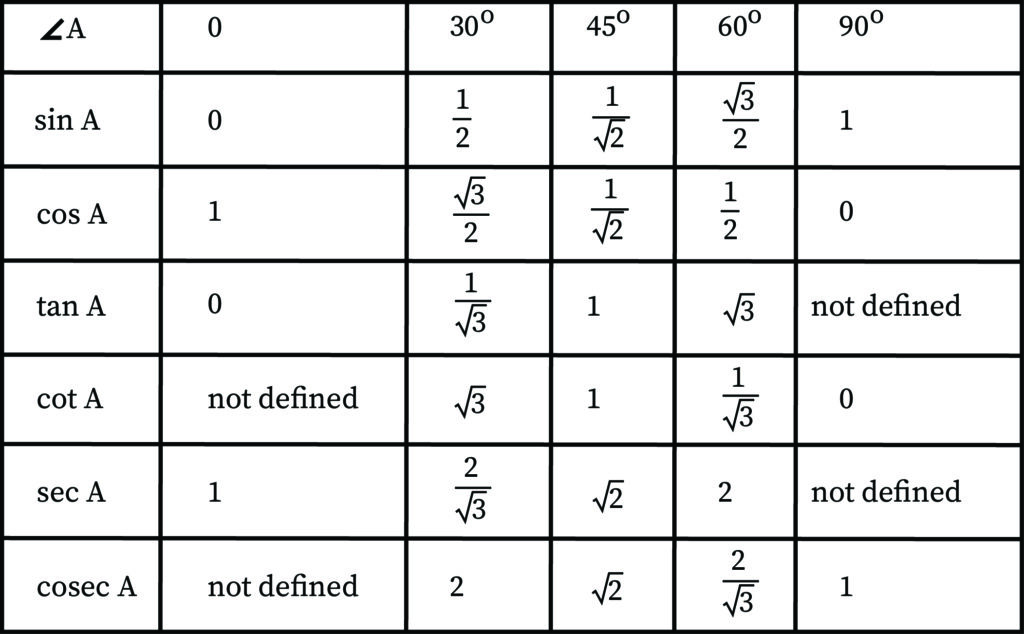
How many Trigonometric Ratios are there?
There are 6 trigonometric ratios called the trigonometric functions. Let us see how each is defined. We have seen about the sides placing a torch at A and we called it angle A. How do I find the trigonometric function?
- Take the ratio of the side that is opposite to the torchlight and the hypotenuse. We will define this as the sine of angle A. In short, it is called sin A.
- Take the ratio of the side that is adjacent to angle A and the hypotenuse. We will define this as the cosine of angle A. In short, it is called cos A.
- Take the ratio of the side that is opposite to angle A and the adjacent side. We will define this as the tangent of angle A. In short, it is called tan A. This is the same as taking the ratio of sin A and cos A.
How to remember trigonometric ratios? Remember SOH-CAH-TOA. S stands for the sine function, C stands for the cosine function, and T for the tangent. O is the opposite side, A is the adjacent side and H is the hypotenuse. These are the sides of a triangle.
How find the remaining trigonometric functions? They are the reciprocals of the first three trigonometric functions.
4. Take the multiplicative inverse of sin A. We define this as cosecant A. This is the ratio . This is called shortly as cosec A.
5. Take the multiplicative inverse of cos A. We define this as secant A. This is the ratio . In short, this is called sec A.
6. Take the multiplicative inverse of tan A. We define this as cotangent A. This is the ratio . In short, this is called cot A. This is the same as taking the ratio of cosec A and sec A.
These are the reciprocal functions.
Now you can place the torch at C and find the trigonometric functions of the angle C. This is in the right triangle ABC.
Examples where Trigonometric Functions are used to find the Side Lengths
Let us try finding the sides.
1. If cos A is , find the side BC here.
Solution: The trigonometric function is cosine here. Here AB= 10 and AC= 8. This is because cos A is the ratio of the adjacent side to angle A and the longest side AB called the hypotenuse. To find BC, we make use of the Pythagoras theorem. This theorem is used only for right triangles. It says that the square of the longest side is the same as the sum of the squares of the opposite side and the adjacent side.
The longest side here is 10. If you square it, it becomes 100. We have the adjacent side to angle A as 8. When you square this, it becomes 64. How will you find the square of the opposite side BC? Keep it as x2. So,
The length cannot be negative. The length of the side BC is 6.
2. If tan A is , find the other side.
Solution: The trigonometric function is tangent here. Since tan A is the ratio of the side opposite to angle A and the adjacent side, we have the opposite side as 3 and the adjacent side as 4. Since this is a right triangle we can use the Pythagoras theorem. The square of the hypotenuse is not known and it is taken as x2.
We can write x2=32+42. This will give us the value of x.
The side AB= 5. It is not -5 because the length cannot be negative.
3. If sin A is , find AC.
Hint: The trigonometric function is sine here.
Formulas
Consider that A is an acute angle of a right triangle.
The formulas for the three basic trigonometric functions are:
The formulas for the reciprocal functions are:
Common Mistakes
For sin A, do not think sin is multiplied with A. It means the sine of angle A. Similarly for all other terms. Notice the angle properly and write the trigonometric ratios. For each angle, the ratios are different.
Context and Applications
This topic is significant in the professional exams for both undergraduate and graduate courses, especially for
- Bachelor of Science in Mathematics
- Master of Science in Mathematics
Want more help with your trigonometry homework?
*Response times may vary by subject and question complexity. Median response time is 34 minutes for paid subscribers and may be longer for promotional offers.
Search. Solve. Succeed!
Study smarter access to millions of step-by step textbook solutions, our Q&A library, and AI powered Math Solver. Plus, you get 30 questions to ask an expert each month.
Search. Solve. Succeed!
Study smarter access to millions of step-by step textbook solutions, our Q&A library, and AI powered Math Solver. Plus, you get 30 questions to ask an expert each month.