
Database System Concepts
7th Edition
ISBN: 9780078022159
Author: Abraham Silberschatz Professor, Henry F. Korth, S. Sudarshan
Publisher: McGraw-Hill Education
expand_more
expand_more
format_list_bulleted
Concept explainers
Question
The off-line minimum problem maintains a dynamic set T of elements from the domain {1, 2,...,n}
under the operations INSERT and EXTRACT-MIN. A sequence S of n INSERT and m EXTRACT-
MIN calls are given, where each key in {1, 2,...,n} is inserted exactly once. Let a sequence S be
represented by I1 , E, I2, E, ... , E, Im+1 , where each Ij stands for a subsequence (possibly empty) of
INSERT and each E stands for a single EXTRACT-MIN. Let Kj be the set of keys initially obtained
from insertions in Ij. Thealgorithm to build an array extracted[1..m], where for i = 1, 2, ..., m,
extracted[i] is the key returned by the ith EXTRACT-MIN call is given below:
under the operations INSERT and EXTRACT-MIN. A sequence S of n INSERT and m EXTRACT-
MIN calls are given, where each key in {1, 2,...,n} is inserted exactly once. Let a sequence S be
represented by I1 , E, I2, E, ... , E, Im+1 , where each Ij stands for a subsequence (possibly empty) of
INSERT and each E stands for a single EXTRACT-MIN. Let Kj be the set of keys initially obtained
from insertions in Ij. The
extracted[i] is the key returned by the ith EXTRACT-MIN call is given below:
Off-Line-Minimum(m, n)
for i = 1 to n
determine j such that i ∈ Kj
if j ≠ m + 1
extracted[j] = i
let L be the smallest value greater than j for which KL exists
KL = KL U Kj, destoying Kj
return extracted
KL = KL U Kj, destoying Kj
return extracted
Given the operation sequence 9, 4, E, 6, 2, E, E, 5, 8, E, 1, 7, E, E, 3; where each
number stands for its insertion. Draw a table showing the building process of extracted[1..6].
number stands for its insertion. Draw a table showing the building process of extracted[1..6].
You have to show all Kj from Ij in the table and how they change (e.g. some have more elements due to merge
and some disappeared due to merge).
and some disappeared due to merge).
Use the attached table for the operation sequence 4, 8, E, 3, E, 9, 2, 6, E, E, E, 1, 7, E, 5 as a guide
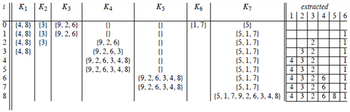
Transcribed Image Text:K₁ K₂ K3
0 (4,8) (3) (9,2, 6)
1 (4,8) (3) (9,2, 6)
2 (4,8) (3)
3
(4,8)
i
K4
0}
0
(9,2,6)
(9,2,6,3)
(9,2, 6, 3, 4, 8)
(9, 2, 6, 3, 4, 8)
Ks
0
0
(9,2, 6, 3, 4, 8)
(9,2, 6, 3, 4, 8)
K6
|{1,7}
K₁
(5)
(5, 1,7)
(5, 1,7)
(5, 1,7)
(5,1,7)
(5,1,7)
(5.1.7)
(5,1,7)
(5, 1, 7, 9, 2, 6, 3, 4, 8)
extracted
1|2|3|4|5|6
32
432
432
4326
4
32 6
43 26 8
TITL
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, computer-science and related others by exploring similar questions and additional content below.Similar questions
- A hash-map has been constructed with double-hashing by applying h;(k;) = [h(k;) + jd(k;)] mod N. The primary hashing function is given as h(k;) hashing function as d;(k;) = k;divN where div is integer division. The maximum number of cells probed is N = 13. How many cells will be probed by the call of findElement(38) = k;modN and the secondary Note: The first row contains the indices and the second row contains the elements. 1 2 4 5 6. 7 8 10 11 12 27 14 available 6. 35 25 51arrow_forwardAlgorithm for minimising a partition given a pair of pointsInput: a group G formed by S = s 1, s2,..... Sm operating on a set s = 1,2,..... n; two co I 0>2 of f2;Output: the best invariant partition of f such that co I and co 2 are in the same subset;arrow_forwardConsider a Skip List with n nodes. Each node k has a random "height" hg, which is the number of linked lists that includes node k. The heights nodes are statistically independent, where P[hx = m] = 2-m, for m 2 1 and P[h = m] = 0 , for m m] 0 such that P[H > m] <= for m = c log, narrow_forward
- The off-line minimum problem maintains a dynamic set T of elements from the domain {1, 2,...,n}under the operations INSERT and EXTRACT-MIN. A sequence S of n INSERT and m EXTRACT-MIN calls are given, where each key in {1, 2,...,n} is inserted exactly once. Let a sequence S berepresented by I1 , E, I2, E, ... , E, Im+1 , where each Ij stands for a subsequence (possibly empty) ofINSERT and each E stands for a single EXTRACT-MIN. Let Kj be the set of keys initially obtainedfrom insertions in Ij. The algorithm to build an array extracted[1..m], where for i = 1, 2, ..., m,extracted[i] is the key returned by the ith EXTRACT-MIN call is given below: Off-Line-Minimum(m, n)for i = 1 to n determine j such that i ∈ ?? if j ≠ m + 1 extracted[j] = i let L be the smallest value greater than j for which KL exists KL = KL U Kj, destroying ????return extracted (1) Given the operation sequence 9, 4, E, 6, 2, E, E, 5, 8, E, 1, 7, E, E, 3; where eachnumber stands for its…arrow_forwardInterchange Base Points AlgorithmOutput: a base B = [131,132..... 13j-1, 13L.+1, 13j, 13j+2, 13j+3..... 13k] for G; a strong generating set relative to B; procedure interchange(var B: sequence of points; vat S: set of elements; j: 1..k-1);Interchange Base Points AlgorithmInput: a group G; a base [131,132..... 13k] for G and a strong generating set; an integer j between 1 and k-l; Output: a base [131,132..... 13k] for G and a strong generating set.arrow_forwardProblem 2: In this problem we assume that h: U → {0, 1, . . . , m − 1} is a good hashfunction, that is, every key k has the same probability 1/m to map to any place in the tableT of length m.(i) What is the probability that three pairwise distinct elements u1, u2, u3 ∈ U aremapped by the function h to the same place in the table (that is, h(u1) = h(u2) =h(u3))?(ii) We insert three elements into an empty hash table T using the hash function h. Ifcollision is solved by chaining, what is the probability that T[0] and T[1] are empty?arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Database System ConceptsComputer ScienceISBN:9780078022159Author:Abraham Silberschatz Professor, Henry F. Korth, S. SudarshanPublisher:McGraw-Hill Education
Database System ConceptsComputer ScienceISBN:9780078022159Author:Abraham Silberschatz Professor, Henry F. Korth, S. SudarshanPublisher:McGraw-Hill Education Starting Out with Python (4th Edition)Computer ScienceISBN:9780134444321Author:Tony GaddisPublisher:PEARSON
Starting Out with Python (4th Edition)Computer ScienceISBN:9780134444321Author:Tony GaddisPublisher:PEARSON Digital Fundamentals (11th Edition)Computer ScienceISBN:9780132737968Author:Thomas L. FloydPublisher:PEARSON
Digital Fundamentals (11th Edition)Computer ScienceISBN:9780132737968Author:Thomas L. FloydPublisher:PEARSON C How to Program (8th Edition)Computer ScienceISBN:9780133976892Author:Paul J. Deitel, Harvey DeitelPublisher:PEARSON
C How to Program (8th Edition)Computer ScienceISBN:9780133976892Author:Paul J. Deitel, Harvey DeitelPublisher:PEARSON Database Systems: Design, Implementation, & Manag...Computer ScienceISBN:9781337627900Author:Carlos Coronel, Steven MorrisPublisher:Cengage Learning
Database Systems: Design, Implementation, & Manag...Computer ScienceISBN:9781337627900Author:Carlos Coronel, Steven MorrisPublisher:Cengage Learning Programmable Logic ControllersComputer ScienceISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education
Programmable Logic ControllersComputer ScienceISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education

Database System Concepts
Computer Science
ISBN:9780078022159
Author:Abraham Silberschatz Professor, Henry F. Korth, S. Sudarshan
Publisher:McGraw-Hill Education

Starting Out with Python (4th Edition)
Computer Science
ISBN:9780134444321
Author:Tony Gaddis
Publisher:PEARSON

Digital Fundamentals (11th Edition)
Computer Science
ISBN:9780132737968
Author:Thomas L. Floyd
Publisher:PEARSON

C How to Program (8th Edition)
Computer Science
ISBN:9780133976892
Author:Paul J. Deitel, Harvey Deitel
Publisher:PEARSON

Database Systems: Design, Implementation, & Manag...
Computer Science
ISBN:9781337627900
Author:Carlos Coronel, Steven Morris
Publisher:Cengage Learning

Programmable Logic Controllers
Computer Science
ISBN:9780073373843
Author:Frank D. Petruzella
Publisher:McGraw-Hill Education