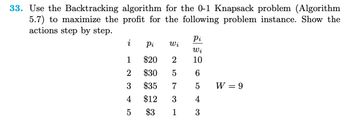
Use the Backtracking algorithm for the 0-1 Knapsack problem (Algorithm 5.7) to maximize the profit for the following problem instance. Show the actions step by step. Pi i Pi Wi Wi 1 $20 2 $30 25 10 6 3 $35 7 5 W = 9 4 $12 3 4 5 $3 1 3

X-Kingdom has trapped n number of soldiers of their opponent. They want to execute them. They created a strategy so that the prisoners will kill each other and at the end one prisoner will be alive and eventually released. As part of the process, they assigned each trapped soldier a sequence number starting from 1 and ending at n.    If n = 5 and k = 2, then the safe position is 3. Firstly, the person at position 2 is killed, then person at position 4 is killed, then person at position 1 is killed. Finally, the person at position 5 is killed. So, the person at position 3 survives. If n = 7 and k = 3, then the safe position is 4. The people at positions 3, 6, 2, 7, 5, 1 are killed in order, and the person at position 4 survives.   Input:n and k Output:Print the list of prisoners in reverse order from n to 1Then reverse the list to print it in correct order from 1 to nDisplay the position number who will survive. You must have to use circular doubly linked list for your solution. You…

In a hypothetical study of population dynamics, scientists have been tracking the number of rabbits and foxes on an island. The number of rabbits and foxes are determined once a year using high resolution infrared cameras and advanced computer vision methods.Each year, the number of rabbits and foxes are found to change by the following equations: $$ {\Delta}R = round( kr*R - krf*R*F ) $$ $$ {\Delta}F = round( -kf*F + kfr*R*F ) $$ where $ {\Delta}r $ and $ {\Delta} f $ are the changes in number of rabbits and foxes by the end of that year; and R and F are the population sizes at the end of the previous year. kr, krf, kf, kfr are coefficients that depend on the species of rabbits and foxes.With these dynamics, the scientists realize that one or both species can become extinct on the island. At the end of each year, if there are fewer than 2 animals of a kind, the scientists transfer rabbits and/or foxes to make sure there are at least 2 of each kind.Write a function…

An object leaves point P1 with coordinates (−1,0) and travels with velocity v1=1 in a straight line to point P2 with coordinates (0,h) . It then travels with velocity v2=2 in a straight line from P2 to point P3 with coordinates (x , y ). Given x and y values there will be some optimal h value that minimizes the total travel time. Write a program in Scilab containing a function in the form function u = h(x,y) [your code goes here] endfunction u = h(1,2); disp(u); that calculates this optimal h value (to an accuracy of about 10^−6 ) given x and y values as arguments. Your function must work for any positive values of x and y.