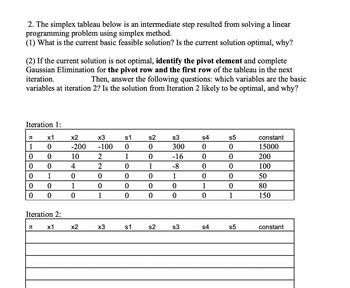
2. The simplex tableau below is an intermediate step resulted from solving a linear programming problem using simplex method. (1) What is the current basic feasible solution? Is the current solution optimal, why? (2) If the current solution is not optimal, identify the pivot element and complete Gaussian Elimination for the pivot row and the first row of the tableau in the next iteration. Then, answer the following questions: which variables are the basic variables at iteration 2? Is the solution from Iteration 2 likely to be optimal, and why? Iteration 1: Π x1 x2 x3 s1 s2 s3 s4 s5 constant 1 0 -200 -100 0 0 300 0 0 15000 0 0 10 2 1 0 -16 0 0 200 0 0 4 2 0 1 -8 0 0 100 0 1 0 0 0 0 1 0 0 50 0 0 1 0 0 0 0 1 0 80 0 0 0 1 0 0 0 0 1 150 Iteration 2: Π x1 x2 x3 s1 s2 s3 s4 $5 constant

1. (a)The objective is to shuffle a given array of n different elements. You would like to compare various shuffling algorithms and select the best of them. What does it mean: “the best shuffling algorithm”? What is a requirement for the perfect shuffling algorithm? (b) Is the Fisher-Yates Shuffle the perfect shuffling algorithm? Present a formal mathematical proof that this algorithm meets (or does not meet) the requirement given in (a). (c)  Evaluate empirically performance of the Fisher-Yates Shuffle for selected numerical values of n. Propose your evaluation criteria and explain your evaluation methodology. Report your observations and conclusions.

Solve the following exercise using jupyter notebook for Python, to find the objective function, variables, constraint matrix and print the graph with the optimal solution. Use linear programming methods to solve this problem. Represent the constraints and the objective function mathematically in code. Use Python and the SciPy and Matplotlib libraries to solve the problem and visualize the optimal solution on a graph. Clearly state the optimal solution and any assumptions made while solving the problem.   A farm specializes in the production of a special cattle feed, which is a mixture of corn and soybeans. The nutritional composition of these ingredients and their costs are as follows: - Corn contains 0.09 g of protein and 0.02 g of fiber per gram, with a cost of.$0.30 per gram.- Soybeans contain 0.60 g of protein and 0.06 g of fiber per gram, at a cost of $0.90 per gram.0.90 per gram. The dietary needs of the specialty food require a minimum of 30% protein and a maximum of 5% fiber.…

any idea how to solve ?

Exercise 2. Here is a classic geometric problem, in the same application domain as the Center Selection problem: Given n points with Cartesian coordinates (₁, 3) in the plane, and positive weights w, find one(!) point (x, y) that minimizes the weighted sum of the Euclidean distances to the given points. For formal clarity: We want to minimize n Σwi i-1 n •√(x- i-1 (x − x)² + (y - y₁)². This problem is at least not very easy to solve exactly. In the following we therefore propose a rough but rather quick and simple approximation algorithm: Instead of the Euclidean distance, take the Manhattan distance and minimize 1 w₁(x − x₂|+|y-yil). 2.1. Explain how the point that minimizes the weigthed sum of Manhattan distances can be found in polynonial time. That is: Sketch an algorithm, argue why it is correct, and explain your time bound. Try to keep the time bound as low as possible. 2.2. Show that this algorithm has an approximation ratio √2. More specifi- cally: The point found by the…

Linear Programming: Graphical Method Formulate the LP problem, which includes the objective function and the constraints. Then solve. Use Desmos calculator to graph. A furniture company produces inexpensive tables and chairs. The production process for each is similar in that both require a certain number of hours of carpentry work and a certain number of labor hours in the painting department. Each table takes 4 hours of carpentry and 2 hours in the painting department. Each chair requires 3 hours of carpentry and 1 hour in the painting department. During the current production period, 240 hours of carpentry time are available and 100 hours in painting is available. Each table sold yields a profit of P600; each chair produced is sold for a $250 profit. Find the best combination of tables and chairs to manufacture in order to reach the maximum profit.

Course: Linear Programming

MUST BE DONE IN PHYTON

Knapsack 0/1 problem: Given N items where each item has some weight and profit associated with it and also given a bag with capacity W, [i.e., the bag can hold at most W weight in it]. The task is to put the items into the bag such that the sum of profits associated with them is the maximum possible. Given the problem is solved using a dynamic programming approach and the matrix derived is given below, answer the below set of questions by analyzing the DP matrix. weights = [2, 3, 4, 5], profits = [1, 2, 5, 6], Capacity W = 8 Capacity 2 3 Profits weights|0 1 2 5 16 14 |-> 5 10 0 O 10 1 2 3 4 0 O 0 1 1 0 1 0 1 O 10 1 2 2 2 5 2 O 15 50 1 356 6 O 1 3 6 18 00378 7 10 10 1 3 7 7 1 18

Machine Learning Problem Perform the optimization problem of finding the minimum of J(x) = (2x-3)2 by: (i) defining theta, J(theta), h(theta) as defined in the Stanford Machine Learning videos in Coursera; (ii) plotting J(theta) vs theta by hand then use a program (iii) determining its minimum using gradient descent approach starting from a random initial value of theta = 5. Perform the search for the minimum using the gradient descent approach by hand calculations, i.e., step 1, step 2, etc. showing your work completely