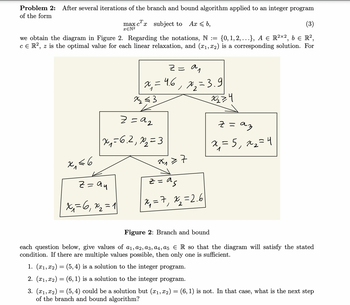
After several iterations of the branch and bound algorithm applied to an integer program max cx subject to Ax≤ b, (3) EN² we obtain the diagram in Figure 2. Regarding the notations, N = {0, 1, 2,...}, A E R²x2, 6 € R², CE R2, z is the optimal value for each linear relaxation, and (x1, x2) is a corresponding solution. For Problem 2: of the form x₁≤6 z = 9₁ x₁ = 4.6 x ₁₂ = 3.9 2 Z=94 x₁ = 6₁20/₂=1 x2≤3 2 7=92 x₁ = 6.2,%₂2=3 x₁ = 7 z=as x₁ = 7, x₂ = 2.6 20274 z = 93 x ₂₁ = 5₁ x ₂ = 4 Figure 2: Branch and bound each question below, give values of a1, a2, a3, a4, a5 ER so that the diagram will satisfy the stated condition. If there are multiple values possible, then only one is sufficient. 1. (x1, x2)=(5, 4) is a solution to the integer program.

Question 7 Classify the nature of this linear program. Assume all the variables are non-negative. Min-2X + Y subject to 3X-Y 22 -X+ 2Y ≤ 10 Y ≤5

CLO3: The following graph represents the growth functions of two different algorithms. Use the graph to answer the questions follow: Function's Growth 140 120 100 80 60 20 1 2 3 4 5 6 7 9 10 11 8 FF-5*x+14 for agor thm B Gx)= x*2 for algorithm A 1) Which algorithm, A or B, performs better when (problem size) n is less than 7? (a 2) Which algorithm, Aor B, performs better when (problem size) n is greater than 7? 3) What can you conclude about the performance of the two algorithms when (problem size) n= 7?

1. Solve the following linear programmne using the 2-phase simplex algorithm. You should give the initial tableau and each further tableau produced during the exe- cution of the algorithm. If the program has an optimal solution, give this solution and state its objective value. If it does not have an optimal solution, say why. 2x2x34x4 no need maximize x1- subject to 2x1+x22x3 — x4 ≥ 1, 5x1 x2 x3x4 -1, 2x1 x2 x3 3x4 2, X1, X2, X3, X4 ≥ 0. 2. Apply the first phase of the 2-phase simplex algorithm to the following linear pro- gramme giving the initial tableau and each further tableau produced. Give the starting tableau for the second phase if there is one. maximize 2x1 + x2 + 3x3 x2-x32, subject to x13x2+2x3 ≥ 3, 2x12x2 x3 = 4, X1, X2, X3 0.