
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
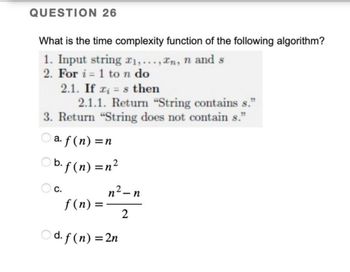
Transcribed Image Text:QUESTION 26
What is the time complexity function of the following algorithm?
1. Input string ₁,..., n, n and s
2. For i=1 to n do
2.1. If zis then
2.1.1. Return "String contains s."
3. Return "String does not contain s."
a. f(n)=n
b₁f(n) = n²
C.
f(n)=
n²_n
2
d. f(n) = 2n
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps

Knowledge Booster
Similar questions
- 31.arrow_forwardWhat is the largest n for which one can solve within 10 seconds a problem using an algorithm that requires f(n) bit operations, where each bit operation is carried out in 10-10 seconds, with these functions of n? a. log₂ n b. √n c. n7 d. 10n³arrow_forward1 Consider the roots (zeros) of f(x) =a³ – 4x + 1. 7+ 6- 5- 4- -4 -3 -2 3 -1- -2- -3 -4 -5+ We will see that small changes in the choice of x, produce different roots, or none at all. For each x, given, state the root (zero) of f(x) to which the algorithm converges, or write DNE if it does not converge. round to 3 decimal places If x, = 1.85, then Newton's Method converges to: x = If r. 1.7, then Newton's Method converges to: * = If To 1.55, then Newton's Method converges to: 2 = 3.arrow_forward
- 1. Draw the flowchart and trace table for the exponential function shown below. The Algorithm should request from the user the value of "x" and the number of iterations (approximation-stopping point). Both should be positive integers. For the factorial, you can use the built-in function, or in other words, you can represent the factorial (n!) as a Predefined Process 1=1+x+x²+x²³...arrow_forwardSolve the following Integer Programming problem using the graphical or branch and bound algorithm, where n=5, s=13.arrow_forward5. Find the generating function to determine the number of ways to pick k objects from n objects when repetitions are allowed and the ith object appears i or 2 times for 1≤ i ≤n.arrow_forward
- 6. Discover the recursive formula for Hn. Find Hn for n=1,2,3...6. Based on these values you will be able tosee the recursive pattern. proving the formula is optional for extra credit. Consider all subsets of N={1, 2, 3,..., n). How many of these subsets, SC N, satisfy the property that (i) Vx (x ES → x +1 ¢ S) ? Denote the number of subsets satisfying this property (i) by Hn. (a) Find the first 6 values of Hn . I have calculated n=1 and n=2, so you need to do n=3, 4, 5, 6 (b) Find and prove the recursive formula for the sequence Hn . Example: Let n=1. Then N={1} and the subsets satisfying property (i) are {1} and O so H1=2. Let n=2. Then N={1,2} and the subsets satisfying property (i) are {1}, {2}, and Ø, i.e. H3=3. Note: {1,2} is also subset of {1,2} but since for x=1, x+1=2 and both 1 and 2 are contained in {1,2} therefore the set {1,2} fails to satisfy property (i). 7. Prove by induction that the number of subsets for a set with n elements is 2^n.arrow_forwardPlease include justifications for each step.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

