
Concept explainers
(a)
The
(a)
Answer to Problem 11.29P
The
Explanation of Solution
Write the expression to find the Hamiltonian matric for a spinning charge particle in a magnetic field
Here,
Write the expression for appropriate spin matrix
Where,
Given, the total magnetic field
Where,
Solving equation (I),
Substitute equation (II) in the above equation,
Solving further,
Conclusion:
Thus, the
(b)
Show that
(b)
Answer to Problem 11.29P
It has been proved that
Explanation of Solution
Write the time-dependent Schrodinger equation
Given,
Thus,
Substitute the above relations in equation (IV)
From part (a),
Thus,
Hence proved.
Conclusion:
It has been proved that
(c)
Whether the general solution for
(c)
Answer to Problem 11.29P
It has been proved that the general solution for
Explanation of Solution
From part (b),
Using the above relations,
Differentiate
Rewrite the above equation in the form of second order differential equation,
Solving the terms inside the bracket,
Substitute the above relation in equation (V)
The characteristic equation is
Solving the above quadratic equation,
Where,
The general solution
The general solution
For the initial condition,
From part (b),
Substitute
Substitute equation (IX) and (X) in (VII)
And
Hence proved.
Conclusion:
It has been proved that the general solution for
(d)
The probability of a transition to spin down, as a function of time.
(d)
Answer to Problem 11.29P
The probability of a transition to spin down, as a function of time is
Explanation of Solution
Write the expression to find the probability of transition to spin down
Given,
Substitute
Now, the probability of a transition to spin down is
Conclusion:
Thus, the probability of a transition to spin down, as a function of time is
(e)
Sketch the resonance curve,
(e)
Answer to Problem 11.29P
The resonance curve,
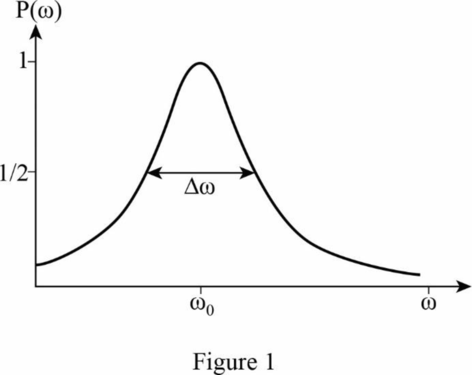
the full width at half maximum is
Explanation of Solution
Given,
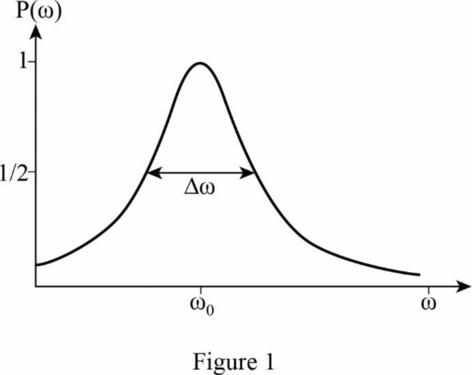
Taking square root on both sides,
Thus, the full width at half maximum,
Conclusion:
The resonance curve,
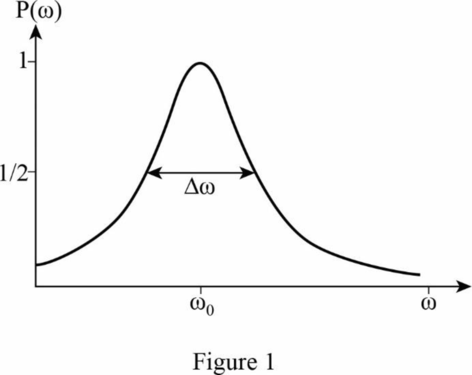
the full width at half maximum is
(f)
The resonant frequency in a nuclear magnetic resonance experiment and the width of the resonance curve.
(f)
Answer to Problem 11.29P
The resonant frequency in a nuclear magnetic resonance experiment is
Explanation of Solution
Compare Equation 4.156 and 7.89, the gyro-magnetic ratio for a proton is
Where,
Write the expression to find the resonant frequency
Where,
Substitute equation (XI) in the above equation,
Substitute
Write the expression to find the width of the resonance curve
Where,
Thus, the above equation becomes,
Substitute
Conclusion:
Thus, the resonant frequency in a nuclear magnetic resonance experiment is
Want to see more full solutions like this?
Chapter 11 Solutions
INTRO TO QUANTUM MECHANICS
- What is the maximum kinetic energy of an electron such that a collision between the electron and a stationary hydrogen atom in its ground state is definitely elastic?arrow_forwardWhat is the magnitude of the spin momentum of an electron? (Express you answer in terms of h.)arrow_forwardFor a 3d electron in an external magnetic field of 2.50 × 10-3 T, find (a) the current associated with the orbital angular momentum, and (b) the maximum torque.arrow_forward
- What is β for a particle with (a) K = 5.29 E0 and (b) E = 5.29 E0?arrow_forwardThe total potential energy of a system is determined by the sum of all pairwise interactions. (LJ potential) For a given number of particles, what is the total number of pairwise potential energy calculations required to define the total potential energy of the system?arrow_forwardIn this question, please explain to me why x and y components are not considered? Why is it simply B1cos60 + B1cos60?arrow_forward
- An electron undergoes a spontaneous transition emitting electromagnetic radiation with a frequency centered on a wavelength λ = 532 nm. Ideally, from a classical point of view, what is the characteristic decay time of the electron and what is the width of the spectral line?arrow_forwardsolve mcq number 8 and give answer from any four of the followingarrow_forwardWould the radial wave functions be different for a potential V(r) other than the Coulomb potential? Explainarrow_forward
- Suppose that a hydrogen atom in its ground state moves 80 cm through and perpendicular to a vertical magnetic field that has a magnetic field gradient dB/dz = 1.6 * 102 T/m. (a) What is the magnitude of force exerted by the field gradient on the atom due to the magnetic moment of the atom’s electron, which we take to be 1 Bohr magneton? (b) What is the vertical displacement of the atom in the 80 cm of travel if its speed is 1.2 * 105 m/s?arrow_forwardAt time t = 0 the wave function of the hydrogen atom is: where we ignore the spin.(a) Calculate the expected value of energy for this system.(b) What is the probability of finding the system at l = 1, m = +1 as a function of time?(c) What is the probability of finding the electron around 10−10 cm from the proton, at t = 0s (canapproximate).(d) Write the time-dependent wave function: ψ (r,t)arrow_forward
 Modern PhysicsPhysicsISBN:9781111794378Author:Raymond A. Serway, Clement J. Moses, Curt A. MoyerPublisher:Cengage Learning
Modern PhysicsPhysicsISBN:9781111794378Author:Raymond A. Serway, Clement J. Moses, Curt A. MoyerPublisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning University Physics Volume 3PhysicsISBN:9781938168185Author:William Moebs, Jeff SannyPublisher:OpenStax
University Physics Volume 3PhysicsISBN:9781938168185Author:William Moebs, Jeff SannyPublisher:OpenStax Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning
Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning




